Question
Section 7.4 Reading Assignment: Relative Rates of Growth Answer Only Exercise 1, 2, and 3 by using a screenshot provided Calculus Pearson textbook. Make sure

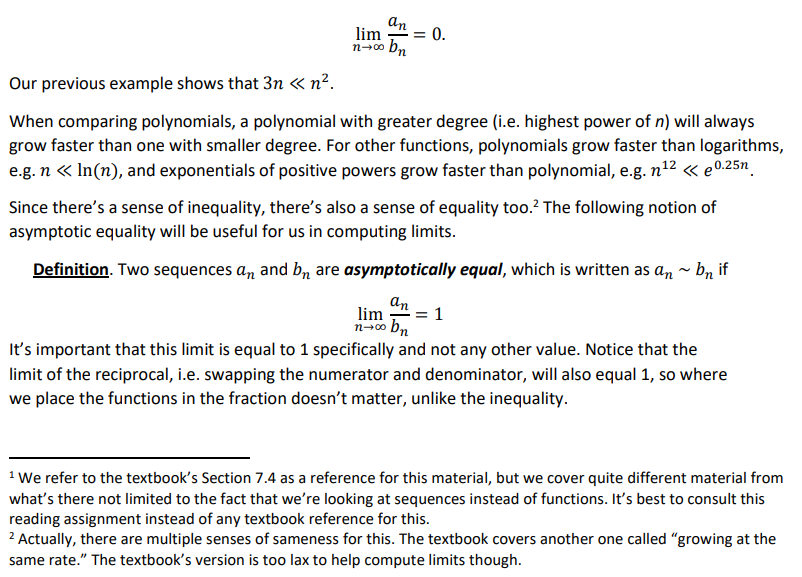
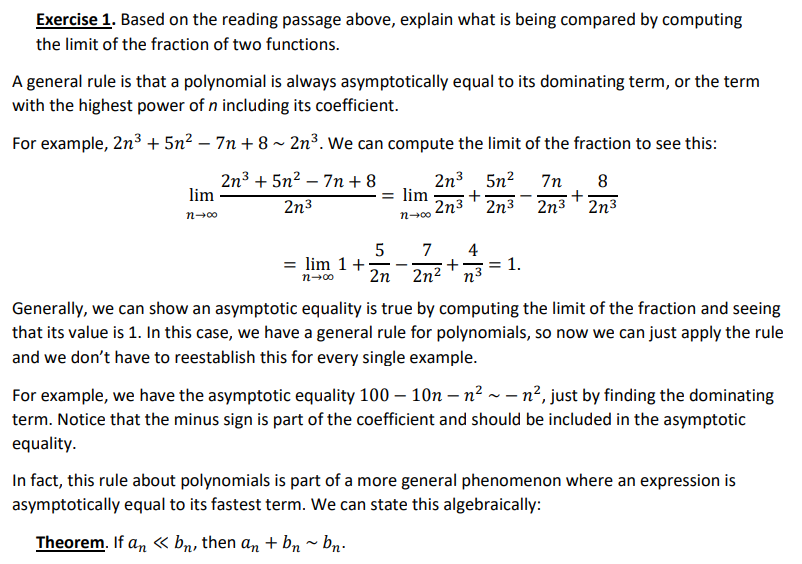



Section 7.4 Reading Assignment: Relative Rates of Growth
Answer Only Exercise 1, 2, and 3 by using a screenshot provided Calculus Pearson textbook. Make sure you read these three questions very carefully and see on what it is asking for and what is really about. Please be careful with this assignment.
References: Thomas' Calculus: Early Transcendentals | Calculus | Calculus | Mathematics | Store | Pearson+
Feedback: #1: What exactly is being compared when we consider the limit of this fraction based on the reading? (0/2) #2: Reconsider this one based on the reading, especially the numerical result. (1/2) #3: Rephrase this one and reconsider the process considered in this reading. (1/2).

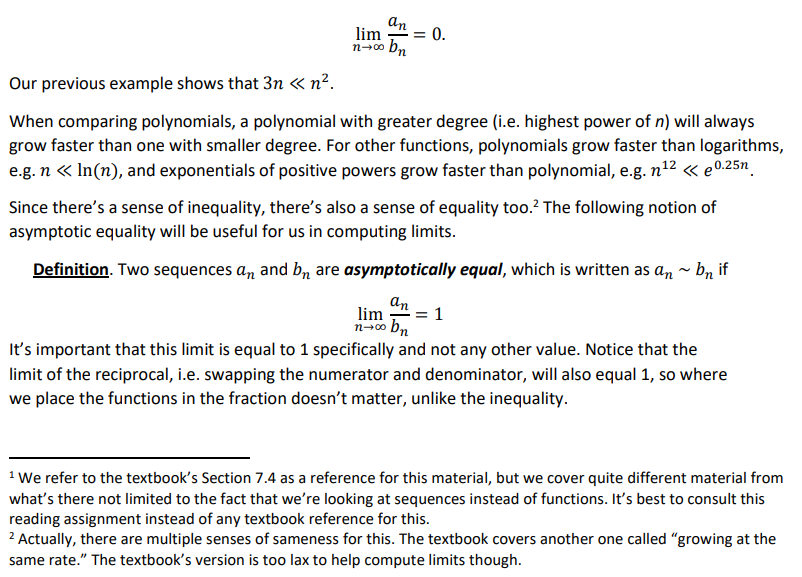


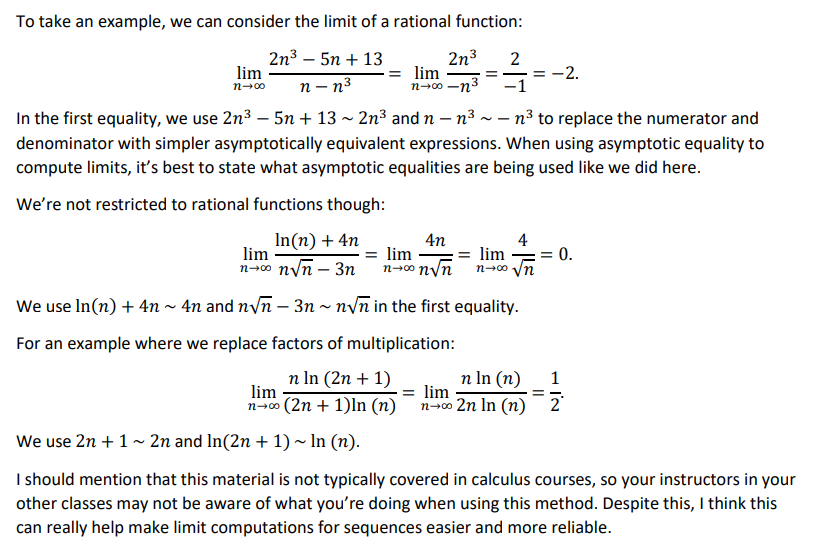

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


