Show all the workings

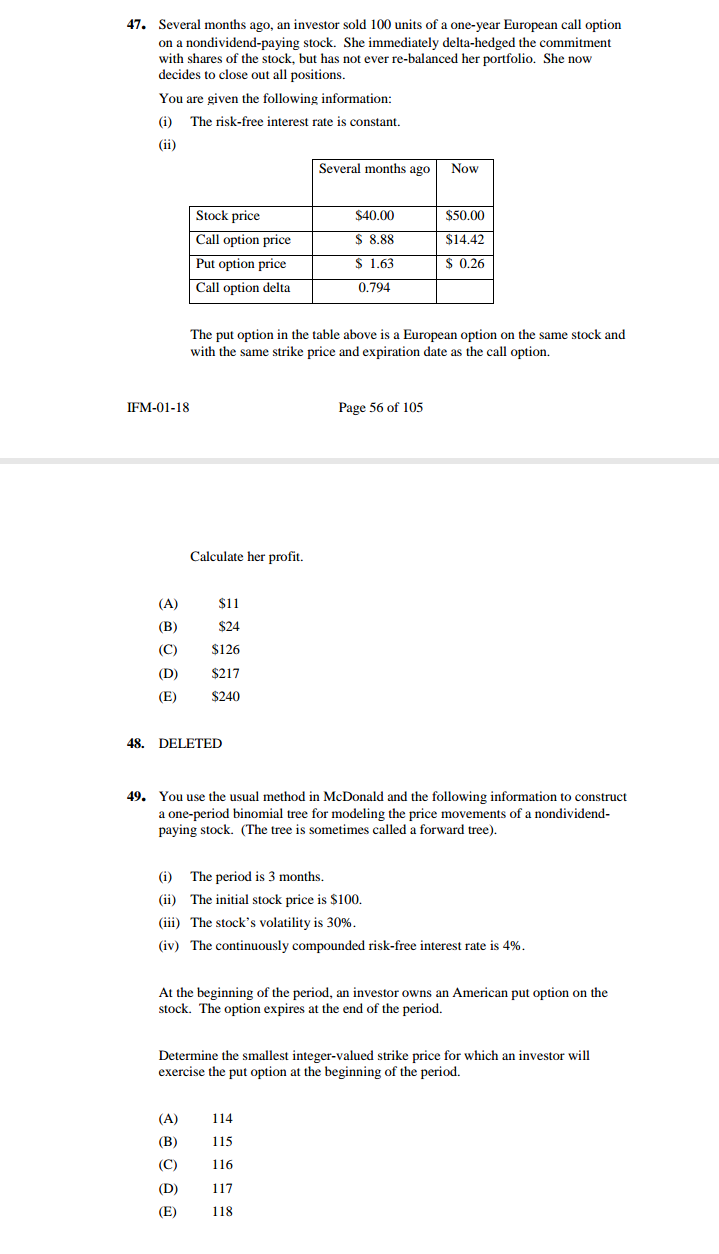
44. Consider the following three-period binomial tree model for a stock that pays dividends continuously at a rate proportional to its price. The length of each period is 1 year, the continuously compounded risk-free interest rate is 10%, and the continuous dividend yield on the stock is 6.5%. 585.9375 468.75 375 328.125 300 262.5 210 183.75 147 102.9 Calculate the price of a 3-year at-the-money American put option on the stock. (A) 15.86 (B) 27.40 (C) 32.60 (D) 39.73 (E) 57.49 45. DELETED IFM-01-18 Page 55 of 105 46. You are to price options on a futures contract. The movements of the futures price are modeled by a binomial tree. You are given: (i) Each period is 6 months. (ii) uld = 4/3, where u is one plus the rate of gain on the futures price if it goes up. and d is one plus the rate of loss if it goes down. (iii) The risk-neutral probability of an up move is 1/3. (iv) The initial futures price is 80. (v) The continuously compounded risk-free interest rate is 5%. Let Cr be the price of a 1-year 85-strike European call option on the futures contract, and Cyr be the price of an otherwise identical American call option. Determine Cu - Cr. (A) 0 (B) 0.022 (C) 0.044 (D) 0.066 (E) 0.08847. Several months ago, an investor sold 100 units of a one-year European call option on a nondividend-paying stock. She immediately delta-hedged the commitment with shares of the stock, but has not ever re-balanced her portfolio. She now decides to close out all positions. You are given the following information: (i) The risk-free interest rate is constant. (ii) Several months ago Now Stock price $40.00 $50.00 Call option price $ 8.88 $14.42 Put option price $ 1.63 $ 0.26 Call option delta 0.794 The put option in the table above is a European option on the same stock and with the same strike price and expiration date as the call option. IFM-01-18 Page 56 of 105 Calculate her profit. (A) $11 (B) $24 (C) $126 (D) $217 (E) $240 48. DELETED 49. You use the usual method in Mcdonald and the following information to construct a one-period binomial tree for modeling the price movements of a nondividend- paying stock. (The tree is sometimes called a forward tree). (i) The period is 3 months. (ii) The initial stock price is $100. (iii) The stock's volatility is 30%. (iv) The continuously compounded risk-free interest rate is 4%. At the beginning of the period, an investor owns an American put option on the stock. The option expires at the end of the period. Determine the smallest integer-valued strike price for which an investor will exercise the put option at the beginning of the period. (A) 114 (B) 115 (C) 116 (D) 117 (E) 11850. Assume the Black-Scholes framework. You are given the following information for a stock that pays dividends continuously at a rate proportional to its price. (i) The current stock price is 0.25. (ii) The stock's volatility is 0.35. (iii) The continuously compounded expected rate of stock-price appreciation is 15%. Calculate the upper limit of the 90% lognormal confidence interval for the price of the stock in 6 months. (A) 0.393 (B) 0.425 (C) 0.451 (D) 0.486 (E) 0.529 51-53. DELETED 54. Assume the Black-Scholes framework. Consider two nondividend-paying stocks whose time-f prices are denoted by Si(t) and Sz(t), respectively. You are given: (i) SI(0) = 10 and $2(0) = 20. (ii) Stock I's volatility is 0.18. (iii) Stock 2's volatility is 0.25. The correlation between the continuously compounded returns of the two stocks is -0.40. (v) The continuously compounded risk-free interest rate is 5%. (vi) A one-year European option with payoff max { min[251(1), $2(1)] - 17, 0) has a current (time-0) price of 1.632. Consider a European option that gives its holder the right to sell either two shares of Stock 1 or one share of Stock 2 at a price of 17 one year from now. Calculate the current (time-0) price of this option. IFM-01-18 Page 58 of 105 (A) 0.67 (B) 1.12 (C) 1.49 (D) 5.18 (E) 7.86 55. Assume the Black-Scholes framework. Consider a 9-month at-the-money European put option on a futures contract. You are given: (i) The continuously compounded risk-free interest rate is 10%. (ii) The strike price of the option is 20. (iii) The price of the put option is 1.625. If three months later the futures price is 17.7, what is the price of the put option at that time? (A) 2.09 (B) 2.25 (C) 2.45 (D) 2.66l. Cmpetition and Hospital market (20%} The demand of hospital care at Town A. is P = 15- X, where p is the price of a hospital service, and .t' is the quantity of services demanded by patients. Suppose there is only one hospital with the cost function C = 2D +531. {a} Assuming that this hospital cares only the profit, compute the optimal price and quantity for hospital B. [5'36] (h) 1What is the HHI index based on output? {5%} Suppose that this hospital is a nonprot hospital which has an incentive to provide as much health care as possible. {c} 1Write down the zero-prot condition for this hospital (5'36) {I-Iint: TR=TC) [d] Compute the price and quantity that this hospital would charge under zero-prot condition (5'36) 2. Physitan-indnced Demand {21} points} Consider the following physician utility: =mr-.5i2 where at: he the dollars per unit of service, i: be the total unit of provided service, and i indicates the inducement that the physician takes to inuence the patient's demand. It is believed that the relationship between it and i can be written as: x: -lmtili {a} Suppose the fee per unit of service is ID. Please calculate the optimal inducement [i] and demanded service [it] for the physician. (5'36) (h) Now the govemment announced a fee reduction from it} to 5, how does the fee reduction affect the inducement? What is the optimal demanded service {a}? {5%} {c} According to {h}, what portion of the change of x is due to the change of inducement? (5%] [d] According to {c}, explain why it is empirically difficult to use the changes of demanded service after fee reduction as the evidence of P111 Please explain careilly {5%} {e} Empirically testing the physician induced demand follows closely the mode] of McGuire and Pauly {1991}, where they employ two markets {services} to identify PID. Can you explain the intuition why two markets {services} would help identifying PIIJ? (5%} 3. Brand-name and Genetic Drugs {25%) A pharmaceutical company E considers in developing the new drug K. The annual demand of the new drug K is x = Ell-2p, where p is the price of a prescription drug, and r is the quantity demanded by patients. For simplicity, assume that company B can produce the new drug an extra pill at a constant cost, and hence the marginal cost function is MC24. {a} How much Comp B should charge for the price of Drug K? What is the annual prot'}.I Please explain carefully [5'36] Suppose the demand for Drug K comes from two towns: Al and A2, where xl-p and 12:1{l-p is the demand for each town separately [h] Suppose that lComp E can charge different prices for each town. How much should lCon-11p B charge for the new drug at A1 and A2 respectively? Please explain the answer carefully (5%] {c} Comparing (h) with {a}, which one has a higher price at Town All Please use this result to explain why some branded name drugs charge an even higher price after the expiration of patent. (5'36) The government grants the new drug's patent for three years. After three years, it is assumed that a generic drug would enter the market that could drive the market price near the marginal cost. [:1] Assume patients in Al have no interests in generic drug, and those in A2 treat the new and the generic drug as indifferent [two drugs share the market equally}. What is the annual prot of Comp B from Al and A2 respectively after patent'}.I Please explain carefully {5%) {e} The president of Comp B has decided to develop Drug K if the company could recoup all the as: D cost [approximately 35D) within five years. Based on these numbers. please help him decide whether he should invest the new drug {5% interest rate when calculating the present value} {5%} (Hint: the new drug will be granted for patent in the rst three years} 4. CEA and CBA (25%) Paul fell off his bike and damaged his neck. Without treatment his quality of life (QOL) per year is expected to be 0.75 for the rest of his life (five years). Paul has the following two treatment choices: Neck surgery: Costs $4,000 immediately and increases Paul's QoL to 1.0 for the remaining life (four years) Physiotherapy: Costs $1000 per year (at the beginning of each year) and increases Paul's Qol to 0.8 for five years. Assume a discount rate (r) of 5% per annum (a) Calculate the QALYs for each treatment (5%) (b) Conduct the CER (cost and effective ratio) and help Paul decide which treatment to take (5%) Paul is more inclined to have the surgery, but he hopes to conduct the cost and benefit analysis to confirm this choice. (c) It is reported that the chance of saving one person from the airbag is one out of 10000 and the cost of one airbag is 500. From these two numbers, please calculate the value of statistical life. (5%) (d) Please write down the marginal benefit and cost of doing the surgery in the last five years. (5%) (Hint: compare with no treatment) (e) Suppose that the value of one year of life (undiscounted) is 50000. Use the cost and benefit analysis to help Paul decide whether he should have the surgery (5%) 5. Single Choice Questions (10%) I. Which following country has the highest health expenditure (in terms of GDP) (a) Taiwan (b) Japan (c) France (d) United States II. According to J. DiMasi, R. Hansen, and H. Grabowski, "The Price of Innovation: New Estimates of Drug Development Costs", Jan 2002, how much does it cost to produce a new drug? (a) 100 millions (b) 400 millions (c) 8 millions (d) 12 millions III. Which number is a more reasonable estimate of statistical life in US? (a) 1 million (b) 5 millions (c) 10 millions (d) 20 millions IV. On average, how long does it take for a new drug to complete the clinical testing? (Phase 1-III) (a) 3 years (b) 7 years (c) 10 years (d) 15 years V. What was the life expectancy in Taiwan around 1920? (a) 40 years (b) 50 years (c) 60 years (d) 70 years