Question: Show that the map d: R x R R defined by d(x, y) = ||x-y||/1+||x-y|| ,is a metric, where || || denotes the Euclidean

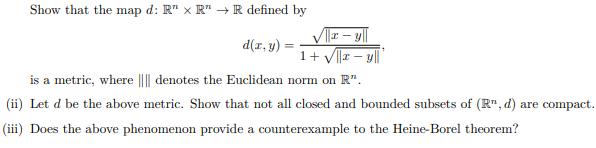
Show that the map d: R" x R" R defined by d(x,

Show that the map d: R" x R" R defined by d(x, y) = ||x-y||/1+||x-y|| ,is a metric, where || || denotes the Euclidean norm on R". (ii) Let d be the above metric. Show that not all closed and bounded subsets of (R", d) are compact. (iii) Does the above phenomenon provide a counterexample to the Heine-Borel theorem? r-yll 1+ ||z-y||

Step by Step Solution

★★★★★

3.48 Rating (158 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The detailed answ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Show that the map t: P2 P2 given by t(ax2 + bx + c) = bx2 - (a + c)x + a is an isomorphism.

Show that the map f: R1 R1 given by f(x) = x3 is one-to-one and onto. Is it an isomorphism?

The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...

Stream functions for steady three-dimensional flow. (a) Show that the velocity functions pv = [ x A] and pv = [( 1 ) x ( 2 )] both satisfy the equation of continuity identically for steady...

A painting process consists of two stages. In the first stage, the paint is applied, and in the second stage, a protective coat is added. Let X be the time spent on the first stage, and let Y be the...

Let G be a group and let g be one fixed element of G. Show that the map i g , such that i g (x) = gxg' for x G, is an isomorphism of G with itself.

The group defined by generators a, b and relations a n = e (3 n N*), b 2 = e and abab = e is the dihedral group D n . [See Theorem 6.13.] Theorem 6.13 For each n 3 the dihedral group D n is a...

Use Theorems 14 to show that the function is continuous. (x) = x + sin x THEOREM 1 Basic Laws of Continuity If f and g are continuous at x = c, then the following functions are also continuous at x =...

The following exercise furnishes an example of a martingale $\left(M_{n}ight)_{n \in \mathbb{N}}$ on the probability space \(\left([0,1], \mathscr{B}[0,1],...

12.9 The trade theorems The construction of the production possibility curve shown in Figures 12.2 and 12.3 can be used to illustrate three important theorems in international trade theory. To get...

If the exchange rate value of the British pound goes from U.S. $1.80 to U.S. $1.60, then a. The pound has appreciated, and the British will find U.S. goods cheaper. b. The pound has appreciated, and...

Ed Lubbock's FlashCard bill is below. There are entries missing. a. What is Ed's average daily balance? b. What is Ed's finance charge? c. What is Ed's new balance? d. What is Ed's available credit?...

x = 7 7 t , y = t - 8 by eliminating the parameter.

The Shady Tree Company is preparing to announce their quarterly earnings numbers. The company expects to beat the analysts' forecast of earnings by at least 5 cents a share. In anticipation of the...

Marty and Jean are married and have 4-year-old twins. Jean is going to school full-time for 9 months of the year, and Marty earns $45,000. The twins are in day care so Jean can go to school while...

The table below shows annual returns on Archer Daniels Midland (ADM) and Walmart. The last column of the table shows the annual return that a portfolio invested 50% in ADM and 50% in Walmart would...

Clarita is a single taxpayer with two dependent children, ages 10 and 12. Clarita pays $3,000 in qualified child care expenses during the year. If her adjusted gross income (all from wages) for the...

Refer to the data regarding Juda Products in Exercise E26-24. Compute the IRR of each project, and use this information to identify the better investment. In Exercise 26.24 Use the NPV method to...

Which of the following statements about the Family and Medicall Leave Act is accurate?Employers having 10 or more employees during 20 or more calendar woekweeks in the current or pooceding year ase...

Q4- Write the program in the next page, complete it with the header files. Save it as "lab 12q4.c" a. Include the source file in your lab report and show the commands used in the lab report and their...

in c programming please Task Three the robotwill ask the user about his/her destination where he/she can choose from the following three destinations: America, Europe, and Africa, as shown in Fig.3.1...

(a) Prove that for any linear map h: V W and any W, the set h-1() has the form { + | N (h)} for V with h() = (if h is not onto then this set may be empty). Such a set is a coset of N (h) and we...

1 (Graded). (i) Determine the exact solution to the IVP y' = siWy 11(1) = 2 (ii) Implement the 4stage RungeKutta method given by the Butcher tableau Test it on the IVP from (i J on the interval I [1...

15. Consider the mapping from M,(Z) into Z given by a b d -> a . Prove or disprove that this is a ring homomorphism. 16. Let R = 1. ab, cEZ . Prove or disprove that the map- ping a b C . - a is a...