





Show the workings in each.
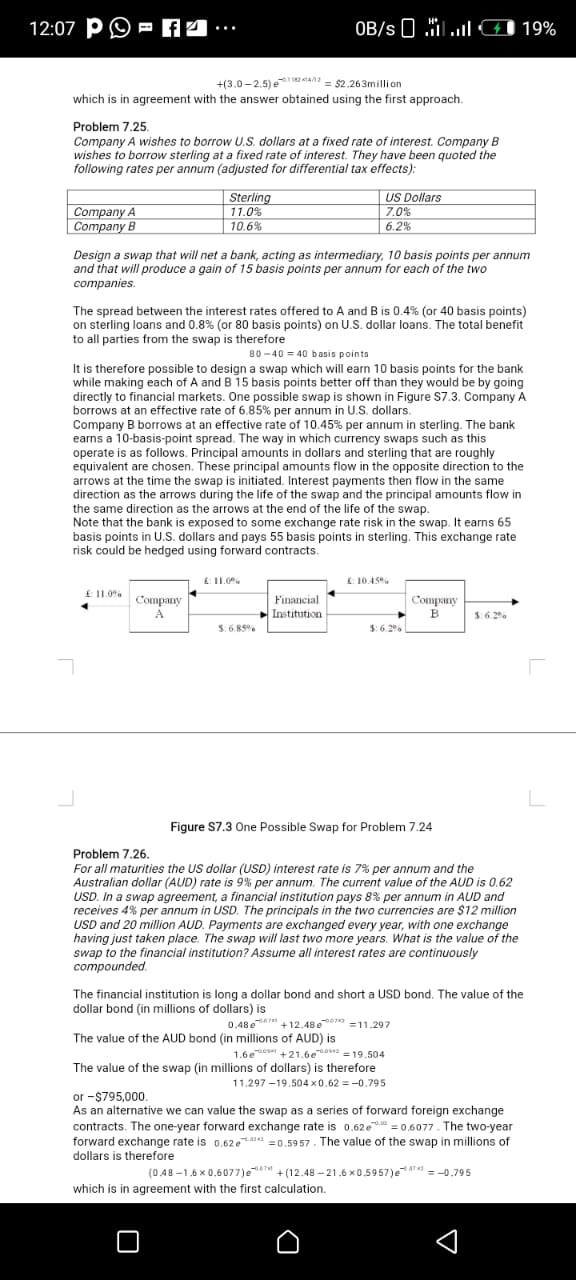
20. Compare the adjustment to medium run equilibrium after a fiscal contraction of a fixed and flexible exchange rate economy. What happens in the short run and during the adjustment to the medium run? Compare your results with the closed economy case.(b) Suppose the policy is implemented through a payroll tax. What is the new equilibrium wage, total compensation for a worker, cost of employing a worker, and labor supplied? What is the tax revenue and DWL? Who bears more of the burden of the tax?Question 4 (20 points) Suppose Montana establishes an income tax schedule that has a tax rate of 5% on the first $20,000 of income, 10% on the next $10,000, and then 20% on all taxable income above $30,000. Montana provides a $2,000 exemption (deduction) per family member. (Let's call this tax system A.) 1. The Abrams family has three members. Thomas is the sole earner, and he has two kids. The family's annual income is $35,000. Calculate their:12:07 p - f 4 ... OB/s O Mil .ill 1 19% +(3.0-2.5)= $2.263million which is in agreement with the answer obtained using the first approach. Problem 7.25. Company A wishes to borrow U.S. dollars at a fixed rate of interest. Company B wishes to borrow sterling at a fixed rate of interest. They have been quoted the following rates per annum (adjusted for differential tax effects): Sterling US Dollars Company A 11.0% 7.0% Company B 10.6% 6.2% Design a swap that will net a bank, acting as intermediary, 10 basis points per annum and that will produce a gain of 15 basis points per annum for each of the two companies. The spread between the interest rates offered to A and B is 0.4% (or 40 basis points) on sterling loans and 0.8% (or 80 basis points) on U.S. dollar loans. The total benefit to all parties from the swap is therefore 80 -40 = 40 basis points It is therefore possible to design a swap which will earn 10 basis points for the bank while making each of A and B 15 basis points better off than they would be by going directly to financial markets. One possible swap is shown in Figure S7.3. Company A borrows at an effective rate of 6.85% per annum in U.S. dollars. Company B borrows at an effective rate of 10.45% per annum in sterling. The bank earns a 10-basis-point spread. The way in which currency swaps such as this operate is as follows. Principal amounts in dollars and sterling that are roughly equivalent are chosen. These principal amounts flow in the opposite direction to the arrows at the time the swap is initiated. Interest payments then flow in the same direction as the arrows during the life of the swap and the principal amounts flow in the same direction as the arrows at the end of the life of the swap. Note that the bank is exposed to some exchange rate risk in the swap. It earns 65 basis points in U.S. dollars and pays 55 basis points in sterling. This exchange rate risk could be hedged using forward contracts. (: 10.150% 6: 11.0% Company Financial Company A Institution B 5:6.85% $:6.20% L Figure S7.3 One Possible Swap for Problem 7.24 Problem 7.26. For all maturities the US dollar (USD) interest rate is 7% per annum and the Australian dollar (AUD) rate is 9% per annum. The current value of the AUD is 0.62 USD. In a swap agreement, a financial institution pays 8% per annum in AUD and receives 4% per annum in USD. The principals in the two currencies are $12 million USD and 20 million AUD. Payments are exchanged every year, with one exchange having just taken place. The swap will last two more years. What is the value of the swap to the financial institution? Assume all interest rates are continuously compounded. The financial institution is long a dollar bond and short a USD bond. The value of the dollar bond (in millions of dollars) is 0.48+12.489=11.297 The value of the AUD bond (in millions of AUD) is 1.67+21.67=19.504 The value of the swap (in millions of dollars) is therefore 11.297-19.504 x0.62 = -0.795 or -$795,000. As an alternative we can value the swap as a series of forward foreign exchange contracts. The one-year forward exchange rate is 0.62" =0.6077 . The two-year forward exchange rate is 0.62.=0.5957 . The value of the swap in millions of dollars is therefore (0.48 -1.6*0.6077)+ (12.48-21.6*0.5957)=-0.795 which is in agreement with the first calculation. O34. Assume that at time zero there is a rich country and a poor country. You are in a Solow world without technical progress. When the countries are observed at a later date, they are characterized by the same standard of living. Is this corsistent with [a] absolute convergence; {in} conditional convergence? Specify your assumptions about technology. demography. tastes and policy. 37) A policyholder owns a variable annuity contract with death benefit features defined as follows: (a) Guaranteed minimum death benefit (GMDB) with return of premium: the greater of the account value and the initial investment will be paid when the policyholder dies. (b) Enhanced-income death benefit guarantee: 20% of the account value in excess of the initial investment amount will be added if the account value is greater than the initial investment when the policyholder dies. Let 7 be the random variable denoting the future lifetime of the policyholder. Let K be the initial investment amount of the variable annuity contract. Let S, be the value of the policyholder's account at time t. You are given: 7 follows a distribution with probability density function f (1),/ > 0. . Given T = t, p(1) is the payoff of a European put option based on account value S, with strike price K. . Given T= 1, c(f) is the payoff of a European call option based on account value S, with strike price K. Determine which one of the following statements is true. (A) The total death benefit payout for death at time f can be expressed as max( S, , K ) +0.2x max(K - S,,0). (B) The total death benefit payout for death at time / can be expressed as max( S, - K, 0) +02 x [" P(of(1)di . (9) The expected value of the death benefit can be expressed as K + [" c()f (1)d +0.2x [" p()f (1)dt. (D) The expected value of the death benefit can be expressed as K + [ " p( )f ( )dt + 0.2 x[" c()f (1)dt. (E) The expected value of the death benefit can be expressed as K +1.2x [ c(1)f (1)di .43. You are given: i) An investor short-sells a non-dividend paying stock that has a current price of 44 per share. ii) This investor also writes a collar on this stock consisting of a 40-strike European put option and a 50-strike European call option. Both options expire in one year. ifi) The prices of the options on this stock are: Strike Price Call option Put option 40 8.42 2.47 50 3.86 7.42 iv) The continuously compounded risk-free interest rate is 5%. Assume there are no transaction costs. Calculate the maximum profit for the overall position at expiration. (A) 2.61 (B) 3.37 (C) 4.79 (D) 5.21 (E) 7.39 IFM-01-18 Page 22 of 105 44. You are given the following information about two options, A and B: Option A is a one-year European put with exercise price 45. ii) Option B is a one-year American call with exercise price 55. iii) Both options are based on the same underlying asset, a stock that pays no dividends. iv) Both options go into effect at the same time and expire at f = 1. You are also given the following information about the stock price: i) The initial stock price is 50. ii) The stock price at expiration is also 50. iii) The minimum stock price (from / = 0 to f = 1) is 46. iv) The maximum stock price (from / = 0 to / = 1) is 58.a] Consumption will rise by relatively less in response to lower interest rates: a steeper IS curve [see Fig. 7.3). b] An easing of liquidity constraints will allow a number of households to borrow against future income. This will raise the MPG and make the IS curve flatter [pivots on the raxis - see Fig. 2.15]. c} Precautionary savings will go up. This would reduce the MPG and the size of the multiplier. IS curve becomes steeper {pivots on the r axis see Fig. 2.16]. d} This would reduce consumption. lowering permanent income. IS curve shifts left. The above answers can be related to the estimates presented in Section 1.1. 6. Compare the investment function of Chapter 2 [I = A b*rj with the investment function in section 2.5 [I = A - bus + b.*y]. Why is the additional output term in the equation? How would the following affect the IS curve {oeteris paribus]: {a} increased sales [b] a change in competition law that will allow firms to exploit more economies of scale opportunities in the future. to] a fall in corporation tax {d} afall in the interest rate {e} higher interest-sersitivity of investment {f} higher output-sensitivity of investment






















