Question
Show your work to earn credit! Due on Thursday, February 1, 2024. Let N(t) be the size of a population of fish. Assume this population
Show your work to earn credit! Due on Thursday, February 1, 2024.\ Let
N(t)be the size of a population of fish. Assume this population grows logistically and that the fish are harvested at the constant rate
h,\
(dN)/(dt)=f(N)-h=rN(1-(N)/(K))-h\
Kis the carrying capacity,
ris the intrinsic rate of growth, and
h, the harvest rate, is a bifurcation parameter. Sketch the bifurcation diagram for this system. Be sure to identify the stability of each branch of your bifurcation diagram. For what value of
his there a bifurcation? What kind of bifurcation is this? Describe the long-term behavior of the fish population for harvest rates on either side of this bifurcation. Is there something wrong with this model?
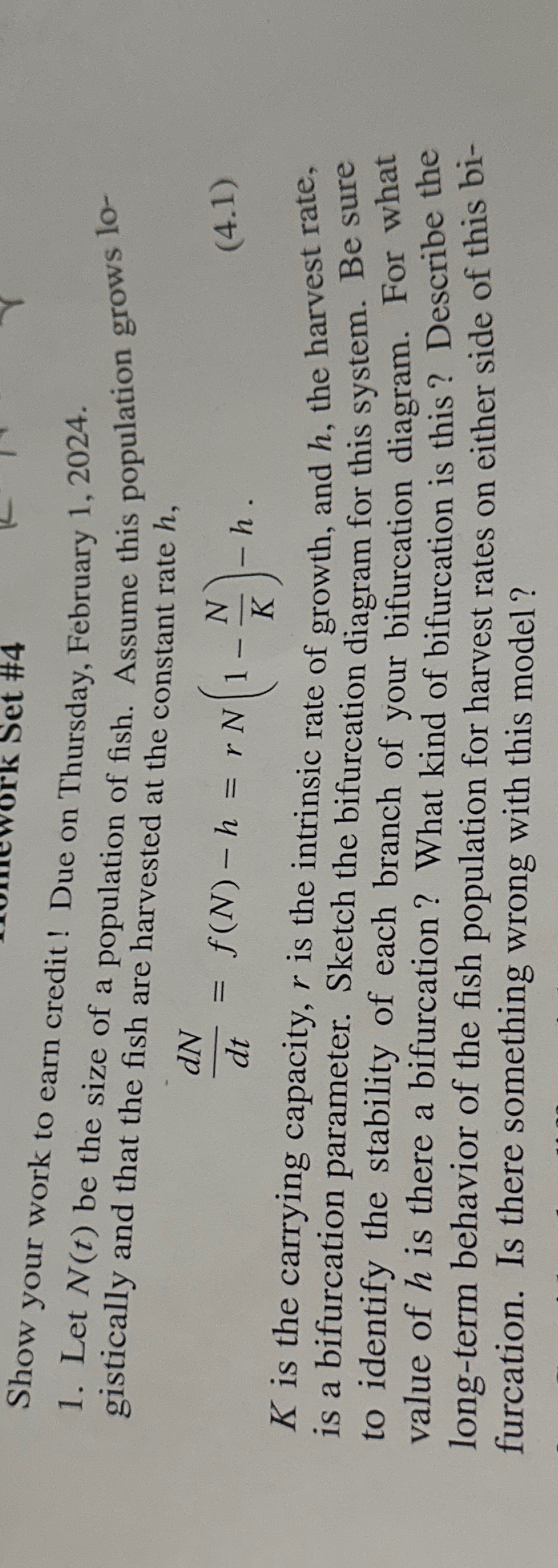
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


