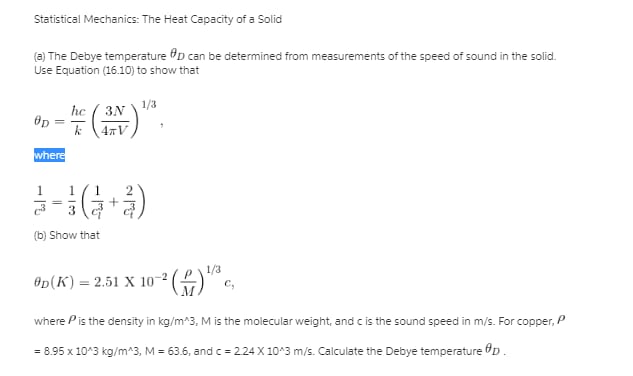
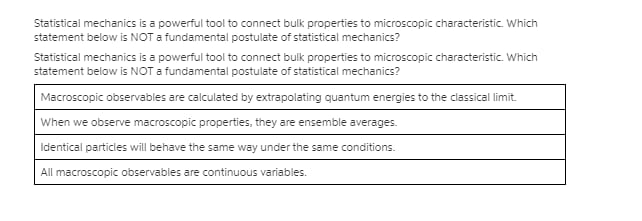
Solve ameakably

Enter Name of reactants and products: Did Precipitate form in the simulation? lead (II) nitrate + sodium sulfide Balanced Total equation (Molecular equation) if so, what is the name of the precipitate? E Net lanie equation: What color was the precipitate? Net ionic equation: Name of reactants and products: Did Precipitate form in the simulation? copper(II) nitrate + sodium iodide > Balanced Total equation (Molecular equation) If so, what is the name of the precipitate" F Net fonic equation: What color was the precipitate? Net ionic equation: Name of reactants and products: Did Precipitate form in the simulation? Balanced Total equation (Molecular equation) If ser, what is the name of the Ca(NO, )(ag) + Nal(ag) > precipitate? G Net fonic equation: What color was the precipitate? Net ionic equation: Page | 53. Metal coordination compounds are difficult system for molecular mechanics methods. Why is this? (Please discuss this in detail.) 4. What are the advantages and disadvantages of semi-empirical methods in term of transferability from one set of compounds to another, and in term of comparing chemically disparate system? 5. Name and explain six energy terms included in molecular mechanics?Statistical Mechanics: The Heat Capacity of a Solid (a) The Debye temperature #D can be determined from measurements of the speed of sound in the solid. Use Equation (16.10) to show that he 3N 1/3 0D = where 091 # + (b) Show that 1/3 Op(K) = 2.51 X 10 2 P C, where is the density in kg/m^3, M is the molecular weight, and c is the sound speed in m/s. For copper, P = 8.95 x 10*3 kg/m^3, M = 63.6, and c = 2.24 X 10 3 m/s. Calculate the Debye temperature D .Statistical mechanics is a powerful tool to connect bulk properties to microscopic characteristic. Which statement below is NOT a fundamental postulate of statistical mechanics? Statistical mechanics is a powerful tool to connect bulk properties to microscopic characteristic. Which statement below is NOT a fundamental postulate of statistical mechanics? Macroscopic observables are calculated by extrapolationg quantum energies to the classical limit. When we observe macroscopic properties, they are ensemble averages. Identical particles will behave the same way under the same conditions. All macroscopic observables are continuous variables