!! Solve by Mathematica ONLY !! For Question 2

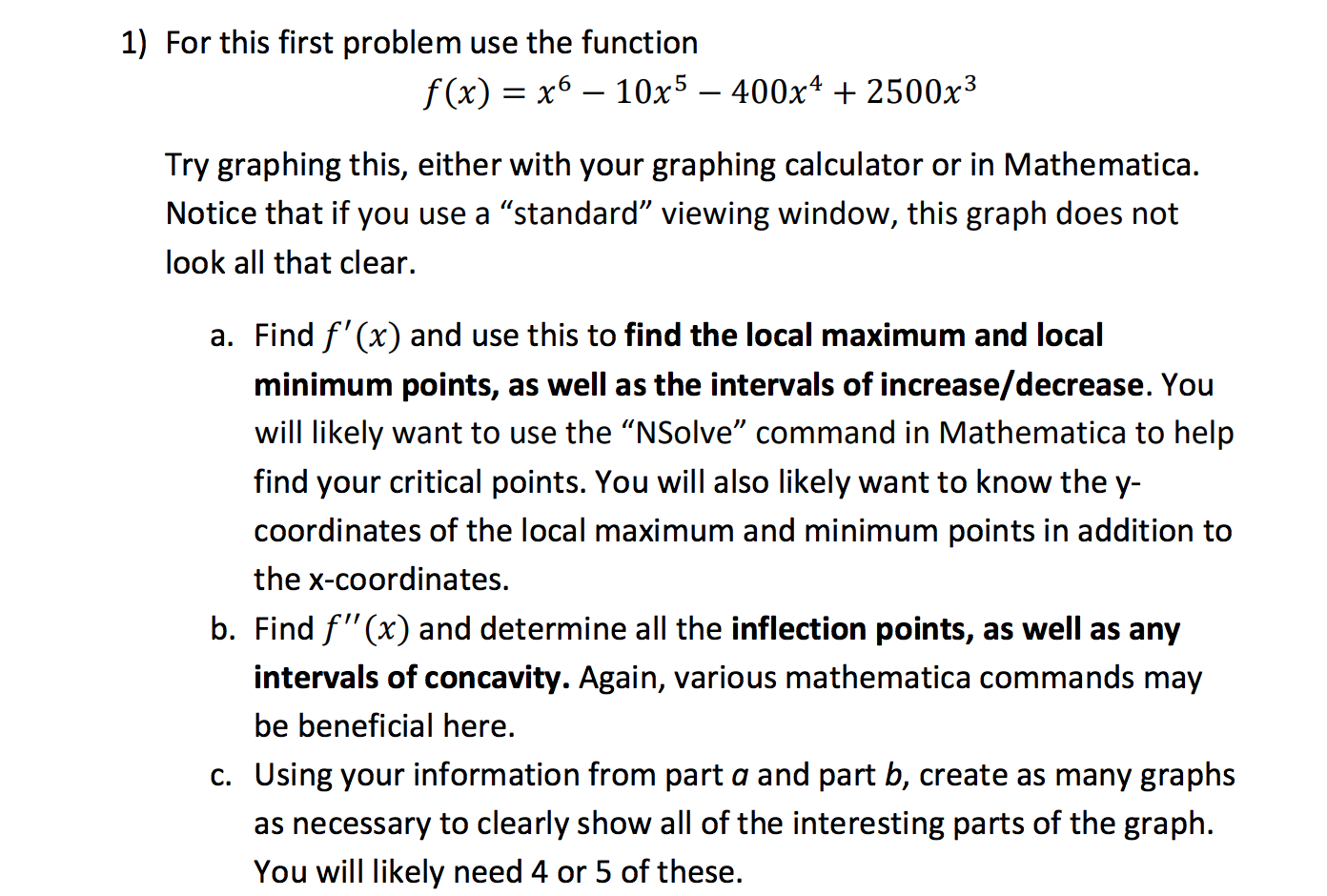
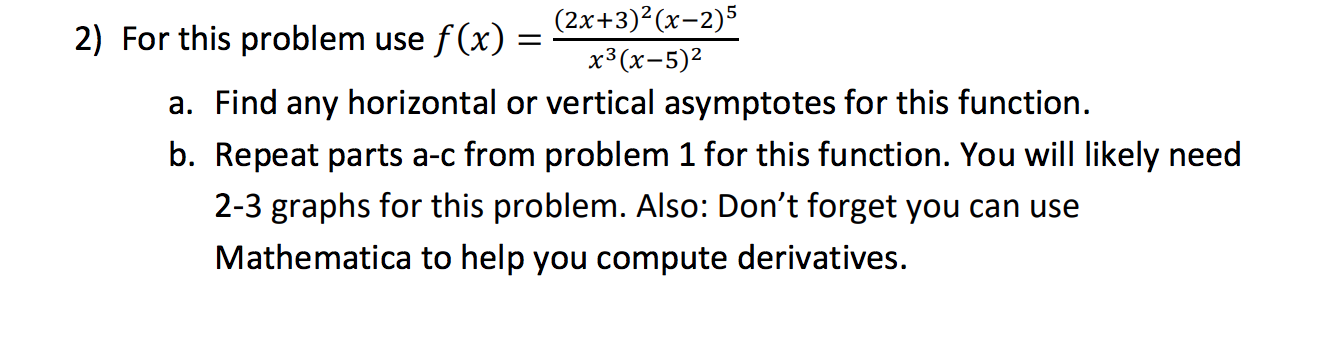
1) For this first problem use the function f(x) = x6 10x5 400x4 + 2500x3 Try graphing this, either with your graphing calculator or in Mathematica. Notice that if you use a standard viewing window, this graph does not look all that clear. a. Find f'(x) and use this to find the local maximum and local minimum points, as well as the intervals of increase/decrease. You will likely want to use the NSolve command in Mathematica to help find your critical points. You will also likely want to know the y- coordinates of the local maximum and minimum points in addition to the x-coordinates. b. Find f'(x) and determine all the inflection points, as well as any intervals of concavity. Again, various mathematica commands may be beneficial here. C. Using your information from part a and part b, create as many graphs as necessary to clearly show all of the interesting parts of the graph. You will likely need 4 or 5 of these. (2x+3)2(x-2)5 2) For this problem use f(x) = x3(x-5)2 a. Find any horizontal or vertical asymptotes for this function. b. Repeat parts a-c from problem 1 for this function. You will likely need 2-3 graphs for this problem. Also: Don't forget you can use Mathematica to help you compute derivatives. 1) For this first problem use the function f(x) = x6 10x5 400x4 + 2500x3 Try graphing this, either with your graphing calculator or in Mathematica. Notice that if you use a standard viewing window, this graph does not look all that clear. a. Find f'(x) and use this to find the local maximum and local minimum points, as well as the intervals of increase/decrease. You will likely want to use the NSolve command in Mathematica to help find your critical points. You will also likely want to know the y- coordinates of the local maximum and minimum points in addition to the x-coordinates. b. Find f'(x) and determine all the inflection points, as well as any intervals of concavity. Again, various mathematica commands may be beneficial here. C. Using your information from part a and part b, create as many graphs as necessary to clearly show all of the interesting parts of the graph. You will likely need 4 or 5 of these. (2x+3)2(x-2)5 2) For this problem use f(x) = x3(x-5)2 a. Find any horizontal or vertical asymptotes for this function. b. Repeat parts a-c from problem 1 for this function. You will likely need 2-3 graphs for this problem. Also: Don't forget you can use Mathematica to help you compute derivatives