Solve the following questions..

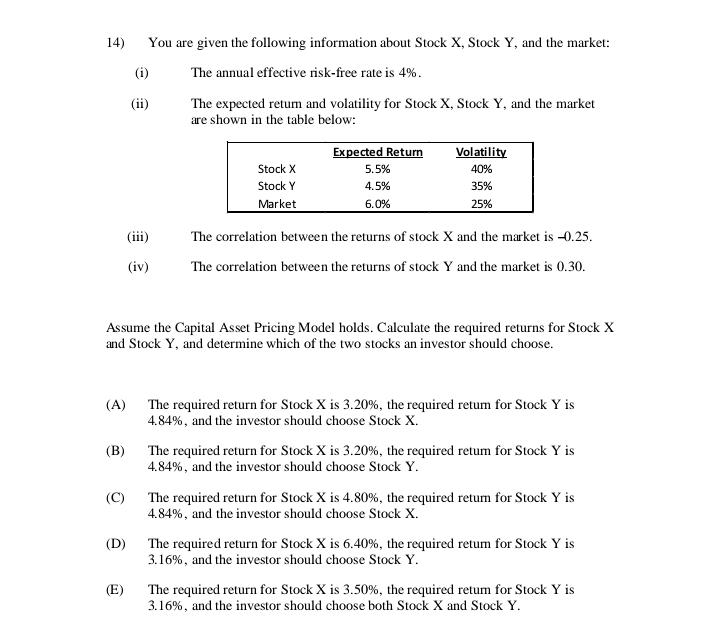
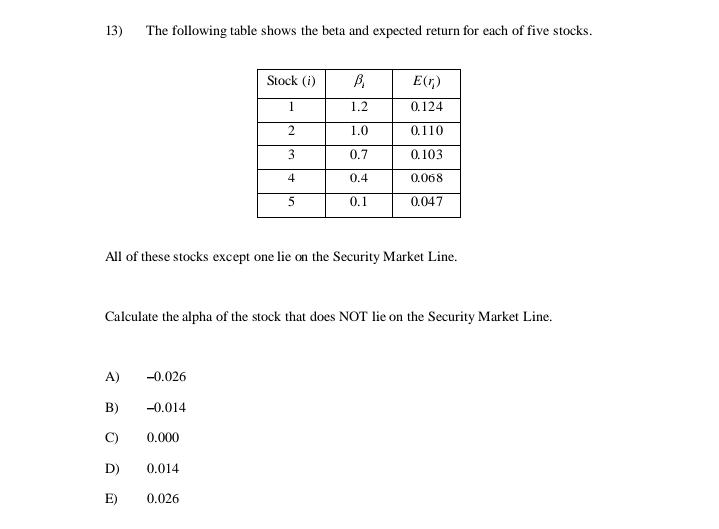
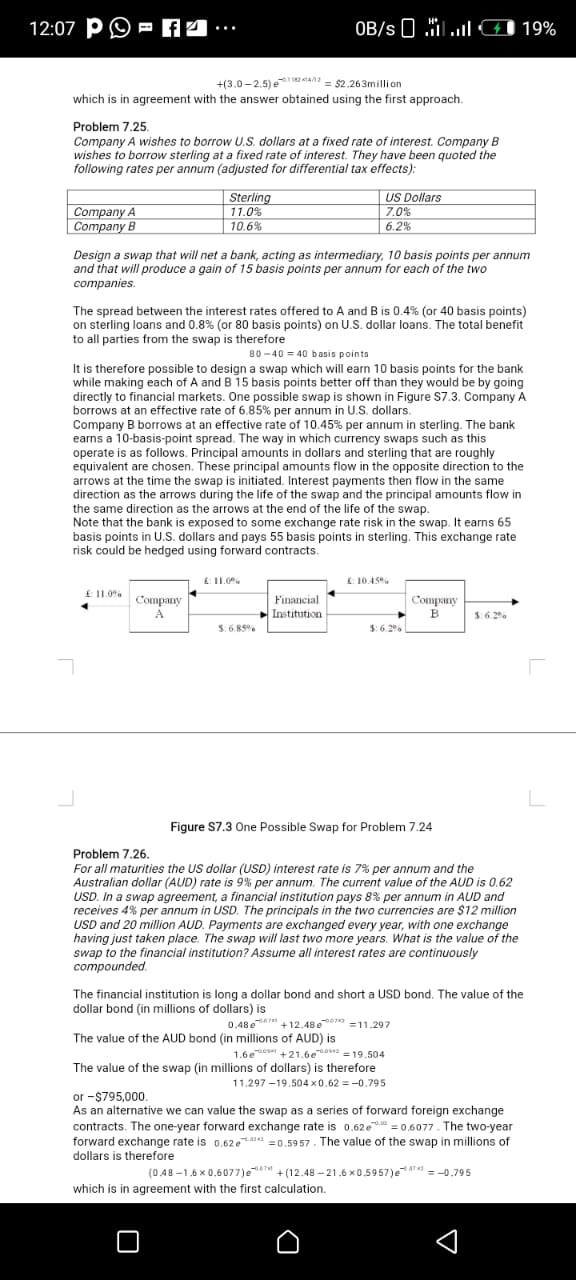
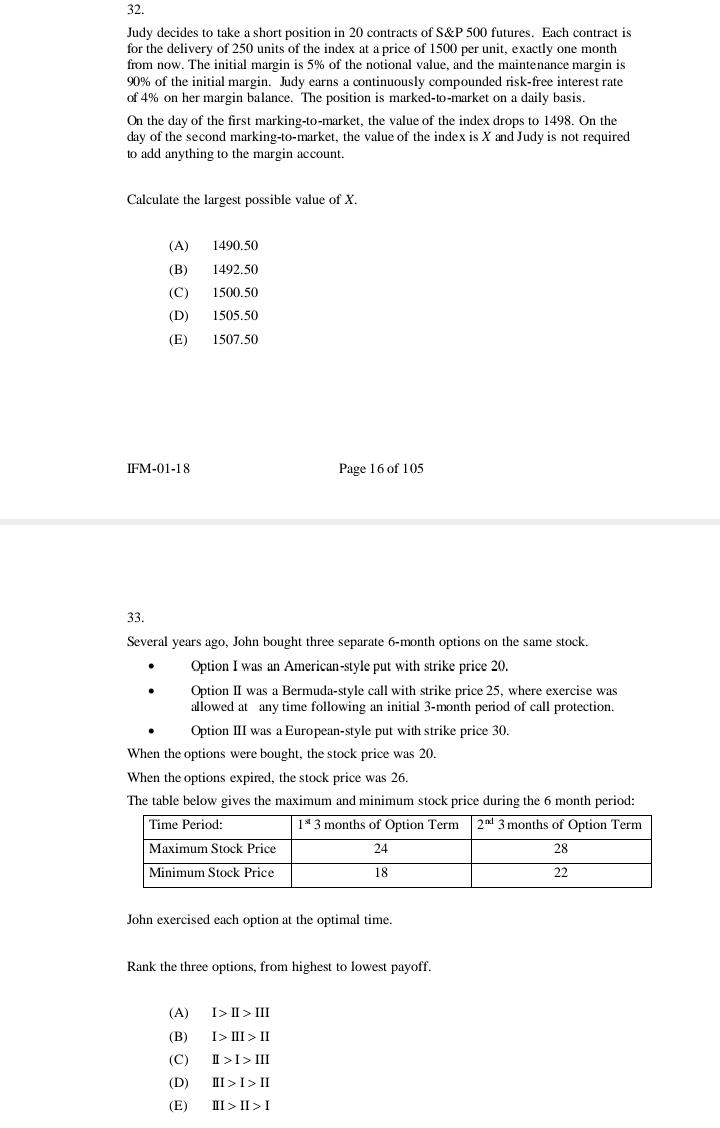
14) You are given the following information about Stock X, Stock Y, and the market: (i) The annual effective risk-free rate is 4%. (ii) The expected return and volatility for Stock X, Stock Y, and the market are shown in the table below: Expected Return Volatility Stock X 5.5% 40% Stock Y 4.5% 35% Market 6.0% 25% (iii) The correlation between the returns of stock X and the market is -0.25. (iv) The correlation between the returns of stock Y and the market is 0.30. Assume the Capital Asset Pricing Model holds. Calculate the required returns for Stock X and Stock Y, and determine which of the two stocks an investor should choose. (A) The required return for Stock X is 3.20%, the required return for Stock Y is 4.84%, and the investor should choose Stock X. (B) The required return for Stock X is 3.20%, the required return for Stock Y is 4.84%, and the investor should choose Stock Y. (C) The required return for Stock X is 4.80%, the required return for Stock Y is 4.84%, and the investor should choose Stock X. (D) The required return for Stock X is 6.40%, the required return for Stock Y is 3.16%, and the investor should choose Stock Y. (E) The required return for Stock X is 3.50%, the required return for Stock Y is 3.16%, and the investor should choose both Stock X and Stock Y.1. Consider a physician who receives m. dollars in income per unit of service she provides. The physician values her income, her leisure time, and her patients\" health. The physician may choose to induce I 3 units of services. If the physician induces no services, she prml'ides Q0 :5 units of services. (a) Show the physician's budget set and an indifference curve on a diagram, placing I on the x-axis and the physician's income on the yaxis. Label all axes, intercepts, and curves. [b] Assume the physician prefers to induce a strictly positive number of services. Show her optimal allocation on a new version of the diagram and label it A. Show the indifference curve passing through point A. (c) Interpret the slope of the indifference curve evaluated at point A and state the physician's equilibrium ccmdition. [d] Un another new version of the diagram, display the physician's new budget set if the fee, so, decreases. [e] [In the same diagram as in part (d), display the physician's new optimal allocation at the lower fee, assuming the physician sets I :5 , and label this point B. Carefully display the income and substitution clients on inducement caused by the fee change, assuming their signs are as we assinned in the lectiu'es. 13) The following table shows the beta and expected return for each of five stocks. Stock (i) B, E(r,) 1.2 0. 124 2 1.0 0.110 3 0.7 0.103 4 0.4 0.068 5 0.1 0.047 All of these stocks except one lie on the Security Market Line. Calculate the alpha of the stock that does NOT lie on the Security Market Line. A) -0.026 B) -0.014 0.000 D) 0.014 E) 0.02612:07 p - f 4 ... OB/s O Mil .ill 1 19% +(3.0-2.5)= $2.263million which is in agreement with the answer obtained using the first approach. Problem 7.25. Company A wishes to borrow U.S. dollars at a fixed rate of interest. Company B wishes to borrow sterling at a fixed rate of interest. They have been quoted the following rates per annum (adjusted for differential tax effects): Sterling US Dollars Company A 11.0% 7.0% Company B 10.6% 6.2% Design a swap that will net a bank, acting as intermediary, 10 basis points per annum and that will produce a gain of 15 basis points per annum for each of the two companies. The spread between the interest rates offered to A and B is 0.4% (or 40 basis points) on sterling loans and 0.8% (or 80 basis points) on U.S. dollar loans. The total benefit to all parties from the swap is therefore 80 -40 = 40 basis points It is therefore possible to design a swap which will earn 10 basis points for the bank while making each of A and B 15 basis points better off than they would be by going directly to financial markets. One possible swap is shown in Figure S7.3. Company A borrows at an effective rate of 6.85% per annum in U.S. dollars. Company B borrows at an effective rate of 10.45% per annum in sterling. The bank earns a 10-basis-point spread. The way in which currency swaps such as this operate is as follows. Principal amounts in dollars and sterling that are roughly equivalent are chosen. These principal amounts flow in the opposite direction to the arrows at the time the swap is initiated. Interest payments then flow in the same direction as the arrows during the life of the swap and the principal amounts flow in the same direction as the arrows at the end of the life of the swap. Note that the bank is exposed to some exchange rate risk in the swap. It earns 65 basis points in U.S. dollars and pays 55 basis points in sterling. This exchange rate risk could be hedged using forward contracts. (: 10.150% 6: 11.0% Company Financial Company A Institution B 5:6.85% $:6.20% L Figure S7.3 One Possible Swap for Problem 7.24 Problem 7.26. For all maturities the US dollar (USD) interest rate is 7% per annum and the Australian dollar (AUD) rate is 9% per annum. The current value of the AUD is 0.62 USD. In a swap agreement, a financial institution pays 8% per annum in AUD and receives 4% per annum in USD. The principals in the two currencies are $12 million USD and 20 million AUD. Payments are exchanged every year, with one exchange having just taken place. The swap will last two more years. What is the value of the swap to the financial institution? Assume all interest rates are continuously compounded. The financial institution is long a dollar bond and short a USD bond. The value of the dollar bond (in millions of dollars) is 0.48+12.489=11.297 The value of the AUD bond (in millions of AUD) is 1.67+21.67=19.504 The value of the swap (in millions of dollars) is therefore 11.297-19.504 x0.62 = -0.795 or -$795,000. As an alternative we can value the swap as a series of forward foreign exchange contracts. The one-year forward exchange rate is 0.62" =0.6077 . The two-year forward exchange rate is 0.62.=0.5957 . The value of the swap in millions of dollars is therefore (0.48 -1.6*0.6077)+ (12.48-21.6*0.5957)=-0.795 which is in agreement with the first calculation. O32. Judy decides to take a short position in 20 contracts of S&P 500 futures. Each contract is for the delivery of 250 units of the index at a price of 1500 per unit, exactly one month from now. The initial margin is 5% of the notional value, and the maintenance margin is 90% of the initial margin. Judy earns a continuously compounded risk-free interest rate of 4% on her margin balance. The position is marked-to-market on a daily basis. On the day of the first marking-to-market, the value of the index drops to 1498. On the day of the second marking-to-market, the value of the index is X and Judy is not required to add anything to the margin account. Calculate the largest possible value of X. (A) 1490.50 (B) 1492.50 (C) 1500.50 (D) 1505.50 (E) 1507.50 IFM-01-18 Page 16 of 105 33. Several years ago, John bought three separate 6-month options on the same stock. Option I was an American-style put with strike price 20. Option II was a Bermuda-style call with strike price 25, where exercise was allowed at any time following an initial 3-month period of call protection. Option III was a European-style put with strike price 30. When the options were bought, the stock price was 20. When the options expired, the stock price was 26. The table below gives the maximum and minimum stock price during the 6 month period: Time Period: 1* 3 months of Option Term 2" 3 months of Option Term Maximum Stock Price 24 28 Minimum Stock Price 18 22 John exercised each option at the optimal time. Rank the three options, from highest to lowest payoff. (A) I> II > III (B) I > III > II (C) I > I > III (D) III > I> II (E) III > II >