solve the question
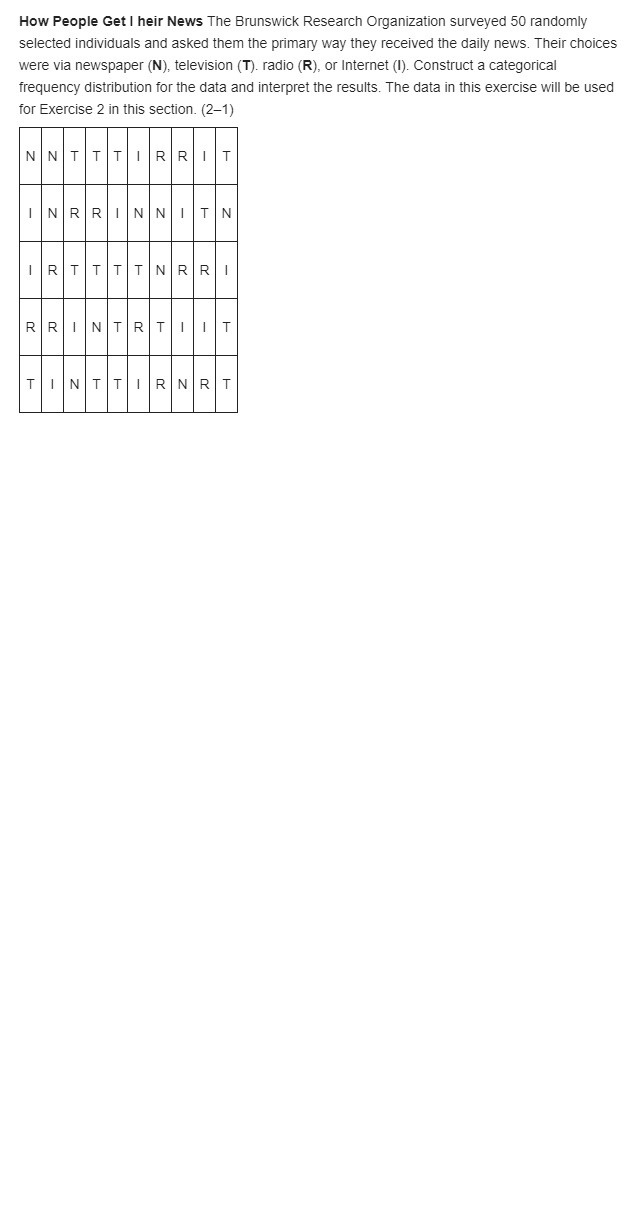
HOW People Getl heir News The Brunswick Research Organization surveved 50 randomly,r selected individuals and asked them the pmal'f wag.r they received the daily news. Their choices were via newspaper {N}, television (Ti. radio (R), or lntemet {I}. Construct a categorical frequency.r distribution forthe data and interpret the results. The data in this exercise will be used for Exercise 2 in this secon. [21] A result called Chebyshev's inequality states that for any probability distribution of an rv X and any number k that is at least 1, P( | X - p | k o) s 1/k . In words, the probability that the value of X lies at least k standard deviations from its mean is at most 1/12. a. What is the value of the upper bound for k = 2? K + 3? K = 4? K= 5? K = 10? b. Compute p and o for the distribution of Exercise 13. Then evaluate P(IX - p| > ko) for the values of k given in part (a). What does this suggest about the upper bound relative to the corresponding probability? c. Let X have possible values -1, 0, and 1, with probabilities 1/18, 8/9 and 1/8 , respectively. What is P(IX - p/2 30), and how does it compare to the corresponding bound? d. Give a distribution for which P(IX - p|2 50) = .04. Reference exercise -13 A mail-order computer business has six telephone lines. Let X denote the number of lines in use at a specified time. Suppose the pmf of X is as given in the accompanying table. P(| X - # | > ko) s 1/k2. Calculate the probability of each of the following events. a. {at most three lines are in use} b. {fewer than three lines are in use} c. {at least three lines are in use} d. {between two and five lines, inclusive, are in use} e. {between two and four lines, inclusive, are not in use} f. {at least four lines are not in use}Exercise 30 (Section 3.3) gave the pmf of Y, the number of traffic citations for a randomly selected individual insured by a particular company. What is the probability that among 15 randomly chosen such individuals a. At least 10 have no citations? b. Fewer than half have at least one citation? c. The number that have at least one citation is between 5 and 10, inclusive?* Reference exercise 30 An individual who has automobile insurance from a certain company is randomly selected. Let Y be the number of moving violations for which the individual was cited during the last 3 years. The 0 1 2 3 pmf of Y is P(y) .60 .25 .10 .05 a. Compute E(Y). b. Suppose an individual with Y violations incurs a surcharge of $100. Calculate the expected amount of the surcharge.An ordinance requiring that a smoke detector be installed in all previously constructed houses has been in effect in a particular city for 1 year. The fire department is concerned that many houses remain without detectors. Let p = the true proportion of such houses having detectors, and suppose that a random sample of 25 homes is inspected. If the sample strongly indicates that fewer than 80% of all houses have a detector, the fire department will campaign for a mandatory inspection program. Because of the costliness of the program, the department prefers not to call for such inspections unless sample evidence strongly argues for their necessity. Let X denote the number of homes with detectors among the 25 sampled. Consider rejecting the claim that p 2 . 8 if x s 15. a. What is the probability that the claim is rejected when the actual value of p is .8? b. What is the probability of not rejecting the claim when p = .7? when p = .6? c. How do the "error probabilities" of parts (a) and (b) change if the value 15 in the decision rule is replaced by 14?Using the experiment in Example 3.3, define two more random variables and list the possible values of each. Reference example 3.3 Example 2.3 described an experiment in which the number of pumps in use at each of two six- pump gas stations was determined. Define rv's X, Y, and U by X= the total number of pumps in use at the two stations Y =the difference between the number of pumps in use at station 1 and the number in use at station 2 U= the maximum of the numbers of pumps in use at the two stations If this experiment is performeds= (2,3) and results, then X((2,3)) =2+3 =5 , so we say that the observed value of X was x= 5 . Similarly, the observed value of Y would be y =2-3 = -1, and the observed value of U would be u = max (2,3) -3. Each of the random variables of Examples 3.1-3.3 can assume only a finite number of possible values. This need not be the case.For each random variable defined here, describe the set of possible values for the variable, and state whether the variable is discrete. , a. X = the number of unbroken eggs in a randomly chosen standard egg carton b. Y = the number of students on a class list for a particular course who are absent on the first day of classes c. U = the number of times a duffer has to swing at a golf ball before hitting it d. X = the length of a randomly selected rattlesnake e. Z = the sales tax percentage for a randomly selected amazon.com purchase f. Y = the pH of a randomly chosen soil sample g. X = the tension (psi) at which a randomly selected tennis racket has been strung h. X = the total number of times three tennis players must spin their rackets to obtain something other than UUU or DDD (to determine which two play next)You have two lightbulbs for a particular lamp. Let X = the lifetime of the first bulb and Y = the lifetime of the second bulb (both in 1000s of hours). Suppose that X and Y are independent and that each has an exponential distribution with parameter A= 1. a. What is the joint pdf of X and Y? b. What is the probability that each bulb lasts at most 1000 hours (i.e., X's 1 and Y= 1)? c. What is the probability that the total lifetime of the two bulbs is at most 2? [Hint. Draw a picture of the region A = > = 1. before integrating.] d. What is the probability that the total lifetime is between 1 and 2