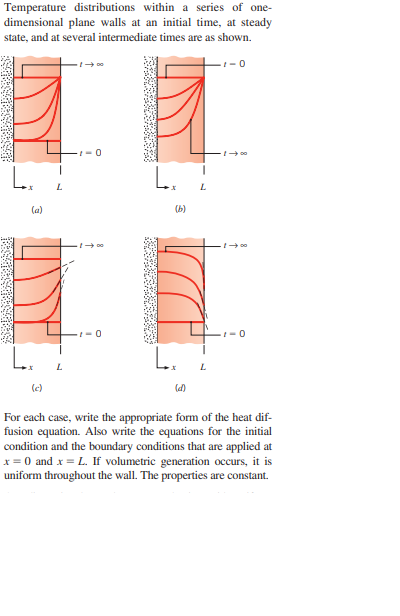
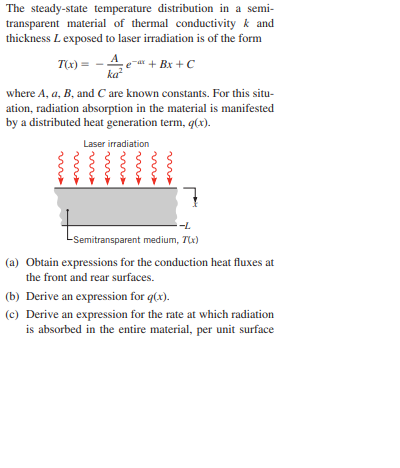
solve the question

Twenty adult males between the ages of 30 and 40 participated in a study to evaluate the effect of a specific health regimen involving diet and exercise on the blood cholesterol. Ten were randomly selected to be a control group, and ten others were assigned to take part in the regimen as the treatment group for a period of 6 months. The following data show the reduction in cholesterol experienced for the time period for the 20 subjects: Control group: 7 3 -4 14 2 522 -795 Treatment group: -6 5 9 4 4 12 37 5 3 3 (a) Do a dot plot of the data for both groups on the same graph. (b) Compute the mean, median, and 10% trimmed mean for both groups. (c) Explain why the difference in means suggests one conclusion about the effect of the regimen while the difference in medians or trimmed means suggests a different conclusion.Temperature distributions within a series of one- dimensional plane walls at an initial time, at steady state, and at several intermediate times are as shown. 1-0 L L (a) L L (c) (d) For each case, write the appropriate form of the heat dif- fusion equation. Also write the equations for the initial condition and the boundary conditions that are applied at x = 0 and x = L. If volumetric generation occurs, it is uniform throughout the wall. The properties are constant.The steady-state temperature distribution in a semi- transparent material of thermal conductivity & and thickness L exposed to laser irradiation is of the form T(x) = - 4 e- + Bx + C ka where A, a, B. and C are known constants. For this situ- ation, radiation absorption in the material is manifested by a distributed heat generation term, q(x). Laser irradiation -L Semitransparent medium, T(x) (a) Obtain expressions for the conduction heat fluxes at the front and rear surfaces. (b) Derive an expression for q(x). (c) Derive an expression for the rate at which radiation is absorbed in the entire material, per unit surfaceMeasurements of scientific systems are always subject to variation, some more than others. There are many structures for measurement error, and statisticians spend a great deal of time modeling these errors. Suppose the measurement error X of a certain physical quantity is decided by the density function f(x) = [*(3-r'), -15251, elsewhere. (a) Determine * that renders f(x) a valid density function.(b) Find the probability that a random error in measurement is less than 1/2.(c) For this particular measurement, it is undesirable if the magnitude of the error (ie., (x]) exceeds 0.8. What is the probability that this occurs? Five independent coin tosses result in HHHHH. It turns out that if the coin is fair the probability of this outcome is (1/2)5 = 0.03125. Does this produce strong evidence that the coin is not fair? Comment and use the concept of P-value discussed inSection 1.1.Suppose that X and Y have the following joint probability distribution: I f(r, y) 2 0.10 0.15 y 0.20 0.30 0.10 0.15 (a) Find the marginal distribution of X. (b) Find the marginal distribution of Y.The amount of kerosene, in thousands of liters, in a tank at the beginning of any day is a random amount Y from which a random amount X is sold during that day. Suppose that the tank is not resupplied during the day so that x sy, and assume that the joint density function of these variables is 12, 0