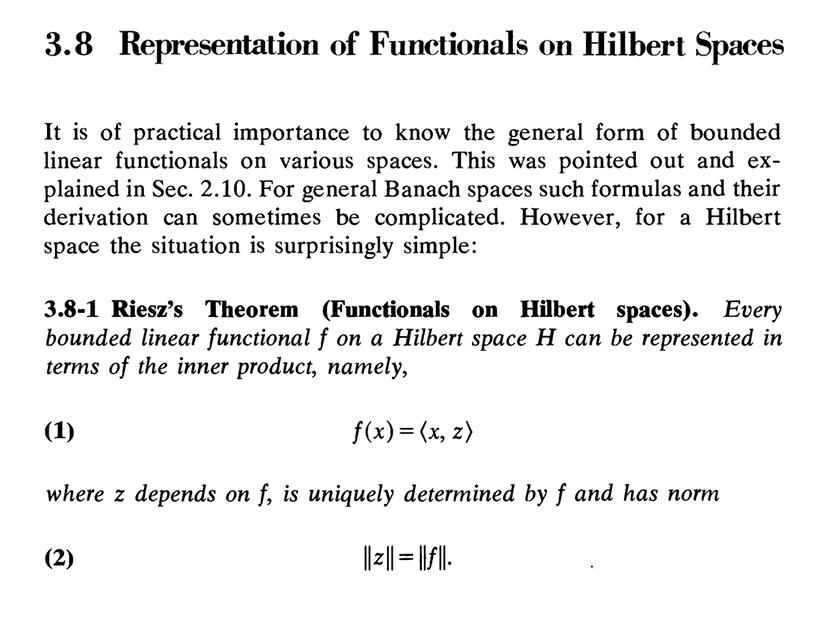
Show that the dual space of the real space l is l. (Use 3.8-1.) 3.8 Representation of Functionals on Hilbert Spaces It is of practical importance to know the general form of bounded linear functionals on various spaces. This was pointed out and ex- plained in Sec. 2.10. For general Banach spaces such formulas and their derivation can sometimes be complicated. However, for a Hilbert space the situation is surprisingly simple: 3.8-1 Riesz's Theorem (Functionals bounded linear functional f on a Hilbert space H can be represented in terms of the inner product, namely, on Hilbert spaces). Every (1) f(x) = (x, z) where z depends on f, is uniquely determined by f and has norm (2)