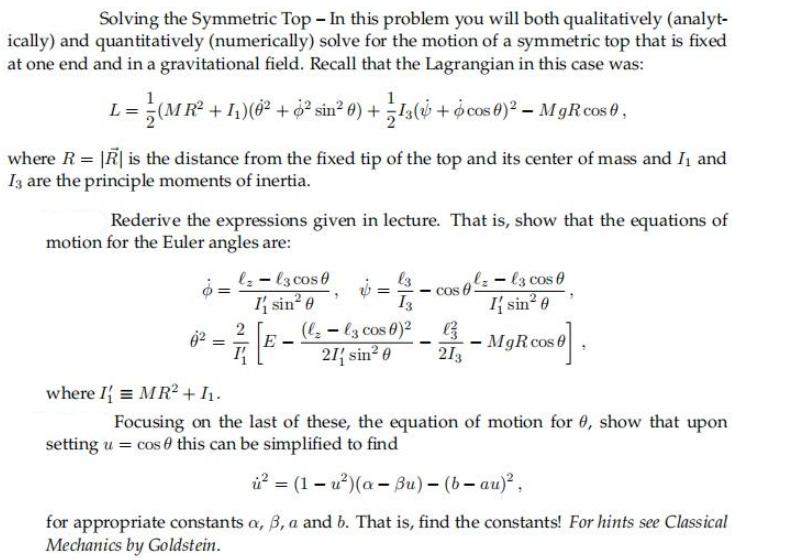
Solving the Symmetric Top - In this problem you will both qualitatively (analyt- ically) and quantitatively (numerically) solve for the motion of a symmetric top that is fixed at one end and in a gravitational field. Recall that the Lagrangian in this case was: L = (MR + 1) (0 + 6 sin 0)+1(+cos 0) - MgR cos, where R = |R| is the distance from the fixed tip of the top and its center of mass and I and I3 are the principle moments of inertia. Rederive the expressions given in lecture. That is, show that the equations of motion for the Euler angles are: lz-l3 cos 0 I sin0 2 E - l3 v= 13 (l-l3 cos 0) 21 sin0 8 -cos-l3 cos I sin0 lz 213 MgR cos e where I = MR + 1. Focusing on the last of these, the equation of motion for 0, show that upon setting u = cos this can be simplified to find i = (1-)(a-Bu)-(b-au), for appropriate constants a, 3, a and b. That is, find the constants! For hints see Classical Mechanics by Goldstein.