sos

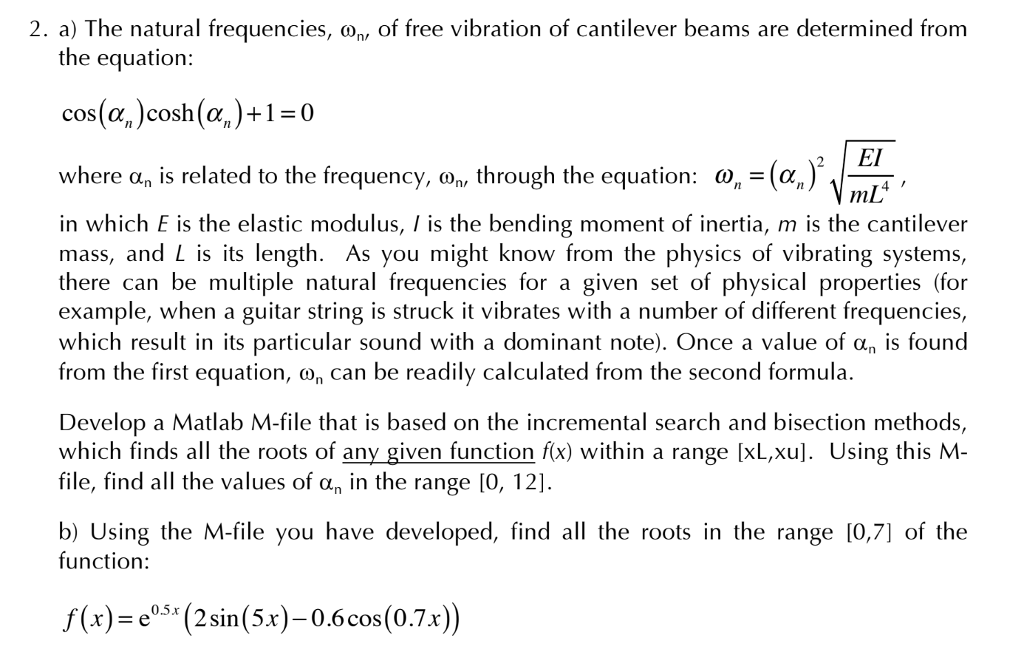
2. a) The natural frequencies, on, of free vibration of cantilever beams are determined from the equation: cos(" )cosh (" ) + 1-0 where is related to the frequ ency, on, through the equation: (0,-(a.) mL in which E is the elastic modulus, is the bending moment of inertia, m is the cantilever mass, and L is its length. As you might know from the physics of vibrating systems, there can be multiple natural frequencies for a given set of physical properties (for example, when a guitar string is struck it vibrates with a number of different frequencies, which result in its particular sound with a dominant note). Once a value of a is found from the first equation, can be readily calculated from the second formula. Develop a Matlab M-file that is based on the incremental search and bisection methods, which finds all the roots of any given function f(x) within a range [xL,xu]. Using this M file, find all the values of an in the range [0, 12] b) Using the M-file you have developed, find all the roots in the range [0,7] of the function: f(x)-e05. (2 sin(5x)-0.6 cos(0.7x)) 2. a) The natural frequencies, on, of free vibration of cantilever beams are determined from the equation: cos(" )cosh (" ) + 1-0 where is related to the frequ ency, on, through the equation: (0,-(a.) mL in which E is the elastic modulus, is the bending moment of inertia, m is the cantilever mass, and L is its length. As you might know from the physics of vibrating systems, there can be multiple natural frequencies for a given set of physical properties (for example, when a guitar string is struck it vibrates with a number of different frequencies, which result in its particular sound with a dominant note). Once a value of a is found from the first equation, can be readily calculated from the second formula. Develop a Matlab M-file that is based on the incremental search and bisection methods, which finds all the roots of any given function f(x) within a range [xL,xu]. Using this M file, find all the values of an in the range [0, 12] b) Using the M-file you have developed, find all the roots in the range [0,7] of the function: f(x)-e05. (2 sin(5x)-0.6 cos(0.7x))