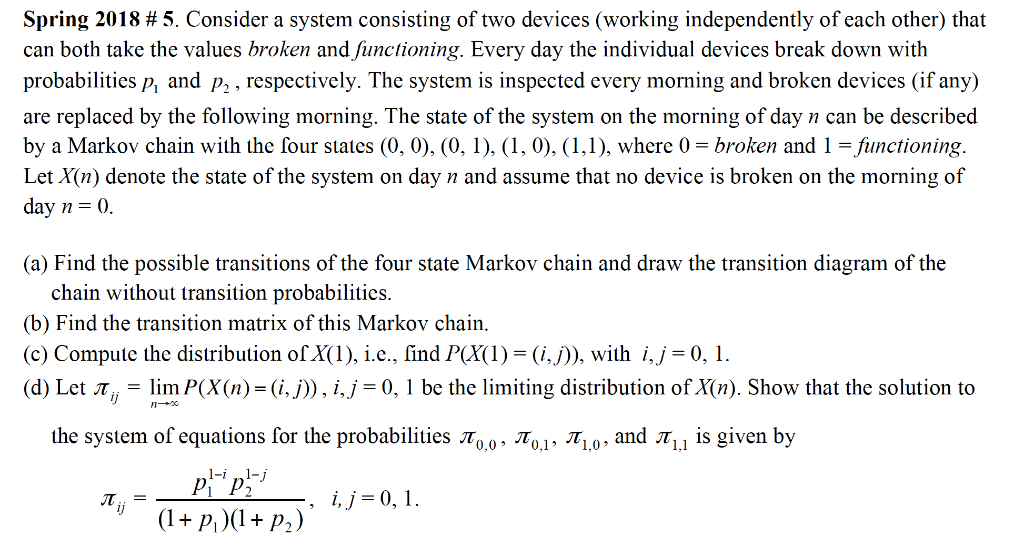
Spring 2018 # 5. Consider a system consisting of two devices (working independently of each other) that can both take the values broken and functioning. Every day the individual devices break down with probabilities p, and P2 , respectively. The system is inspected every morning and broken devices (if any) are replaced by the following morning. The state of the system on the morning of day n can be described by a Markov chain with the four states (0,0), (0, 1), (1, 0), (1,1), where () = broken and 1 = functioning. Let X(n) denote the state of the system on day n and assume that no device is broken on the morning of day n=0. (a) Find the possible transitions of the four state Markov chain and draw the transition diagram of the chain without transition probabilities. (b) Find the transition matrix of this Markov chain. (c) Compute the distribution of X(1), i.e., ind P(X(1) = (i, j)), with i, j = 0, 1. = lim P(X(n)=(i, j)), i, j=0, 1 be the limiting distribution of X(n). Show that the solution to (d) Let Tij the system of equations for the probabilities 10,0, 10,1, 11,0, and 11,1 is given by l- il-j i, j = 0,1. (1+ p.)(1+ p2) Spring 2018 # 5. Consider a system consisting of two devices (working independently of each other) that can both take the values broken and functioning. Every day the individual devices break down with probabilities p, and P2 , respectively. The system is inspected every morning and broken devices (if any) are replaced by the following morning. The state of the system on the morning of day n can be described by a Markov chain with the four states (0,0), (0, 1), (1, 0), (1,1), where () = broken and 1 = functioning. Let X(n) denote the state of the system on day n and assume that no device is broken on the morning of day n=0. (a) Find the possible transitions of the four state Markov chain and draw the transition diagram of the chain without transition probabilities. (b) Find the transition matrix of this Markov chain. (c) Compute the distribution of X(1), i.e., ind P(X(1) = (i, j)), with i, j = 0, 1. = lim P(X(n)=(i, j)), i, j=0, 1 be the limiting distribution of X(n). Show that the solution to (d) Let Tij the system of equations for the probabilities 10,0, 10,1, 11,0, and 11,1 is given by l- il-j i, j = 0,1. (1+ p.)(1+ p2)