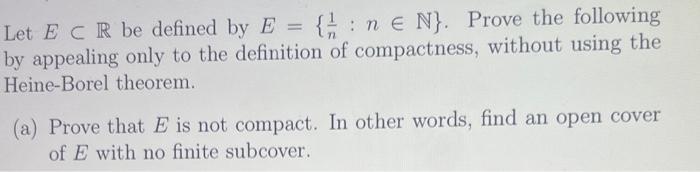
Let ECR be defined by E = { n E N}. Prove the following by appealing only to the definition of compactness, without using the Heine-Borel theorem. (a) Prove that E is not compact. In other words, find an open cover of E with no finite subcover.