STATISTICS. Help in all questions please
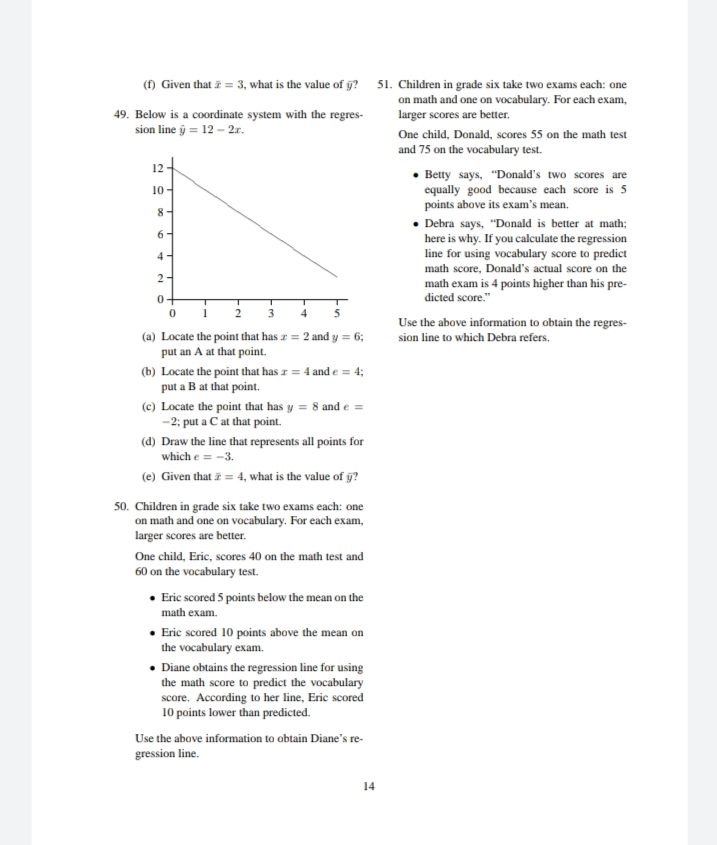
42. Independent random samples are selected from 46. Fifty students take midterm and final exams. two populations. Below are selected summary On the midterm exam, the mean score is 45.0 statistics. and the standard deviation is 7.00. On the final exam, the mean score is 85.0 with a standard Pop. Mean Stand. Dev. Sample size deviation of 14.00. The correlation coefficient 62.00 10.00 of the two scores is 0.64. 54.00 6.00 10 Obtain the least squares regression line for us- (a) Construct the 95% confidence interval for ing the final exam score to predict the midterm HX - HY. exam score. (b) Obtain the P-value for the alternative 47. Fifty students take two midterm exams. On the ux # my. Show your work. You will re- first exam, the mean score is 65.0 and the stan- ceive no credit for simply reporting your dard deviation is 7.00. On the second exam, answer. the mean score is 55.0 with a standard devia- tion of 10.00. The correlation coefficient of the 43. Independent random samples are selected from two scores is 0.70. two populations. Below are selected summary Obtain the least squares regression line for us- statistics. ing the second exam score to predict the first Pop. Mean Stand. Dev. Sample size exam score. 73.00 10.00 48. Below is a coordinate system with the regres- 62.50 6.00 sion line g = 12 - 2x. (a) Construct the 95% confidence interval for HX - HY 12 (b) Obtain the P-value for the alternative 10 44. A regression analysis yields the line 9 = 32 + 0.4x. 2 - One of the subjects, Racheal, has a = 60 and 1 = 52. (a) Calculate Racheal's predicted value, g. (a) Locate the point that has a = 3 and y = 8; (b) Calculate Racheal's residual. put an A at that point. 45. A regression analysis yields the line (b) Locate the point that has a = 5 and e = 2; put a B at that point. #= 18 + 0.25x. (c) Locate the point that has y = 6 and e = One of the subjects, Mary, has a = 40 and y = -2; put a C at that point. 32. (d) Locate the point that has y = 6 and e = -4; put a D at that point. (a) Calculate Mary's predicted value, g. (e) Draw the line that represents all points for (b) Calculate Mary's residual. which e = -2. 13(f) Given that 2 = 3, what is the value of y? 51. Children in grade six take two exams each: one on math and one on vocabulary. For each exam, 49. Below is a coordinate system with the regres- larger scores are better. sion line y = 12 - 2r. One child, Donald, scores 55 on the math test and 75 on the vocabulary test. 12 - . Betty says, "Donald's two scores are 10 - equally good because each score is 5 8 - points above its exam's mean. . Debra says, "Donald is better at math; 6 - here is why. If you calculate the regression line for using vocabulary score to predict math score, Donald's actual score on the N math exam is 4 points higher than his pre- dicted score." Use the above information to obtain the regres- (a) Locate the point that has a = 2 and y = 6; sion line to which Debra refers. put an A at that point. (b) Locate the point that has I = 4 and e = 4; put a B at that point. (c) Locate the point that has y = 8 and e = -2; put a C at that point. (d) Draw the line that represents all points for which e = -3. (e) Given that I = 4, what is the value of y? 50. Children in grade six take two exams each: one on math and one on vocabulary. For each exam, larger scores are better. One child, Eric, scores 40 on the math test and 60 on the vocabulary test. . Eric scored 5 points below the mean on the math exam. . Eric scored 10 points above the mean on the vocabulary exam. . Diane obtains the regression line for using the math score to predict the vocabulary score. According to her line, Eric scored 10 points lower than predicted. Use the above information to obtain Diane's re- gression line. 14









