Question
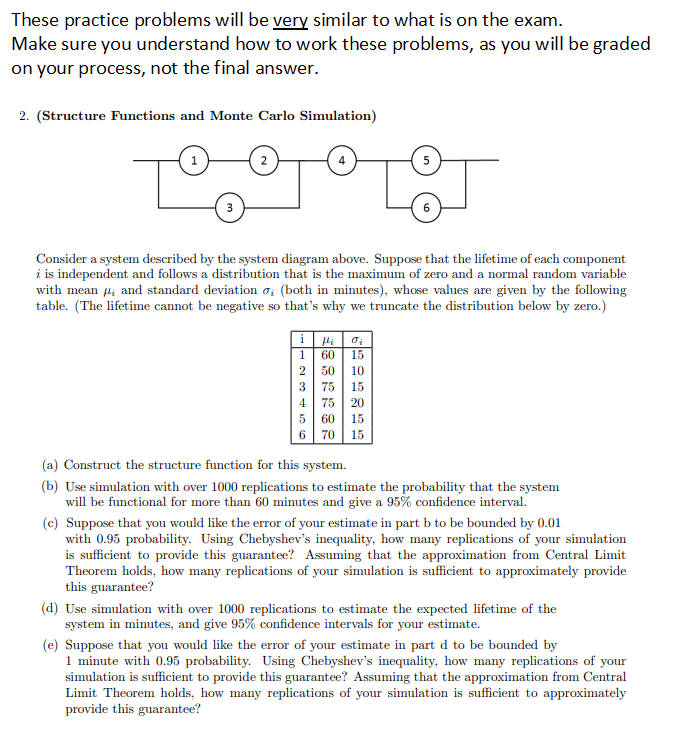
(Structure Functions and Monte Carlo Simulation) Consider a system described by the system diagram above. Suppose that the lifetime of each component i is independent
(Structure Functions and Monte Carlo Simulation) Consider a system described by the system diagram above. Suppose that the lifetime of each component i is independent and follows a distribution that is the maximum of zero and a normal random variable with mean i and standard deviation ?i (both in minutes), whose values are given by the following table.
(The lifetime cannot be negative so that's why we truncate the distribution below by zero.) i i ?i 1 60 15 2 50 10 3 75 15 4 75 20 5 60 15 6 70 15
(a) Construct the structure function for this system.
(b) Use simulation with over 1000 replications to estimate the probability that the system will be functional for more than 60 minutes and give a 95% confidence interval.
(c) Suppose that you would like the error of your estimate in part b to be bounded by 0.01 with 0.95 probability. Using Chebyshev's inequality, how many replications of your simulation is sufficient to provide this guarantee? Assuming that the approximation from Central Limit Theorem holds, how many replications of your simulation is sufficient to approximately provide this guarantee?
(d) Use simulation with over 1000 replications to estimate the expected lifetime of the system in minutes, and give 95% confidence intervals for your estimate.
(e) Suppose that you would like the error of your estimate in part d to be bounded by 1 minute with 0.95 probability. Using Chebyshev's inequality, how many replications of your simulation is sufficient to provide this guarantee? Assuming that the approximation from Central Limit Theorem holds, how many replications of your simulation is sufficient to approximately provide this guarantee?
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


