Question: Suppose a function f is defined by setting f(x) 32n+1x5n+7 (that is, f is defined to be the limit of the n=0 series, and

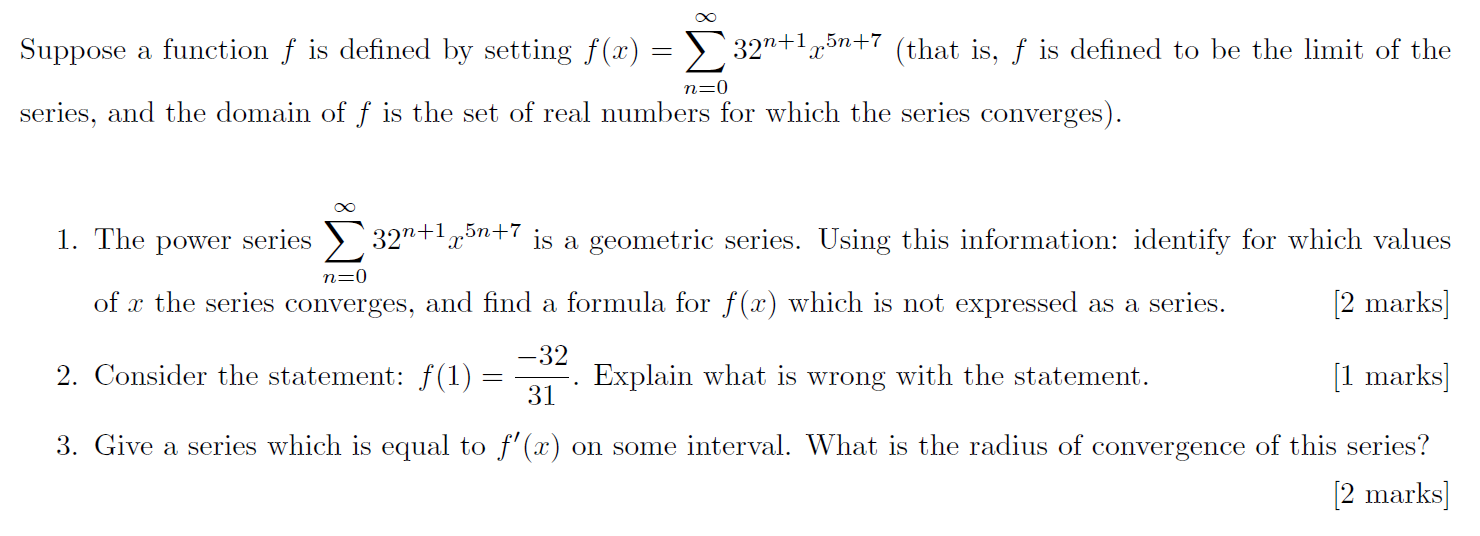
Suppose a function f is defined by setting f(x) 32n+1x5n+7 (that is, f is defined to be the limit of the n=0 series, and the domain of f is the set of real numbers for which the series converges). 1. The power series 32+1x5n+7 is a geometric series. Using this information: identify for which values n=0 of x the series converges, and find a formula for f(x) which is not expressed as a series. 2. Consider the statement: (1) = -32 31 Explain what is wrong with the statement. [2 marks] [1 marks] 3. Give a series which is equal to f'(x) on some interval. What is the radius of convergence of this series? [2 marks]

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Anomaly detection consist in identifying: A. The rate of curvature change of time series. B. All the parameters in the NDVI profile (SOS, EOS, LOS, etc.) C. Values or events that deviate from the...

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...

CANMNMM January of this year. (a) Each item will be held in a record. Describe all the data structures that must refer to these records to implement the required functionality. Describe all the...

MAT1510/101 ASSIGNMENT 03 Due Date: 17 June 2016 Total Marks: 100 UNIQUE ASSIGNMENT NUMBER: 736826 ONLY FOR YEAR MODULE STUDY MATERIAL: SRW and STUDY GUIDE: CHAPTER 4 The answers to only some of the...

Just need answers. PLEASE READ THE BLUE INSTRUCTIONS. 15. The function f is defined as follows. if x 0 ( x ) = X 1 if x = 0 (a) Find the domain of the function. (b) Locate any intercepts. (c) Graph...

PreAlgebra Review/Practice For each pair of functions f and g below, find f g (x)) and g (f (x)). Then, determine whether f and g are inverses of each other. Simplify your answers as much as...

The function f is defined as follows. 2x if x#0 f(x) =. 4 if x = 0 (a) Find the domain of the function. (b) Locate any intercepts (c) Graph the function. (d) Based on the graph, find the range. (a)...

Calculus 10.15 Representing Functions as Power Series CA #1 Name: 1. What is the coefficient of x5 in the Taylor series for the function f(x) = ex sin x about x = 0? If the function f is defined by f...

Suppose a function f is defined by the geometric series a. Evaluate f(0), f(0.2), f(0.5), f(1), and f(1.5), if possible. b. What is the domain of f? f(x) k=0 x 2k

Suppose a function f is defined by the geometric series a. Evaluate f(0), f(0.2), f(0.5), f(1), and f(1.5), if possible. b. What is the domain of f ? f(x) = Co x k k=0

Using long- run supply and demand curves, analyze the effects of an ad valorem excise tax equal to 20 percent of the market (selling) price of gasoline. How do the effects differ from those of the...

Rick was hired as Controller to help sort out and organize the records of a $7 million dollar medical supply firm. This company was recently extended a $1,000,000 small business loan to acquire the...

Ecplain why it is important to select a specific wavelength when performing laboratory testing using a spectrophotometer.

As a new owner of an accounting firm, you are looking into risk management policies. What exposure does the account firm have to professional liability? If you form a profession corporation, is the...

In what study design does the same person, or group, act as both the control group and experimental group? Select one: a. True experimental design b. Solomon four-group case study c. Equivalent time...

What do the "reactive effects of experimental arrangements" mean as a threat to external validity? Select one: a. It means that the data was collected in a setting that did not accurately mimic the...

15. In the context of fraud, explain the differences between (1) incentives and pressures, (2) opportunity, and (3) attitudes and rationalization. Why is it important for an auditor to consider...

A 4-year business loan was taken out commencing on 1 July 2017 the loan establishment fee was $1,600 the deduction of the borrowing expenses in the 2018 financial year should be select one a $1,600 b...

For your response posts, address the following:Comment on your classmates' posts and welcome them to the class.What additional ways do you think AI will affect the future of accounting within broader...

(a) If f(x) = (x3 - x) ex, find f'(x). (b) Check to see that your answer to part (a) is reasonable by comparing the graphs of f and f'.

The motion of a spring that is subject to a frictional force or a damping force (such as a shock absorber in a car) is often modeled by the product of an exponential function and a sine or cosine...

Explain why each of the following integrals is improper. a. b. c. d. cot x dx

=+a. Describe a procedure for calculating capability indexes from the transformed data.

=+22. Three-dimensional (3D) printing is a manufacturing technology that allows the production of three-dimensional solid objects through a meticulous layering process performed by a 3D printer. 3D...

=+b. Transform the data in this problem as described, and then create x and R charts of the transformed data. Use the extended list of out-of-control conditions (Figure 6.5) to evaluate these charts.