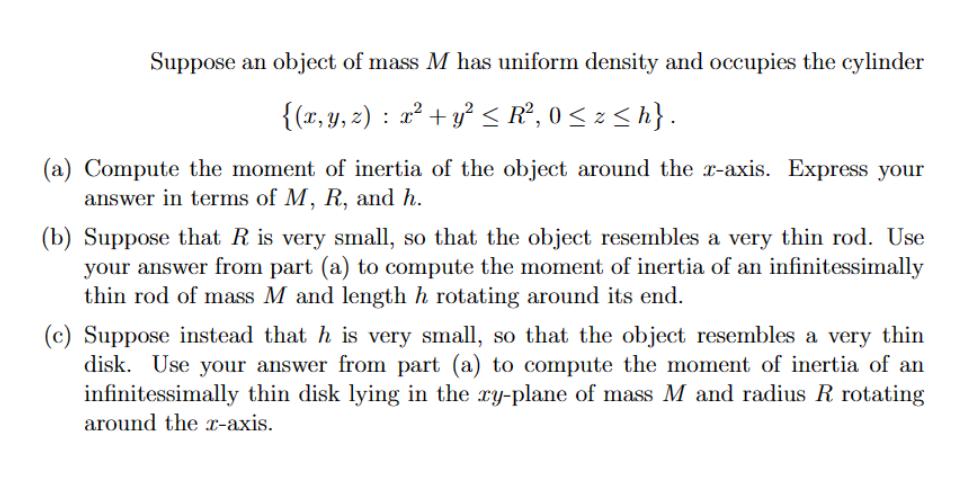
Suppose an object of mass M has uniform density and occupies the cylinder {(x, y, z) = x + y R,0 z h}. : (a) Compute the moment of inertia of the object around the x-axis. Express your answer in terms of M, R, and h. (b) Suppose that R is very small, so that the object resembles a very thin rod. Use your answer from part (a) to compute the moment of inertia of an infinitessimally thin rod of mass M and length h rotating around its end. (c) Suppose instead that h is very small, so that the object resembles a very thin disk. Use your answer from part (a) to compute the moment of inertia of an infinitessimally thin disk lying in the ry-plane of mass M and radius R rotating around the x-axis.