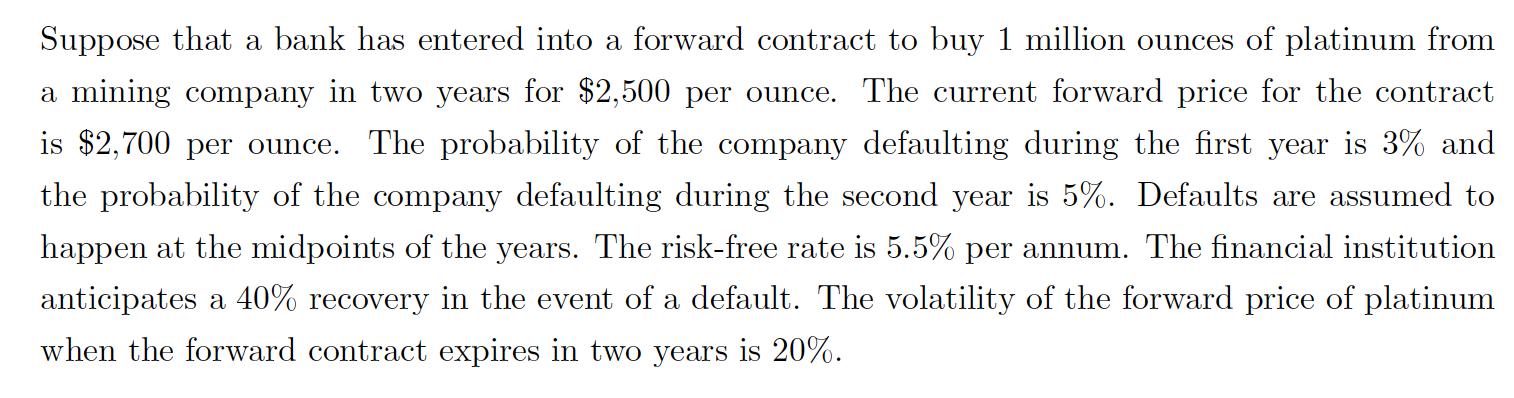
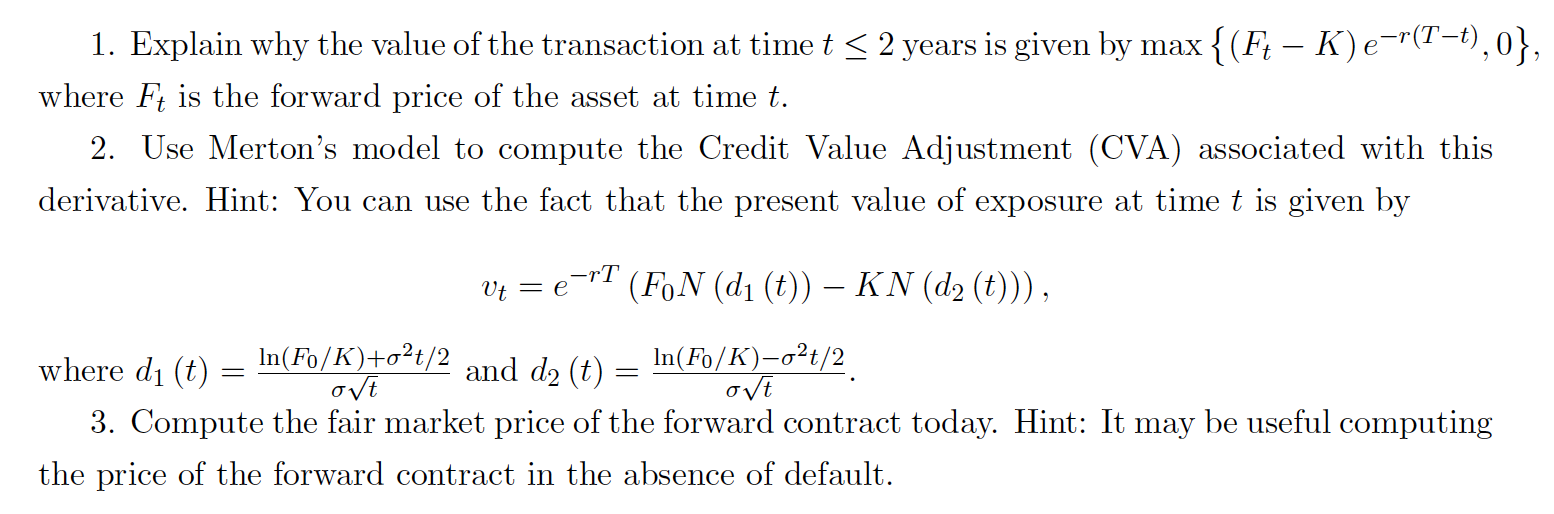
Suppose that a bank has entered into a forward contract to buy 1 million ounces of platinum from a mining company in two years for $2,500 per ounce. The current forward price for the contract is $2,700 per ounce. The probability of the company defaulting during the first year is 3% and the probability of the company defaulting during the second year is 5%. Defaults are assumed to happen at the midpoints of the years. The risk-free rate is 5.5% per annum. The financial institution anticipates a 40% recovery in the event of a default. The volatility of the forward price of platinum when the forward contract expires in two years is 20%. 1. Explain why the value of the transaction at time t2 years is given by max{(FtK)er(Tt),0}, where Ft is the forward price of the asset at time t. 2. Use Merton's model to compute the Credit Value Adjustment (CVA) associated with this derivative. Hint: You can use the fact that the present value of exposure at time t is given by vt=erT(F0N(d1(t))KN(d2(t))), where d1(t)=tln(F0/K)+2t/2 and d2(t)=tln(F0/K)2t/2. 3. Compute the fair market price of the forward contract today. Hint: It may be useful computing the price of the forward contract in the absence of default. Suppose that a bank has entered into a forward contract to buy 1 million ounces of platinum from a mining company in two years for $2,500 per ounce. The current forward price for the contract is $2,700 per ounce. The probability of the company defaulting during the first year is 3% and the probability of the company defaulting during the second year is 5%. Defaults are assumed to happen at the midpoints of the years. The risk-free rate is 5.5% per annum. The financial institution anticipates a 40% recovery in the event of a default. The volatility of the forward price of platinum when the forward contract expires in two years is 20%. 1. Explain why the value of the transaction at time t2 years is given by max{(FtK)er(Tt),0}, where Ft is the forward price of the asset at time t. 2. Use Merton's model to compute the Credit Value Adjustment (CVA) associated with this derivative. Hint: You can use the fact that the present value of exposure at time t is given by vt=erT(F0N(d1(t))KN(d2(t))), where d1(t)=tln(F0/K)+2t/2 and d2(t)=tln(F0/K)2t/2. 3. Compute the fair market price of the forward contract today. Hint: It may be useful computing the price of the forward contract in the absence of default