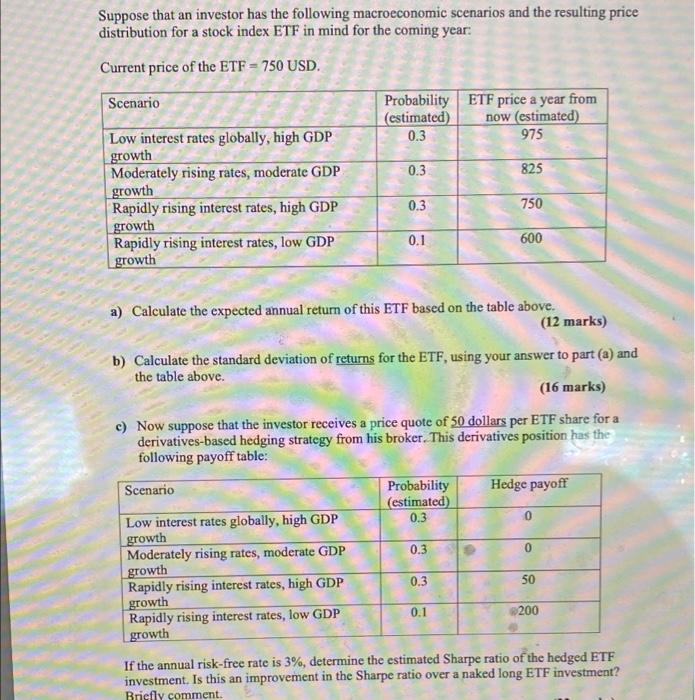
Suppose that an investor has the following macroeconomic scenarios and the resulting price distribution for a stock index ETF in mind for the coming year. Current price of the ETF = 750 USD. Scenario Probability ETF price a year from (estimated) now (estimated) Low interest rates globally, high GDP 0.3 975 growth Moderately rising rates, moderate GDP 0.3 825 growth Rapidly rising interest rates, high GDP 0.3 750 growth Rapidly rising interest rates, low GDP 0.1 600 growth a) Calculate the expected annual return of this ETF based on the table above. (12 marks) b) Calculate the standard deviation of returns for the ETF, using your answer to part (a) and the table above. (16 marks) c) Now suppose that the investor receives a price quote of 50 dollars per ETF share for a derivatives-based hedging strategy from his broker. This derivatives position has the following payoff table: Scenario Hedge payoff Probability (estimated) 0.3 0 0.3 0 Low interest rates globally, high GDP growth Moderately rising rates, moderate GDP growth Rapidly rising interest rates, high GDP growth Rapidly rising interest rates, low GDP growth 0.3 50 0.1 200 If the annual risk-free rate is 3%, determine the estimated Sharpe ratio of the hedged ETF investment. Is this an improvement in the Sharpe ratio over a naked long ETF investment? Briefly comment. Suppose that an investor has the following macroeconomic scenarios and the resulting price distribution for a stock index ETF in mind for the coming year. Current price of the ETF = 750 USD. Scenario Probability ETF price a year from (estimated) now (estimated) Low interest rates globally, high GDP 0.3 975 growth Moderately rising rates, moderate GDP 0.3 825 growth Rapidly rising interest rates, high GDP 0.3 750 growth Rapidly rising interest rates, low GDP 0.1 600 growth a) Calculate the expected annual return of this ETF based on the table above. (12 marks) b) Calculate the standard deviation of returns for the ETF, using your answer to part (a) and the table above. (16 marks) c) Now suppose that the investor receives a price quote of 50 dollars per ETF share for a derivatives-based hedging strategy from his broker. This derivatives position has the following payoff table: Scenario Hedge payoff Probability (estimated) 0.3 0 0.3 0 Low interest rates globally, high GDP growth Moderately rising rates, moderate GDP growth Rapidly rising interest rates, high GDP growth Rapidly rising interest rates, low GDP growth 0.3 50 0.1 200 If the annual risk-free rate is 3%, determine the estimated Sharpe ratio of the hedged ETF investment. Is this an improvement in the Sharpe ratio over a naked long ETF investment? Briefly comment