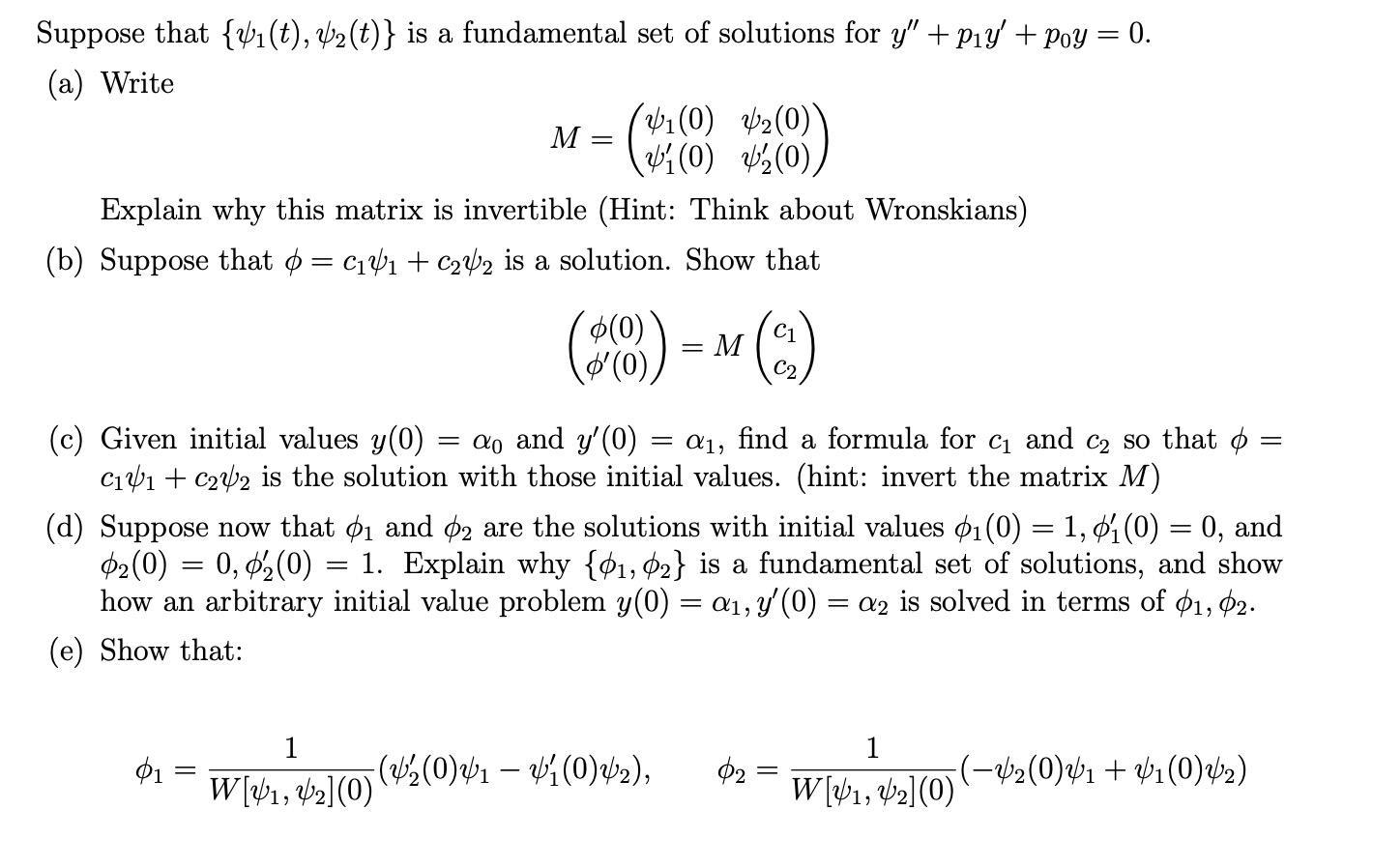
Suppose that {(t), (t)} is a fundamental set of solutions for y" + Py' + poy = 0. (a) Write $1 M Explain why this matrix is invertible (Hint: Think about Wronskians) (b) Suppose that = c1+ C22 is a solution. Show that 1 W[1,2] (0) = = (41 (0) (0) (0) (0), ao and y'(0) (c) Given initial values y(0) 1, find a formula for c and c so that = C1+ C22 is the solution with those initial values. (hint: invert the matrix M) (d) Suppose now that 1 and 2 are the solutions with initial values 1(0) = 1, (0) = 0, and 2(0) = 0, (0) = 1. Explain why {1,2} is a fundamental set of solutions, and show how an arbitrary initial value problem y(0) = a, y'(0) = a2 is solved in terms of 1, 02. (e) Show that: C1 (60) = M (G) = ((0)1 (0)2/2), $2 1 W[V1, V2](0) (42(0)41 + V1(0)42)