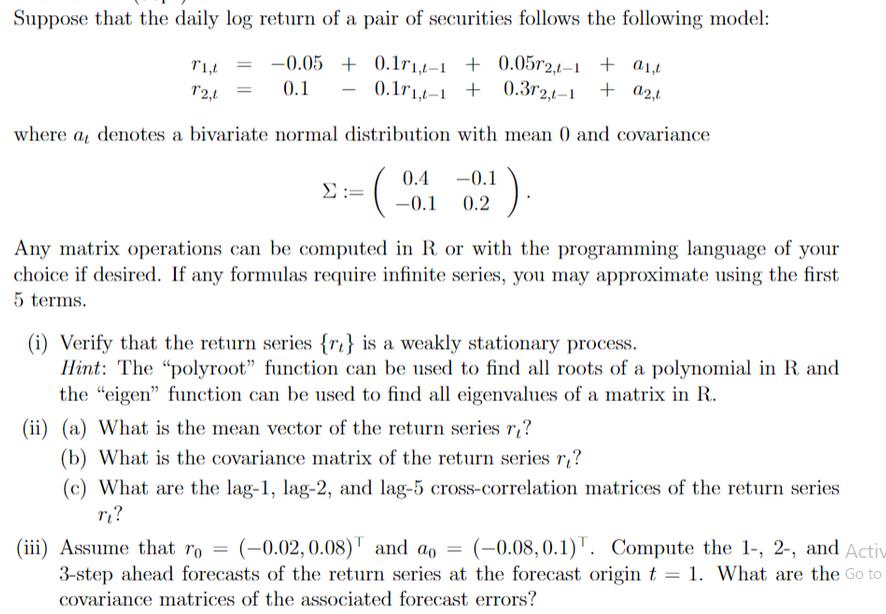
Suppose that the daily log return of a pair of securities follows the following model: T1,t = -0.05 T2,1 = 0.1 0.1r1,-1+0.05r2,1-1 0.171,-0.3r2,1-1 + a, + a2,1 where a, denotes a bivariate normal distribution with mean 0 and covariance ( ). := 0.4 -0.1 -0.1 0.2 Any matrix operations can be computed in R. or with the programming language of your choice if desired. If any formulas require infinite series, you may approximate using the first 5 terms. (i) Verify that the return series {r} is a weakly stationary process. Hint: The "polyroot" function can be used to find all roots of a polynomial in R. and the "eigen" function can be used to find all eigenvalues of a matrix in R. (ii) (a) What is the mean vector of the return series r? (b) What is the covariance matrix of the return series r? (c) What are the lag-1, lag-2, and lag-5 cross-correlation matrices of the return series ri? (iii) Assume that ro = (-0.02,0.08) and ao = (-0.08,0.1)T. Compute the 1-, 2-, and Activ 3-step ahead forecasts of the return series at the forecast origin t = 1. What are the Go to covariance matrices of the associated forecast errors?