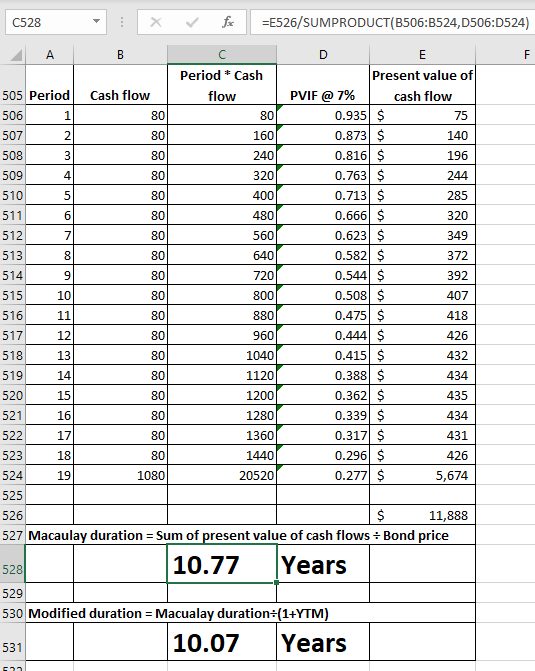
Suppose the yield to maturity on the bond in Problem 29 increases by .25 percent. What is the new price of the bond using duration? What is the new price of the bond using the bond pricing formula? What if the yield to maturity increases by 1 percent? By 2 percent? By 5 percent? What does this tell you about using duration to estimate bond price changes for large interest rate changes?

answer from 29 :

C528 : =E526/SUMPRODUCT(B506:3524,D506:0524) A B F Period * Cash flow 80 Cash flow 505 Period 506 1 80 507 2 80 160 508 3 80 240 509 4 80 320 510 5 80 400 480 511 6 80 7 80 560 512 513 514 80 6401 D E Present value of PVIF @ 7% cash flow 0.935 $ 75 0.873 $ 140 0.816 $ 196 0.763 $ 244 0.713 $ 285 0.666 $ 320 0.623 $ 349 0.582 $ 372 0.544 $ 392 0.508 $ 407 0.475 $ 418 0.444 $ 426 0.415 $ 432 0.388 $ 434 0.362 $ 435 0.339 $ 434 0.317 $ 431 0.296 $ 426 0.277 $ 5,674 9 80 720 10 80 800 515 516 11 80 880 12 80 960 517 518 13 80 14 80 519 520 1040 1120 1200 15 80 521 16 80 522 17 80 1280 1360 1440 20520 523 18 80 524 19 1080 525 526 $ 11,888 527 Macaulay duration = Sum of present value of cash flows + Bond price 5281 10.77 Years 529 530 Modified duration = Macualay duration:(1+YTM) 10.07 Years 531