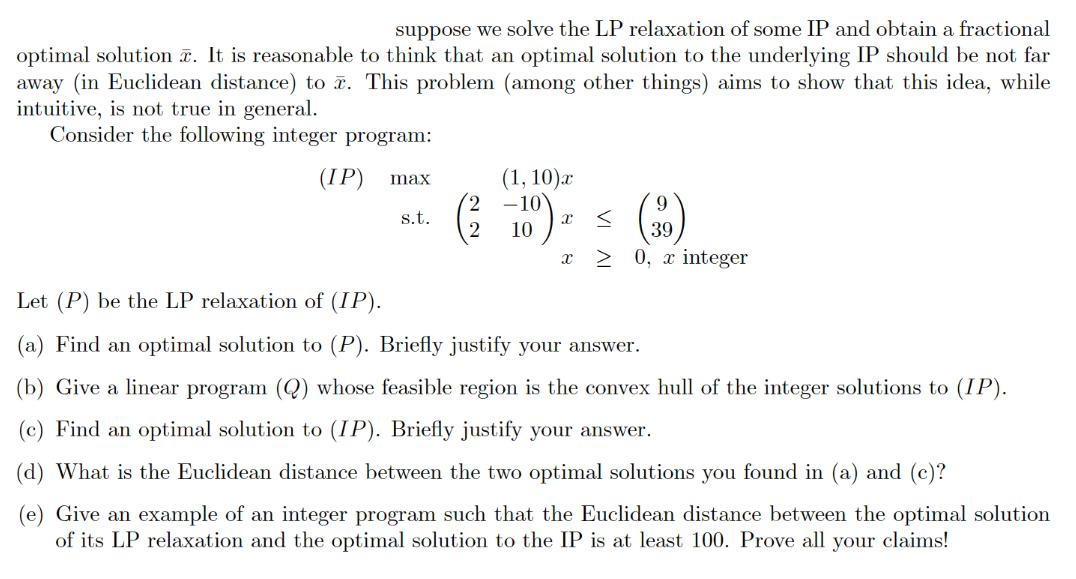
suppose we solve the LP relaxation of some IP and obtain a fractional optimal solution . It is reasonable to think that an optimal solution to the underlying IP should be not far away (in Euclidean distance) to . This problem (among other things) aims to show that this idea, while intuitive, is not true in general. Consider the following integer program: (IP) max (1, 10)x s.t. (2 100) * (39) x < x 0, x integer Let (P) be the LP relaxation of (IP). (a) Find an optimal solution to (P). Briefly justify your answer. (b) Give a linear program (Q) whose feasible region is the convex hull of the integer solutions to (IP). (c) Find an optimal solution to (IP). Briefly justify your answer. (d) What is the Euclidean distance between the two optimal solutions you found in (a) and (c)? (e) Give an example of an integer program such that the Euclidean distance between the optimal solution of its LP relaxation and the optimal solution to the IP is at least 100. Prove all your claims!