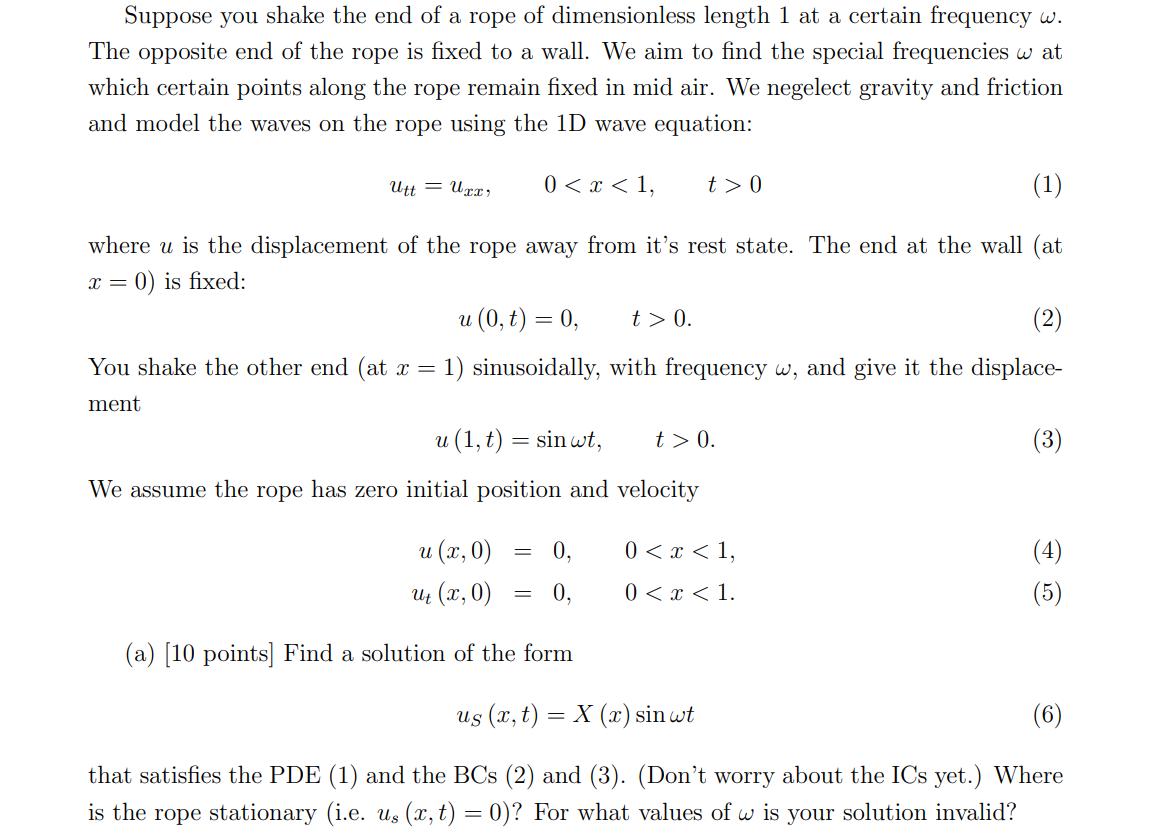
Suppose you shake the end of a rope of dimensionless length 1 at a certain frequency w. The opposite end of the rope is fixed to a wall. We aim to find the special frequencies wat which certain points along the rope remain fixed in mid air. We negelect gravity and friction and model the waves on the rope using the 1D wave equation: 0 < x < 1, t> 0 (1) where u is the displacement of the rope away from it's rest state. The end at the wall (at x = 0) is fixed: UttUxx, u (0, t) = 0, t> 0. (2) You shake the other end (at x = 1) sinusoidally, with frequency w, and give it the displace- ment u (1, t) = sin wt, We assume the rope has zero initial position and velocity u (x, 0) = 0, ut (x, 0) 0, (a) [10 points] Find a solution of the form = t> 0. 0 < x < 1, 0 < x < 1. (3) (4) (5) us (x, t) = X (x) sin wt (6) that satisfies the PDE (1) and the BCs (2) and (3). (Don't worry about the ICs yet.) Where is the rope stationary (i.e. us (x, t) = 0)? For what values of w is your solution invalid?