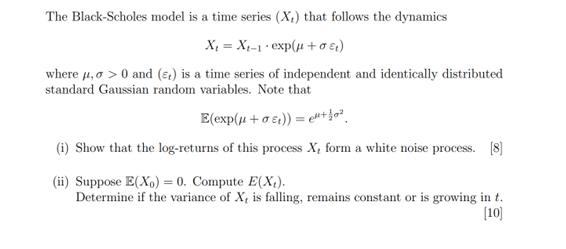
The Black-Scholes model is a time series (X) that follows the dynamics X =X-1 exp(+1) where ,0 and (e) is a time series of independent and identically distributed standard Gaussian random variables. Note that E(exp(+)) =+ f (i) Show that the log-returns of this process X, form a white noise process. [8] (ii) Suppose E(X) = 0. Compute E(X). Determine if the variance of X, is falling, remains constant or is growing in t. [10]