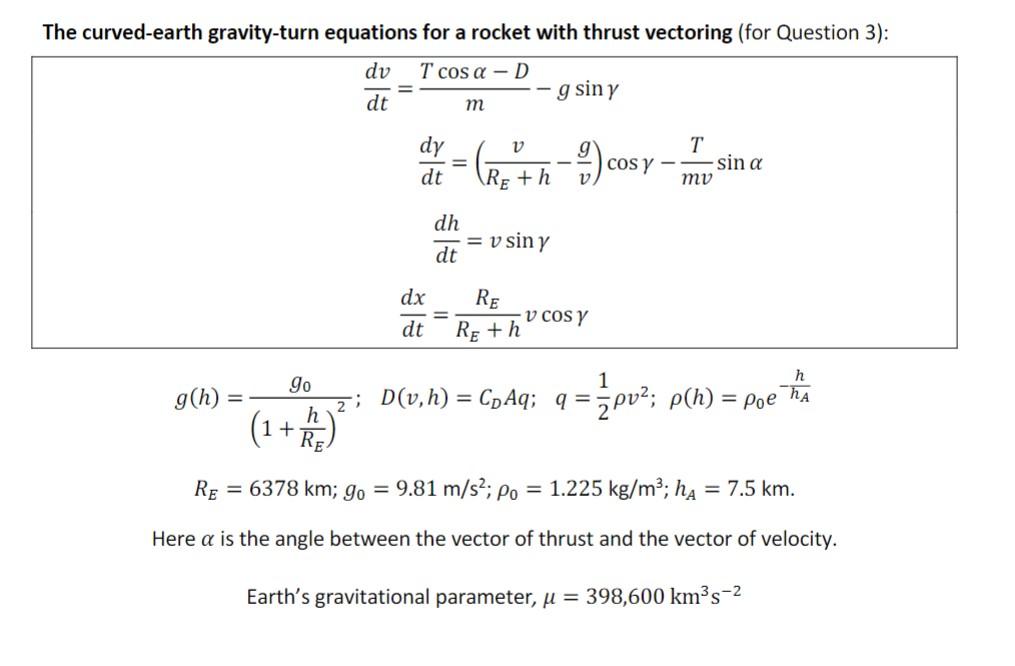
The curved-earth gravity-turn equations for a rocket with thrust vectoring (for Question 3): dtdv=mTcosDgsindtd=(RE+hvvg)cosmvTsindtdh=vsindtdx=RE+hREvcosg(h)=(1+REh)2g0;D(v,h)=CDAq;q=21v2;(h)=0ehAhRE=6378km;g0=9.81m/s2;0=1.225kg/m3;hA=7.5km. Here is the angle between the vector of thrust and the vector of velocity. Earth's gravitational parameter, =398,600km3s2 A single-stage rocket with gimbaled engine is to be launched on a gravity turn trajectory into a circular low Earth orbit; the rocket and mission have the following parameters: Motion of a gimbaled engine rocket can be described by the equations shown in page 1. Remind that angle should be expressed in radians. (a) Prepare a Matlab code for integration of the differential equations (shown on the first page) describing the gravity turn launch in the curved-Earth model with gimbaled engine. Account for the atmospheric drag, gravity variation with the altitude and thrust vectoring. To avoid division by zero consider a small finite initial velocity, say, 10cm/s. Consider the case when the angle between the vector of thrust and the vector of velocity depends on time and pitch-angle as (t,)={max(t/tb)200