The daily demand for a spare engine part is a random variable with a distribution based on past experience, given by

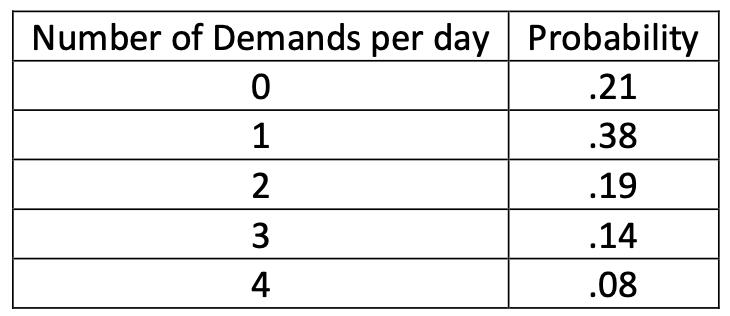
The part is expected to be obsolete after 400 days. Assume that demands from one day to the next are independent. The parts cost $1500 each when acquired in advance of the 400 day period and $5000 each when purchased on an emergency basis during the 400 day period. Holding costs for unused parts are based on a daily interest rate of 0.08%. How many parts should be acquired in advance of the 400 day period. ?

QUESTION 2:- Assume that weekly demand for computers at B&M Computer world is normally distributed, with a mean of 2,500 and a standard deviation of 500. The manufacturer takes 2 weeks to fill an order placed by the B&M manager. The store manager currently orders 10,000 computers when the inventory drops to 6,000.

a. What is the safety inventory carried by B&M

b. What is the average inventory carried by B&M

c. What is the average time spent by a computer at B&M

d. What is the Cycle Service Level for this replenishment policy

e. What is the Fill Rate for this replenishment policy

f. Suppose the company wants to increase its Cycle Service Level to 0.95, what should be the safety inventory

g. What should be the safety inventory if the desired fill rate is 97%.

QUESTION 3:- Weiss Paint store uses a (Q,r) inventory system to control its stock levels. For a particular popular white latex paint, historical data shows that the monthly demand is approximately normal with mean 28 and standard deviation of 8. Replenishment lead time for this paint is about 14 weeks. Each can of paint costs the store owner $6. Although excess demands are back ordered, store owner estimates that unfilled demands cost about $10 in bookkeeping and lost good will. Fixed costs of replenishment are $15 per order and holding costs are based on a 30% annual rate of interest.

a) What is the optimal lot sizes and reorder point for this brand of paint. ?

b) What is the optimal safety stock for this paint?

c) The owner decides that his stockout cost of $10 may not be accurate and decides to switch to a service level model, and decides to set his order quantity using the EOQ model, and determine the reorder point so that there is stock out in 90% of the order cycles. What is the resulting (Q,r) values. ? What is the fill rate that he would achieve using this policy.

d) Suppose he desires a fill rate of 95% what is the optimum (Q,r).
 

Transcribed Image Text:

Number of Demands per day Probability 0 1 23 .21 .38 .19 .14 4 .08 Number of Demands per day Probability 0 1 23 .21 .38 .19 .14 4 .08

Answer rating: 100% (QA)

Determining how many parts should be acquired the concept of theory of Economic Order Quantity EOQ w... View the full answer