Question: The feasible set of a linear programming problem and a mixed-integer problem is given in Figure 1. The feasible region depicts the area within

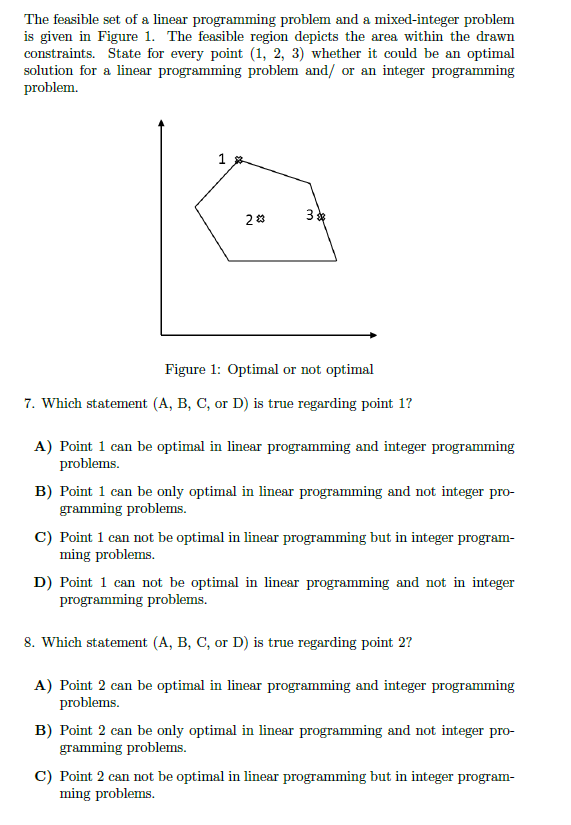
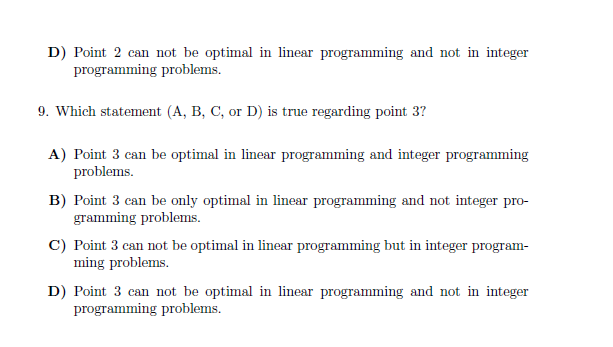
The feasible set of a linear programming problem and a mixed-integer problem is given in Figure 1. The feasible region depicts the area within the drawn constraints. State for every point (1, 2, 3) whether it could be an optimal solution for a linear programming problem and/ or an integer programming problem. 23 3* m Figure 1: Optimal or not optimal 7. Which statement (A, B, C, or D) is true regarding point 1? A) Point 1 can be optimal in linear programming and integer programming problems. B) Point 1 can be only optimal in linear programming and not integer pro- gramming problems. C) Point 1 can not be optimal in linear programming but in integer program- ming problems. D) Point 1 can not be optimal in linear programming and not in integer programming problems. 8. Which statement (A, B, C, or D) is true regarding point 2? A) Point 2 can be optimal in linear programming and integer programming problems. B) Point 2 can be only optimal in linear programming and not integer pro- gramming problems. C) Point 2 can not be optimal in linear programming but in integer program- ming problems. D) Point 2 can not be optimal in linear programming and not in integer programming problems. 9. Which statement (A, B, C, or D) is true regarding point 37 A) Point 3 can be optimal in linear programming and integer programming problems. B) Point 3 can be only optimal in linear programming and not integer pro- gramming problems. C) Point 3 can not be optimal in linear programming but in integer program- ming problems. D) Point 3 can not be optimal in linear programming and not in integer programming problems. The feasible set of a linear programming problem and a mixed-integer problem is given in Figure 1. The feasible region depicts the area within the drawn constraints. State for every point (1, 2, 3) whether it could be an optimal solution for a linear programming problem and/ or an integer programming problem. 23 3* m Figure 1: Optimal or not optimal 7. Which statement (A, B, C, or D) is true regarding point 1? A) Point 1 can be optimal in linear programming and integer programming problems. B) Point 1 can be only optimal in linear programming and not integer pro- gramming problems. C) Point 1 can not be optimal in linear programming but in integer program- ming problems. D) Point 1 can not be optimal in linear programming and not in integer programming problems. 8. Which statement (A, B, C, or D) is true regarding point 2? A) Point 2 can be optimal in linear programming and integer programming problems. B) Point 2 can be only optimal in linear programming and not integer pro- gramming problems. C) Point 2 can not be optimal in linear programming but in integer program- ming problems. D) Point 2 can not be optimal in linear programming and not in integer programming problems. 9. Which statement (A, B, C, or D) is true regarding point 37 A) Point 3 can be optimal in linear programming and integer programming problems. B) Point 3 can be only optimal in linear programming and not integer pro- gramming problems. C) Point 3 can not be optimal in linear programming but in integer program- ming problems. D) Point 3 can not be optimal in linear programming and not in integer programming problems.

Step by Step Solution

★★★★★

3.41 Rating (157 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The skin friction coefficient Cf for a laminar boundary ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Programming Questions!

An asset was acquired on September 3 0 , 2 0 2 4 for $ 1 1 8 , 0 0 0 with an estimated five - year life and $ 3 0 , 0 0 0 residual life. The company uses double declining balance depreciation. What...

Planning is one of the most important management functions in any business. A front office managers first step in planning should involve determine the departments goals. Planning also includes...

Let A, B be sets. Define: (a) the Cartesian product (A B) (b) the set of relations R between A and B (c) the identity relation A on the set A [3 marks] Suppose S, T are relations between A and B, and...

How does unemployment behave over the business cycle?

How could Mattel have allowed these problems to happen? Aside from simply not using Chinese suppliers, could Mattel have avoided the toy quality/safety problems to begin with? Explain your answers.

Suppose that each of two statisticians A and B must estimate a certain parameter p whose value is unknown (0 Answered: 1 week ago

For a dummy variable multiple regression model based on the dummy variables ????1 and ????2 and a single quantitative explanatory variable ???? (a) write down the equation of the interaction model....

Lockheed, one of the largest defense contractors in the United States, reported EBITDA of $1,290 million in a recent financial year, prior to interest expenses of $215 million and depreciation...

A cash equivalent is: Options: An investment readily convertible to a known amount of cash. Close to its maturity date but its market value may still be affected by interest rate changes. Generally...

The following unadjusted trial balance is for Power Demolition Company as of the end of its April 30, 2013, fiscal year. The April 30, 2012, credit balance of the owners capital account was $46,900,...

4 Consider each of the following independent situations: Required: For each situation, calculate the missing amount. 1 . 5 points e B o o k

1.) Explain what is meant by oppressive conduct. 2.) Discuss any two (2) remedies for oppression under s 233 of the Corporations Act 2001 3. What obligations do financial service providers have in...

1. What is the jury foreman? What is their responsibility? 2. How would you explain reasonable and probable grounds? 3. Who is not eligible to sit on a jury? What do you feel is the reason for this?...

1. A detector is placed on a screen onto which a bright red spot is observed. In calculation of the numerical aperture of the fiber, the diameter of the bright spot (W) and the distance of the...

1. What is revealed about human nature (from Genesis 1-2)? 2. What are the consequences of the fall for human nature (from Genesis 3)? 3. What is revealed about human purpose? What does it mean for...

When a 0.051M aqueous solution of a certain acid is prepared, the acid is 2.7% dissociated. Calculate the acid dissociation constant Ka of the acid. Round your answer to 2 significant digits.

What excites you most about working in IT? What hurdles or challenges stand in the way? What do you want to learn more about?

14. In testing the existence assertion, an auditor ordinarily works from the a. Financial statements to the accounting records. b. General journal to the general ledger. c. Supporting evidence to the...

Consider the model with nonpreemptive priorities presented in Sec. 17.8. Suppose there are two priority classes, with 1 = 2 and 2 = 3. In designing this queueing system, you are offered the choice...

A certain large shop doing light fabrication work uses a single central storage facility (dispatch station) for material in process storage. The typical procedure is that each employee personally...

For each of the following models, write the balance equations and show that they are satisfied by the solution given in Sec. 17.6 for the steady-state distribution of the number of customers in the...

Suppose the parameter of interest is =h (t), where h (t) =at2 + bt + c and t is the population total. Let =h (). Show, in a stratified random sample with two observations per stratum, that if Then...

Variance estimation with post stratification. Suppose we post stratify the sample into L post strata, with population counts N1, N2. . . NL. Then the post stratified estimator for the population...

Suppose a stratified random sample is taken with two observations per stratum. Show that if Then First note that Then express BRR (str) directly using yh1 and yh2.