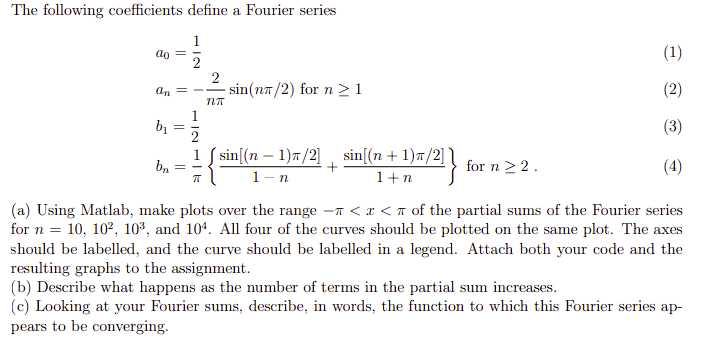
The following coefficients define a Fourier series ao = b an == || HI2 bn = 1 2 - sin(n/2) for n1 NA sin[(n 1)/2] 1-n {sin((1 + sin[(n+1)/2]] 1+n /]} for n 2. (1) (2) (3) (4) (a) Using Matlab, make plots over the range - < x < of the partial sums of the Fourier series for n = = 10, 10, 10, and 104. All four of the curves should be plotted on the same plot. The axes should be labelled, and the curve should be labelled in a legend. Attach both your code and the resulting graphs to the assignment. (b) Describe what happens as the number of terms in the partial sum increases. (c) Looking at your Fourier sums, describe, in words, the function to which this Fourier series ap- pears to be converging.