Question
The following model was applied to manage a coastal fishery: Growth function: = , , thousand tons per year where x is effort, measured in
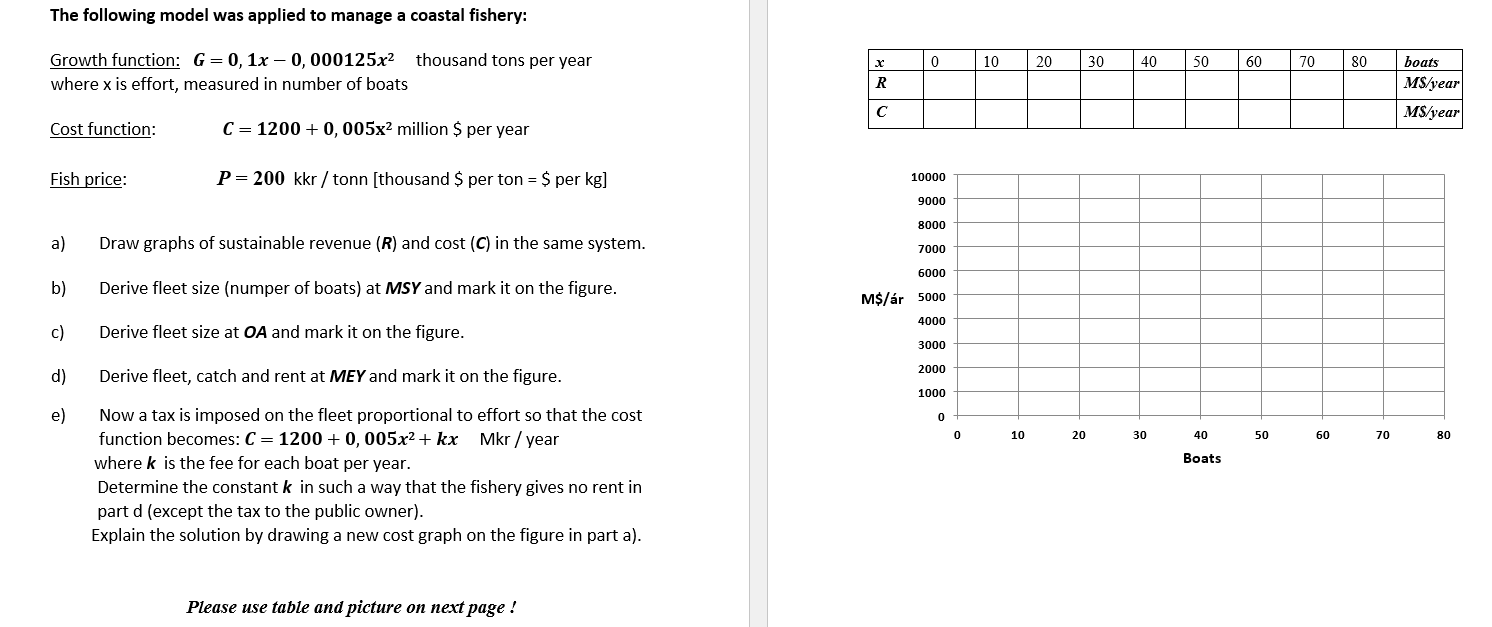
The following model was applied to manage a coastal fishery:
Growth function: = , , thousand tons per year where x is effort, measured in number of boats
Cost function: = + , million $ per year
Fish price: P = 200 k$ / tonn [thousand $ per ton = $ per kg]
- Write function for sustainable revenue (R) and cost (C)
- Derive fleet size (numper of boats) at MSY and mark it on the figure.
- Derive fleet size at OA
- Derive fleet, catch and rent at MEY
- Now a tax is imposed on the fleet proportional to effort so that the cost function becomes: = + , + M$ / year
where k is the fee for each boat per year.
Determine the constant k in such a way that the fishery gives no rent in part d (except the tax to the public owner).
Don't need more info, it's ok i you can't solve this. you can just at least give me refund :)
OA is open access method is fishery economics, and the figure is something each one has to make.
this is the whole example. It's ok to refund
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


