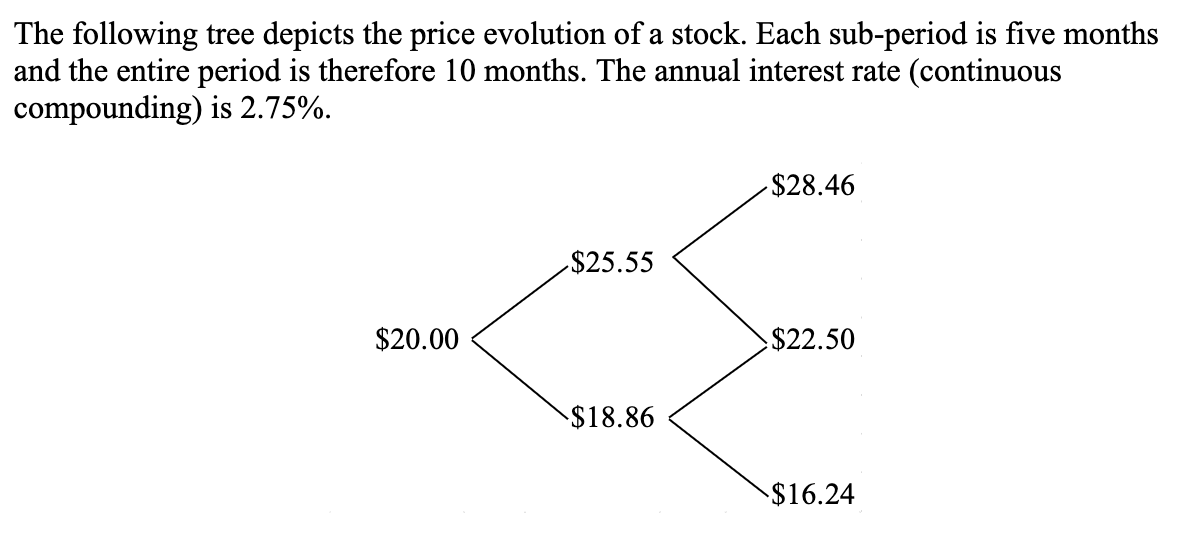
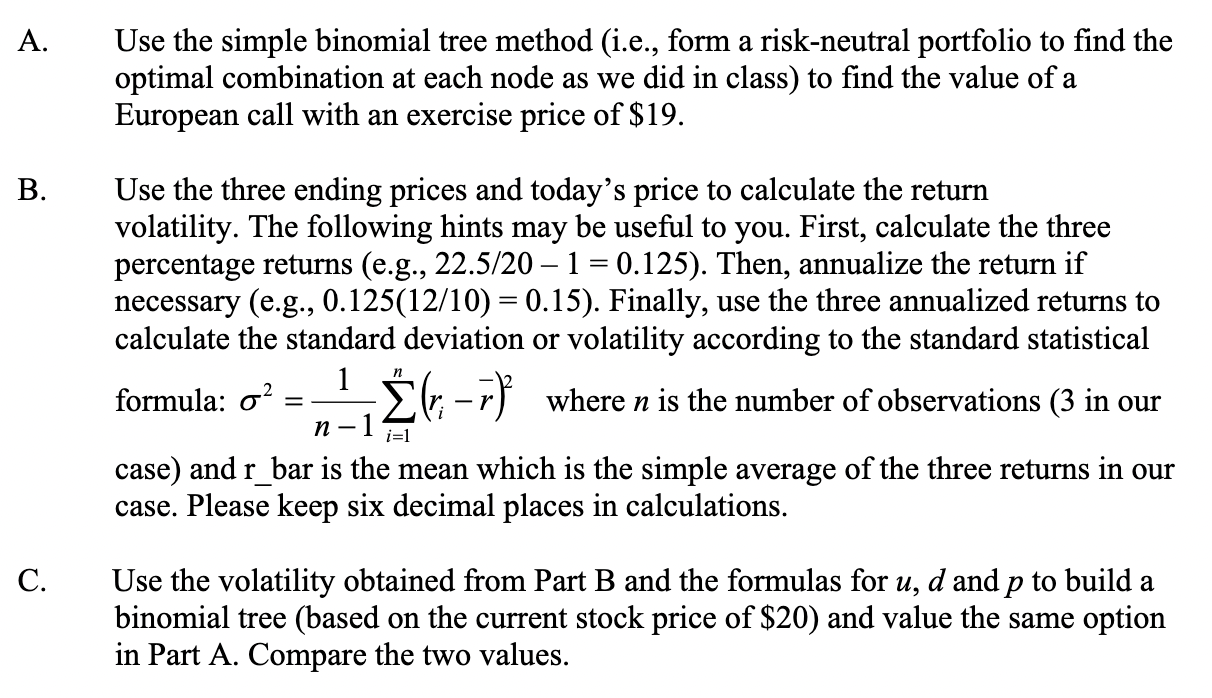
The following tree depicts the price evolution of a stock. Each sub-period is five months and the entire period is therefore 10 months. The annual interest rate (continuous compounding) is 2.75%. $28.46 $25.55 $20.00 $22.50 $18.86 $16.24 A. Use the simple binomial tree method (i.e., form a risk-neutral portfolio to find the optimal combination at each node as we did in class) to find the value of a European call with an exercise price of $19. B. Use the three ending prices and today's price to calculate the return volatility. The following hints may be useful to you. First, calculate the three percentage returns (e.g., 22.5/20 1 = 0.125). Then, annualize the return if necessary (e.g., 0.125(12/10) = 0.15). Finally, use the three annualized returns to calculate the standard deviation or volatility according to the standard statistical 1 formula: o? 3 6: -7} where n is the number of observations (3 in our 1 case) and r_bar is the mean which is the simple average of the three returns in our case. Please keep six decimal places in calculations. n- i=1 C. Use the volatility obtained from Part B and the formulas for u, d and p to build a binomial tree (based on the current stock price of $20) and value the same option in Part A. Compare the two values. The following tree depicts the price evolution of a stock. Each sub-period is five months and the entire period is therefore 10 months. The annual interest rate (continuous compounding) is 2.75%. $28.46 $25.55 $20.00 $22.50 $18.86 $16.24 A. Use the simple binomial tree method (i.e., form a risk-neutral portfolio to find the optimal combination at each node as we did in class) to find the value of a European call with an exercise price of $19. B. Use the three ending prices and today's price to calculate the return volatility. The following hints may be useful to you. First, calculate the three percentage returns (e.g., 22.5/20 1 = 0.125). Then, annualize the return if necessary (e.g., 0.125(12/10) = 0.15). Finally, use the three annualized returns to calculate the standard deviation or volatility according to the standard statistical 1 formula: o? 3 6: -7} where n is the number of observations (3 in our 1 case) and r_bar is the mean which is the simple average of the three returns in our case. Please keep six decimal places in calculations. n- i=1 C. Use the volatility obtained from Part B and the formulas for u, d and p to build a binomial tree (based on the current stock price of $20) and value the same option in Part A. Compare the two values








