Question
The goal of this problem is to show that any n n matrix A is similar to a triangular matrix (here we allow entires to
The goal of this problem is to show that any n n matrix A is similar to a triangular matrix (here we allow entires to be complex numbers). The proof is by induction on n.
a) Show the statement for n = 1
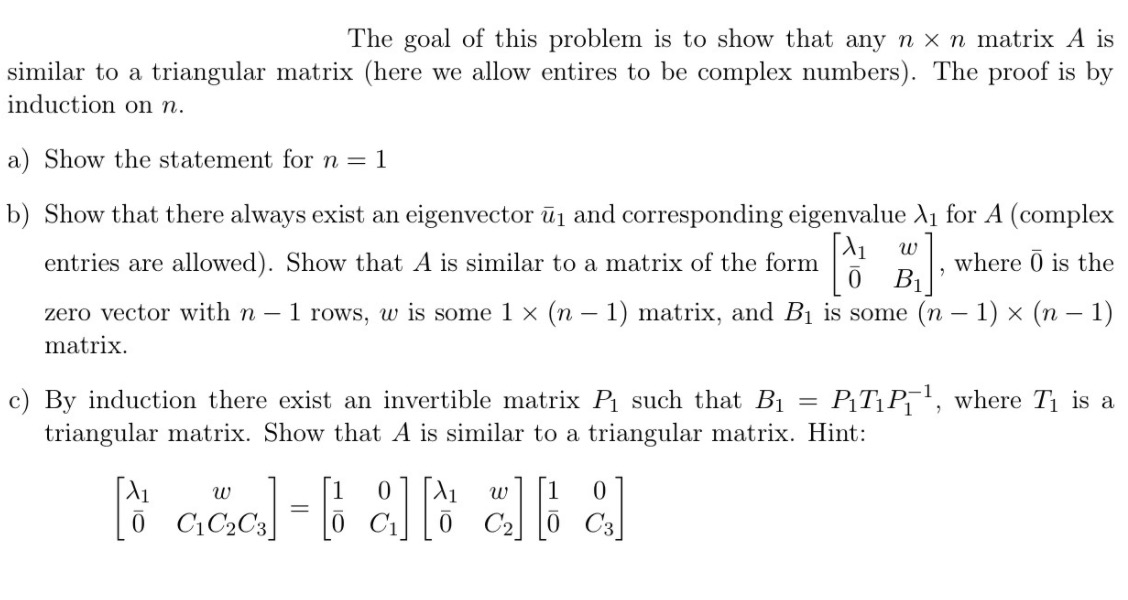
b) Show that there always exist an eigenvector u1 and corresponding eigenvalue 1 for A (complex entries are allowed).
Show that A is similar to a matrix of the form 1 w 0 B1 , where 0 is the zero vector with n 1 rows, w is some 1 (n 1) matrix, and B1 is some (n 1) (n 1) matrix.
c) By induction there exist an invertible matrix P1 such that B1 = P1T1P 1 1 , where T1 is a triangular matrix.
Show that A is similar to a triangular matrix. Hint: 1 w 0 C1C2C3 = 1 0 0 C1 1 w 0 C2 1 0 0 C3
photo attached https://imgur.com/a/JKCBMjj
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


