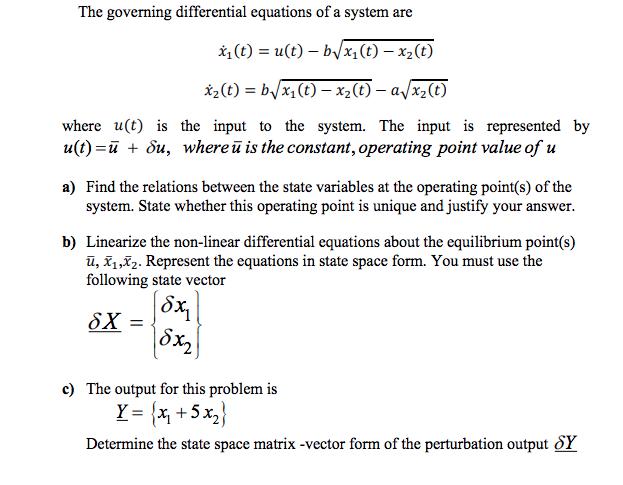
The governing differential equations of a system are x(t) = u(t) bx(t) x(t) x2(t) = bx(t) = x2(t) - ax2(t) where u(t) is the input to the system. The input is represented by u(t) = + Su, where u is the constant, operating point value of u a) Find the relations between the state variables at the operating point(s) of the system. State whether this operating point is unique and justify your answer. b) Linearize the non-linear differential equations about the equilibrium point(s) u, x1,x2. Represent the equations in state space form. You must use the following state vector 8X = Ex 8x2 c) The output for this problem is Y = {x+5x2} Determine the state space matrix -vector form of the perturbation output SY