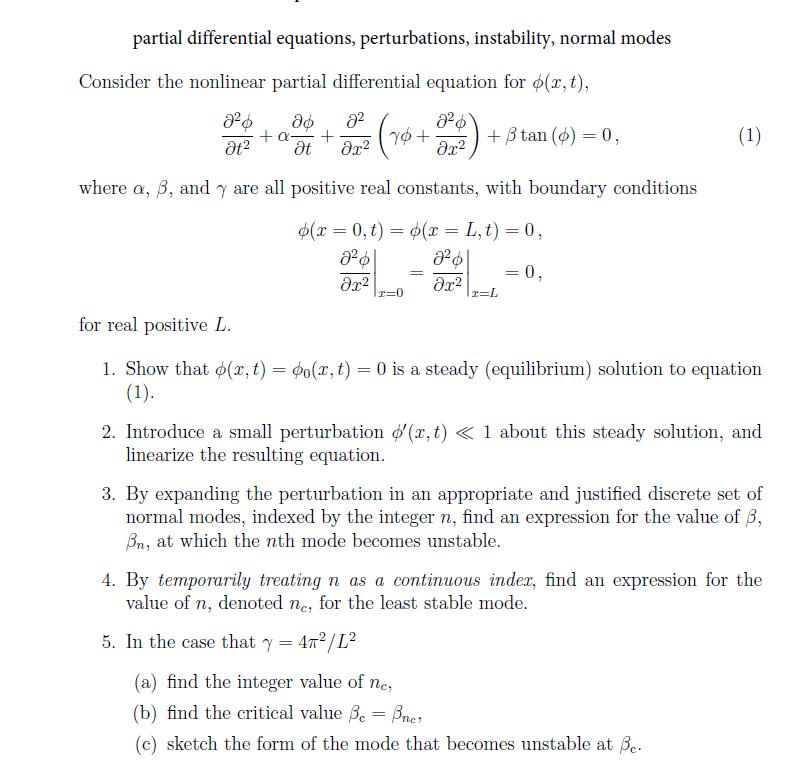
partial differential equations, perturbations, instability, normal modes Consider the nonlinear partial differential equation for o(x,t), t t 2 82 +a. + a 70+ 2 +tan (6) = 0, == where a, B, and y are all positive real constants, with boundary conditions (x = 0,t) = (x = L,t) = 0, | 2 x=0 == 826 2 = 0, |x=L for real positive L. (1) 1. Show that o(x,t) = oo(x,t) = 0 is a steady (equilibrium) solution to equation (1). 2. Introduce a small perturbation o'(x, t) < 1 about this steady solution, and linearize the resulting equation. 3. By expanding the perturbation in an appropriate and justified discrete set of normal modes, indexed by the integer n, find an expression for the value of , Bn, at which the nth mode becomes unstable. 4. By temporarily treating n as a continuous index, find an expression for the value of n, denoted ne, for the least stable mode. 5. In the case that y = 4/L (a) find the integer value of ne, (b) find the critical value e = ne (c) sketch the form of the mode that becomes unstable at Bc.