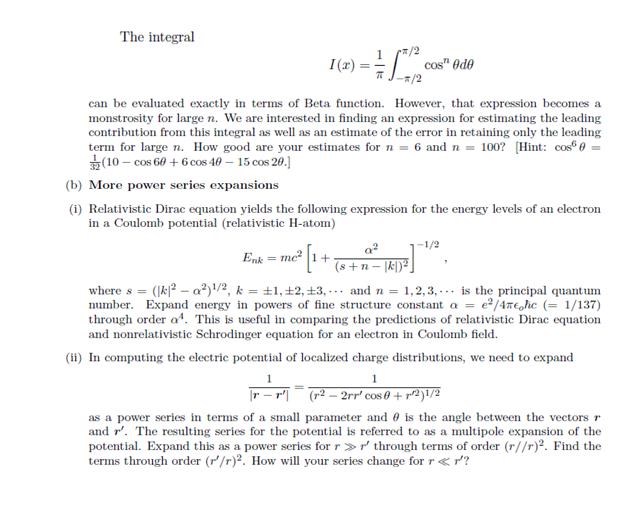
The integral I(x)= == can be evaluated exactly in terms of Beta function. However, that expression becomes a monstrosity for large n. We are interested in finding an expression for estimating the leading contribution from this integral as well as an estimate of the error in retaining only the leading term for large n. How good are your estimates for n = 6 and n = 100? [Hint: cos (10-cos 60+ 6 cos 40 - 15 cos 20.] (b) More power series expansions (i) Relativistic Dirac equation yields the following expression for the energy levels of an electron in a Coulomb potential (relativistic H-atom) Enk 2 [1+ = mc a (s+n-k) cos" ode -1/2 where s= (|k|a)/2, k = 1, 12, 13, 8 = and n = 1,2,3, is the principal quantum number. Expand energy in powers of fine structure constant a e/4me,hic ( 1/137) through order a. This is useful in comparing the predictions of relativistic Dirac equation and nonrelativistic Schrodinger equation for an electron in Coulomb field. (ii) In computing the electric potential of localized charge distributions, we need to expand 1 -r' (r2-2rr' cos@+2)1/2 as a power series in terms of a small parameter and is the angle between the vectors r and r'. The resulting series for the potential is referred to as a multipole expansion of the potential. Expand this as a power series for r>r' through terms of order (r//r)2. Find the terms through order (r/r)2. How will your series change for r