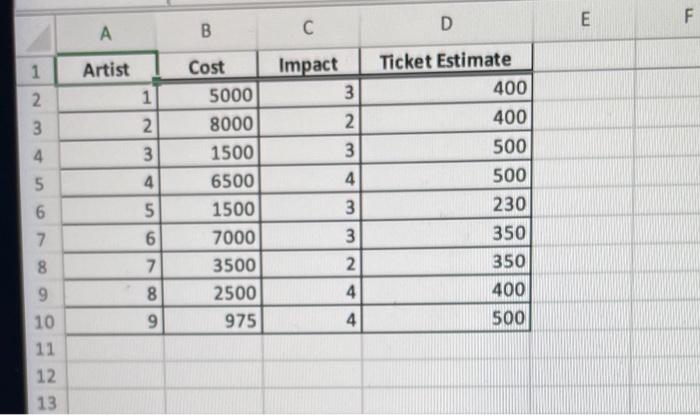
The Kelmer Performing Arts Center offers a series of four programs from a list that includes jazz, bluegrass, folk, classical, and comedy. The Program Coordinator needs to determine which acts to choose for next year's series. She assigned an "impact" rating to each artist that reflects how well the act meets the center's mission and provides community value. This rating is on a scale from 1 to 4 , with 4 being the greatest impact and 1 being the least impact. The theater has 500 seats with an average ticket price of $20. Based on an estimate of the potential sales, the revenue from each artist is calculated. The center has a budget of $20,000 and would like the total impact factor to be at least 12, reflecting an average impact per artist of at least 3 . To avoid duplication of genres, at most one of artists 4,8 , and 9 may be chosen, and at most one of artists 3 and 5 may be chosen. Finally, the center wishes to maximize its revenue. Data are in the accompanying table. Which combination of artists maximizes revenue within the constraints? Select all that apply. A. 3 B. 4 C. 1 D. 9 E. 5 F. 2 G. 6 H. 8 \begin{tabular}{|c|r|r|r|r|} \hline & \multicolumn{1}{|c|}{ A } & \multicolumn{1}{c|}{ B } & \multicolumn{1}{c|}{ D } \\ \hline 1 & Artist & \multicolumn{1}{c|}{ Cost } & \multicolumn{1}{c|}{ Impact } & Ticket Estimate \\ \hline 2 & 1 & 5000 & 3 & 400 \\ \hline 3 & 2 & 8000 & 2 & 400 \\ \hline 4 & 3 & 1500 & 3 & 500 \\ \hline 5 & 4 & 6500 & 4 & 500 \\ \hline 6 & 5 & 1500 & 3 & 230 \\ \hline 7 & 6 & 7000 & 3 & 350 \\ \hline 8 & 7 & 3500 & 2 & 350 \\ \hline 9 & 8 & 2500 & 4 & 400 \\ \hline 10 & 9 & 975 & 4 & 500 \\ \hline \end{tabular} The Kelmer Performing Arts Center offers a series of four programs from a list that includes jazz, bluegrass, folk, classical, and comedy. The Program Coordinator needs to determine which acts to choose for next year's series. She assigned an "impact" rating to each artist that reflects how well the act meets the center's mission and provides community value. This rating is on a scale from 1 to 4 , with 4 being the greatest impact and 1 being the least impact. The theater has 500 seats with an average ticket price of $20. Based on an estimate of the potential sales, the revenue from each artist is calculated. The center has a budget of $20,000 and would like the total impact factor to be at least 12, reflecting an average impact per artist of at least 3 . To avoid duplication of genres, at most one of artists 4,8 , and 9 may be chosen, and at most one of artists 3 and 5 may be chosen. Finally, the center wishes to maximize its revenue. Data are in the accompanying table. Which combination of artists maximizes revenue within the constraints? Select all that apply. A. 3 B. 4 C. 1 D. 9 E. 5 F. 2 G. 6 H. 8 \begin{tabular}{|c|r|r|r|r|} \hline & \multicolumn{1}{|c|}{ A } & \multicolumn{1}{c|}{ B } & \multicolumn{1}{c|}{ D } \\ \hline 1 & Artist & \multicolumn{1}{c|}{ Cost } & \multicolumn{1}{c|}{ Impact } & Ticket Estimate \\ \hline 2 & 1 & 5000 & 3 & 400 \\ \hline 3 & 2 & 8000 & 2 & 400 \\ \hline 4 & 3 & 1500 & 3 & 500 \\ \hline 5 & 4 & 6500 & 4 & 500 \\ \hline 6 & 5 & 1500 & 3 & 230 \\ \hline 7 & 6 & 7000 & 3 & 350 \\ \hline 8 & 7 & 3500 & 2 & 350 \\ \hline 9 & 8 & 2500 & 4 & 400 \\ \hline 10 & 9 & 975 & 4 & 500 \\ \hline \end{tabular}