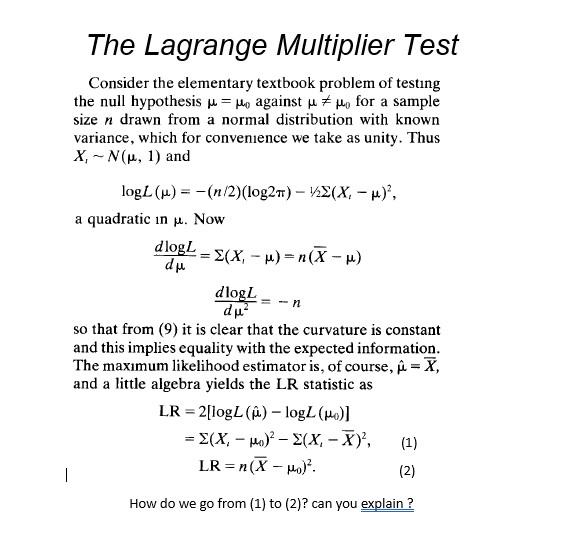
The Lagrange Multiplier Test Consider the elementary textbook problem of testing the null hypothesis = against for a sample size n drawn from a normal distribution with known variance, which for convenience we take as unity. Thus X, N(, 1) and logL (u) (n/2)(log2T) - (X-), a quadratic in . Now dlogL d (X-) n(X-) dlogL d = = n so that from (9) it is clear that the curvature is constant and this implies equality with the expected information. The maximum likelihood estimator is, of course, = X, and a little algebra yields the LR statistic as LR 2[logL(A) - log (po)] = E(X)-(X,-X), LR=n(X-Mo). (1) (2) How do we go from (1) to (2)? can you explain?