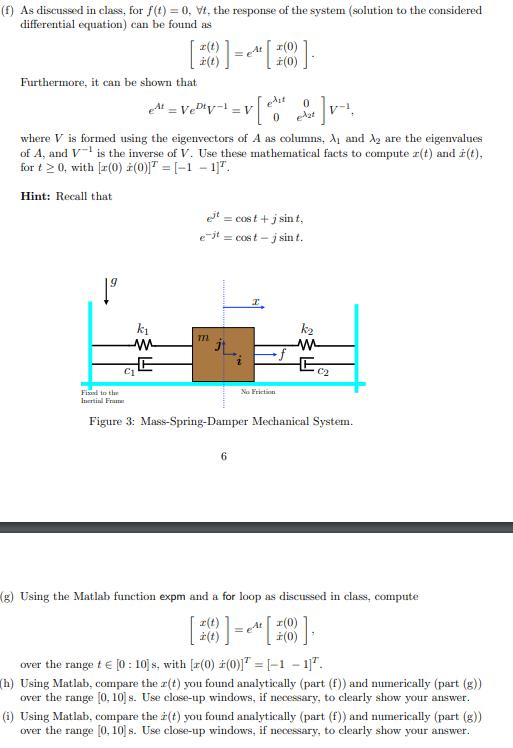
The mechanical system shown in Fig. 3 is composed of a block with mass m, two linear springs with constants ki and k2, and two linear dampers with constants c and c. The block can slide freely (without friction) on the supporting horizontal platform; as seen, the spring and damper with constants k and c connect the block with the left wall; and, as seen, the spring and damper with constants k2 and c2 connect the block with the right wall. The two walls and the supporting surface are fixed with respect to the inertial frame with vector axes i and j. Here, for convenience, the distance r(t) is defined to the right from the origin of the inertial frame. Also, an instantaneous external horizontal force with magnitude f(t) is exerted on the block, as shown. (a) Identify and list all the forces exerted on the block at the instant t, assuming that the block is moving toward the right (i.e., (t) > 0) and is on the right side (i.e.. x(t) > 0). (b) Using your answer for (a), draw the free-body diagram of the system at the instant t assuming that the block is moving toward the right (i.e., i(t) > 0) and is on the right side (i.e., r(t) > 0). (c) Using Newton's second law, write the two equations (vertical and horizontal) that describe the instantaneous kinetics of the system at the instant t, assuming that the block is moving toward the right (i.e., (t) > 0) and is on the right side (i.e., r(t) > 0). (d) Using your answer for (c), show that the entire dynamics of the system can be described with a differential equation of the form [ (6)] = A[2(6)] + Bf (t), i(t) (t) 1 kg, where A is a 2 x 2 real matrix (A E R2x2) and B is a 2 x 1 real matrix (B Rx1). (e) Furthermore, show that for ki = k2 = 1.5 N/m, c = C = 0kg/s, and m= 0 = - [ ] -3 0 B-[8]. and B= (f) As discussed in class, for f(t) = 0, Vt, the response of the system (solution to the considered differential equation) can be found as Furthermore, it can be shown that Hint: Recall that 1 [0]=[] At (0) (t) where V is formed using the eigenvectors of A as columns, A and A2 are the eigenvalues of A, and V is the inverse of V. Use these mathematical facts to compute z(t) and i(t), for t 0, with [z(0) (0)] = [-1-1]. et - Vety-1 = V k 0 v- = v[ edit ]v- 0 3 evit = cost+j sint, e-it=cost-jsin t. m No Friction 6 Find to the Inertial Fram Figure 3: Mass-Spring-Damper Mechanical System. k M = et E C2 (g) Using the Matlab function expm and a for loop as discussed in class, compute z(t) i(t) r(0) * (0) over the range t [0:10]s, with [r(0) (0)] = [-1-1]. (h) Using Matlab, compare the r(t) you found analytically (part (f)) and numerically (part (g)) over the range [0, 10] s. Use close-up windows, if necessary, to clearly show your answer. (i) Using Matlab, compare the i(t) you found analytically (part (f)) and numerically (part (g)) over the range [0, 10] s. Use close-up windows, if necessary, to clearly show your answer.