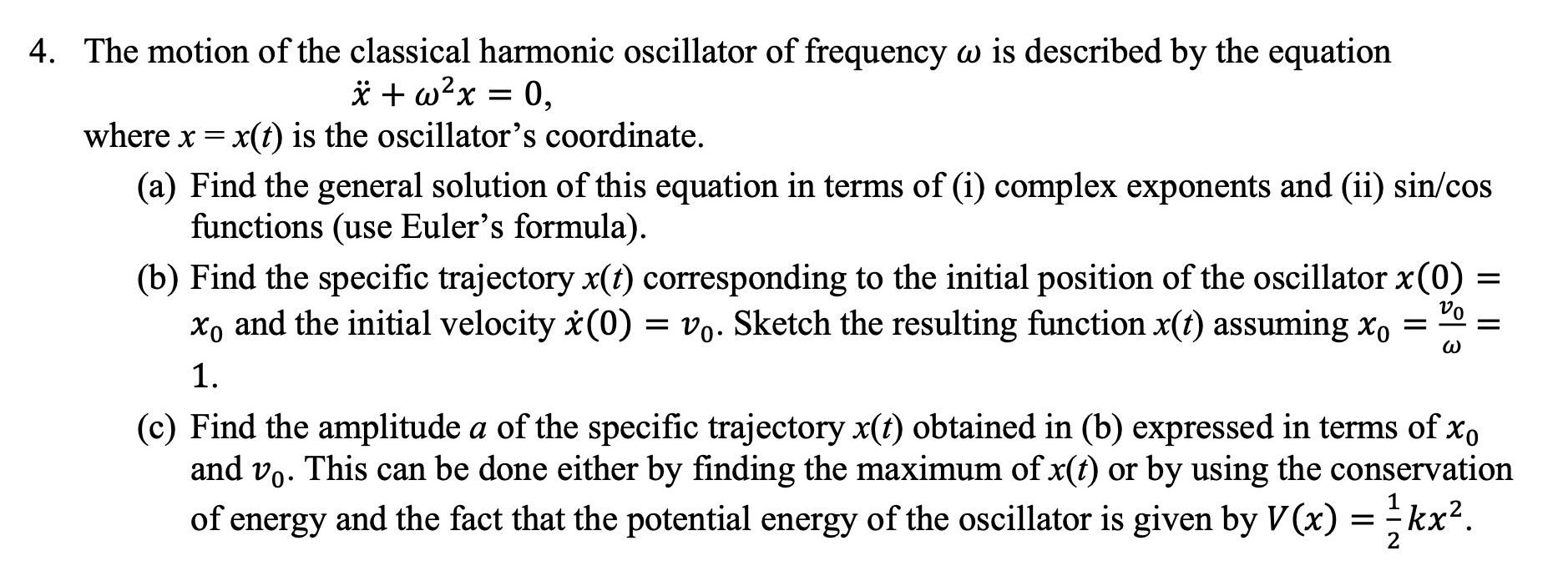
The motion of the classical harmonic oscillator of frequency $$ is described by the equation

$x^{}+{}^{2}x=0,$

where $x=x(t)$ is the oscillator's coordinate.

$($a$)$ Find the general solution of this equation in terms of $($i$)$ complex exponents and $($ii$)s\frac{in}{cos}$

functions $($use Euler's formula$).$

$($b$)$ Find the specific trajectory $x(t)$ corresponding to the initial position of the oscillator $x(0)=$

$x_0$ and the initial velocity $x^{}(0)=v_0.$ Sketch the resulting function $x(t)$ assuming $x_0=\frac{v_0}{}=$

$($c$)$ Find the amplitude $a$ of the specific trajectory $x(t)$ obtained in $($b$)$ expressed in terms of $x_0$

and $v_0.$ This can be done either by finding the maximum of $x(t)$ or by using the conservation

of energy and the fact that the potential energy of the oscillator is given by $V(x)=\frac{1}{2}k{x}^2.$