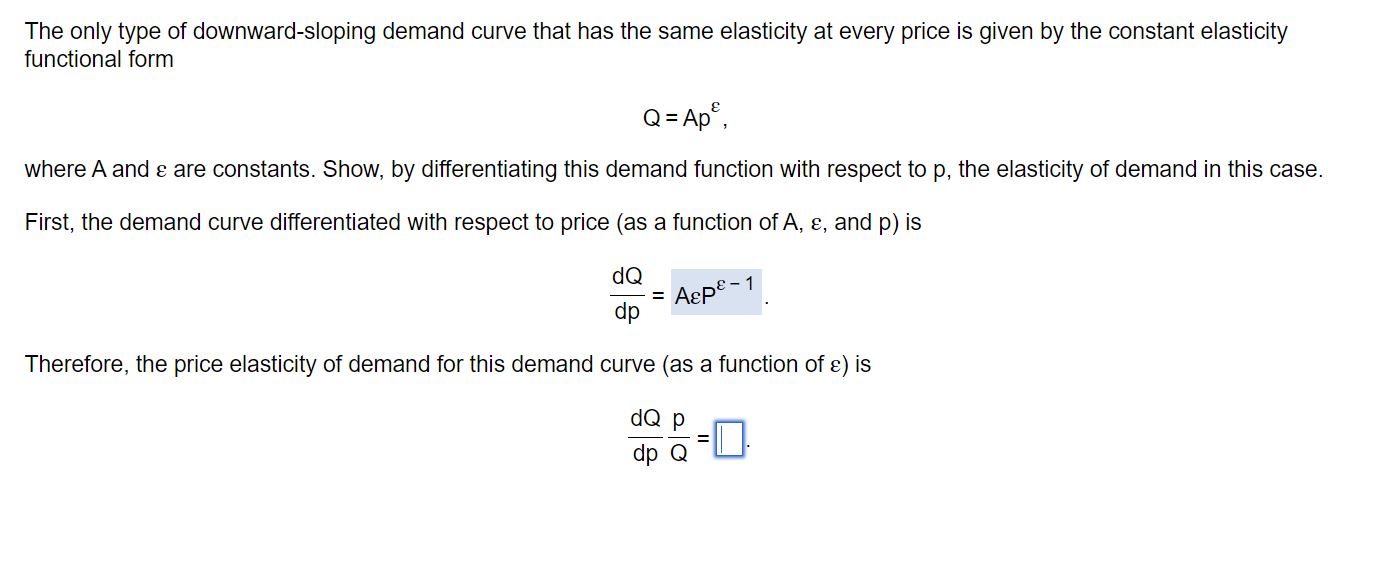
The only type of downward-sloping demand curve that has the same elasticity at every price is given by the constant elasticity functional form Q = Ap, where A and & are constants. Show, by differentiating this demand function with respect to p, the elasticity of demand in this case. First, the demand curve differentiated with respect to price (as a function of A, &, and p) is dQ dp A&P- E-1 Therefore, the price elasticity of demand for this demand curve (as a function of ) is dQ p dp Q