The question is extended on this website https://www.chegg.com/homework-help/questions-and-answers/situation-ah-lee-financial-manager-us-based-mid-sized-manufacturing-firm-caught-guard--eff-q43551998?trackid=2699e863d4e1&strackid=6e3e5e29dc5c

An expert can you help me to answer the question of (i) and Q3 on these two pictures? Some information lists on the above website. Very urgent, please Is that clear now? I posted lots of times this question, no-one can answer the (i) Perform RCY analysis on the 4 bonds. (you need to show details of your calculation) and Q3. Based on Q1s interest rate expectation and Q2s calculations, explain your recommendation on the investment of $10 million? (25 Points)

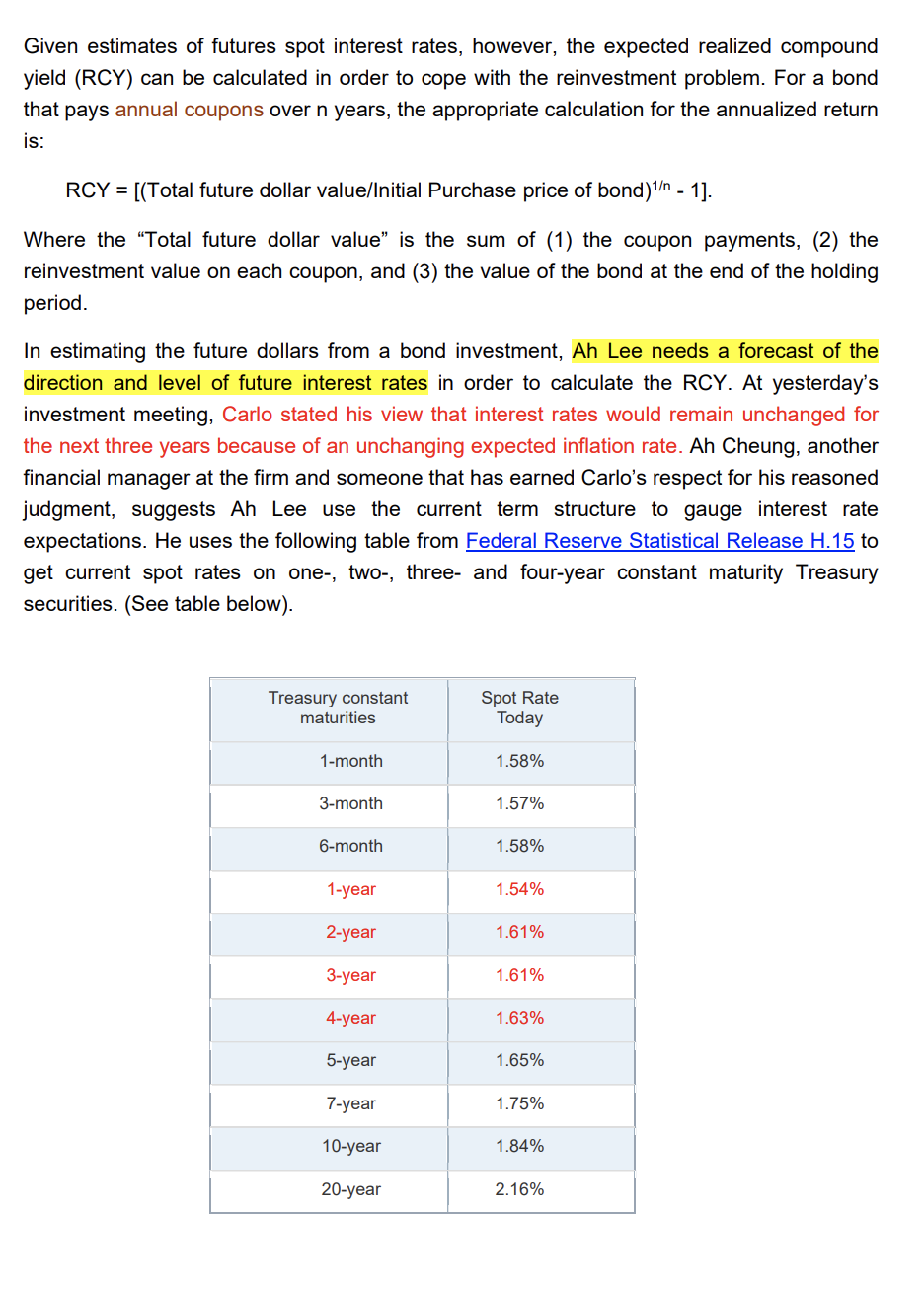
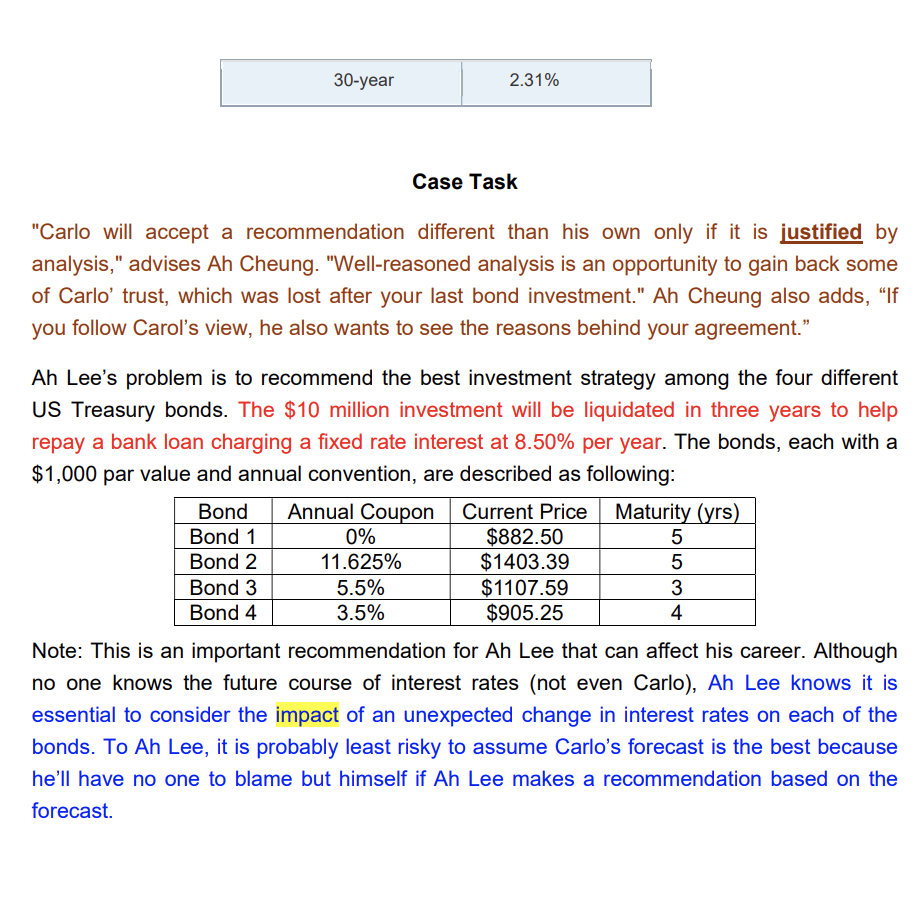
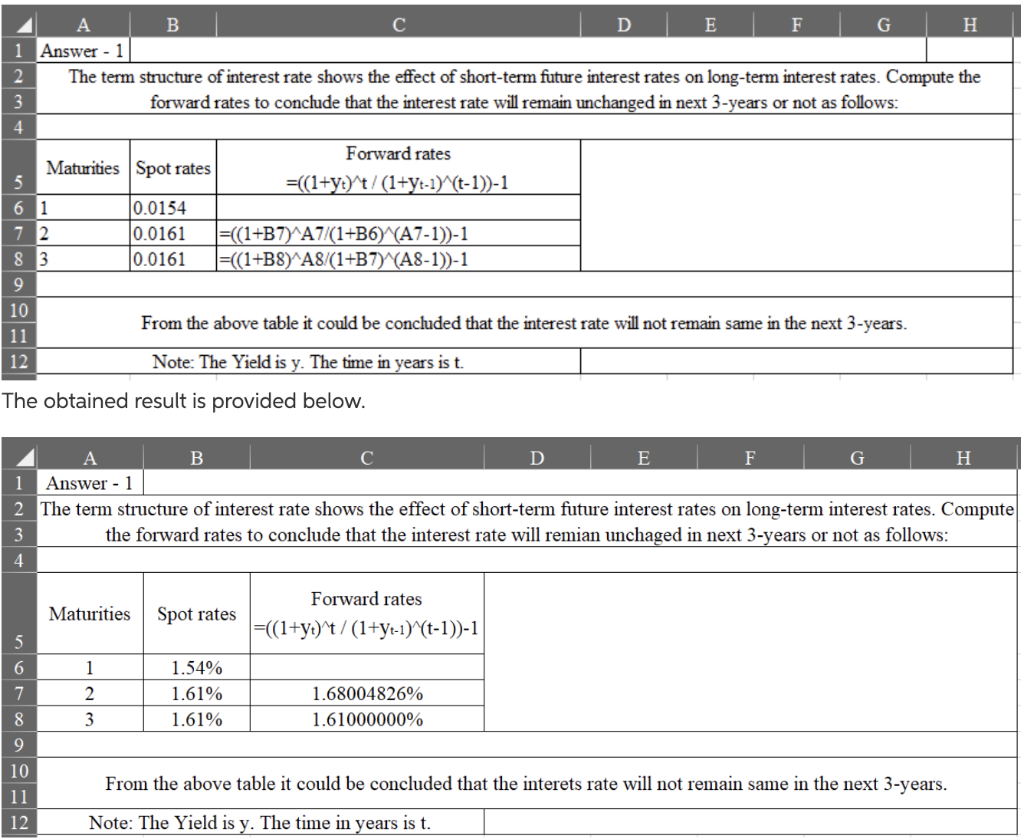
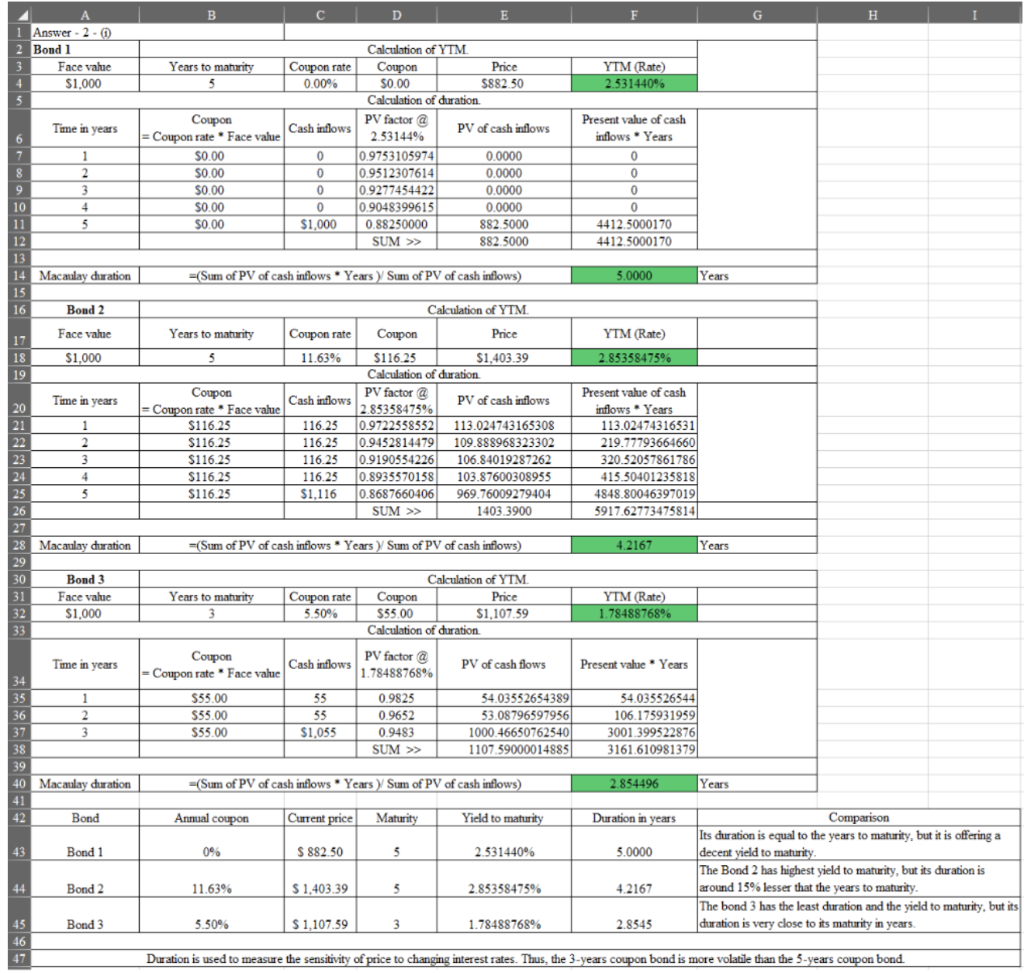
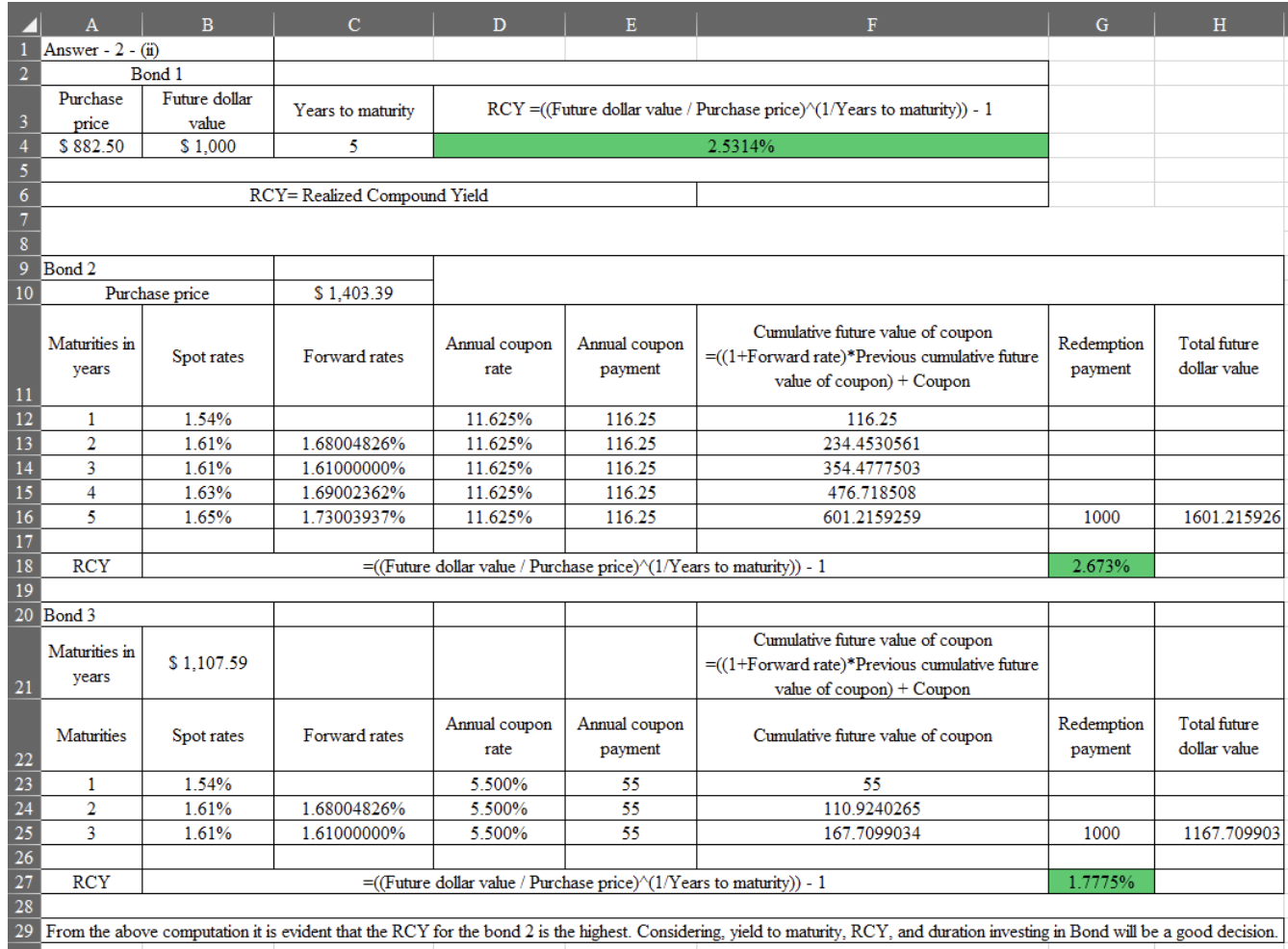
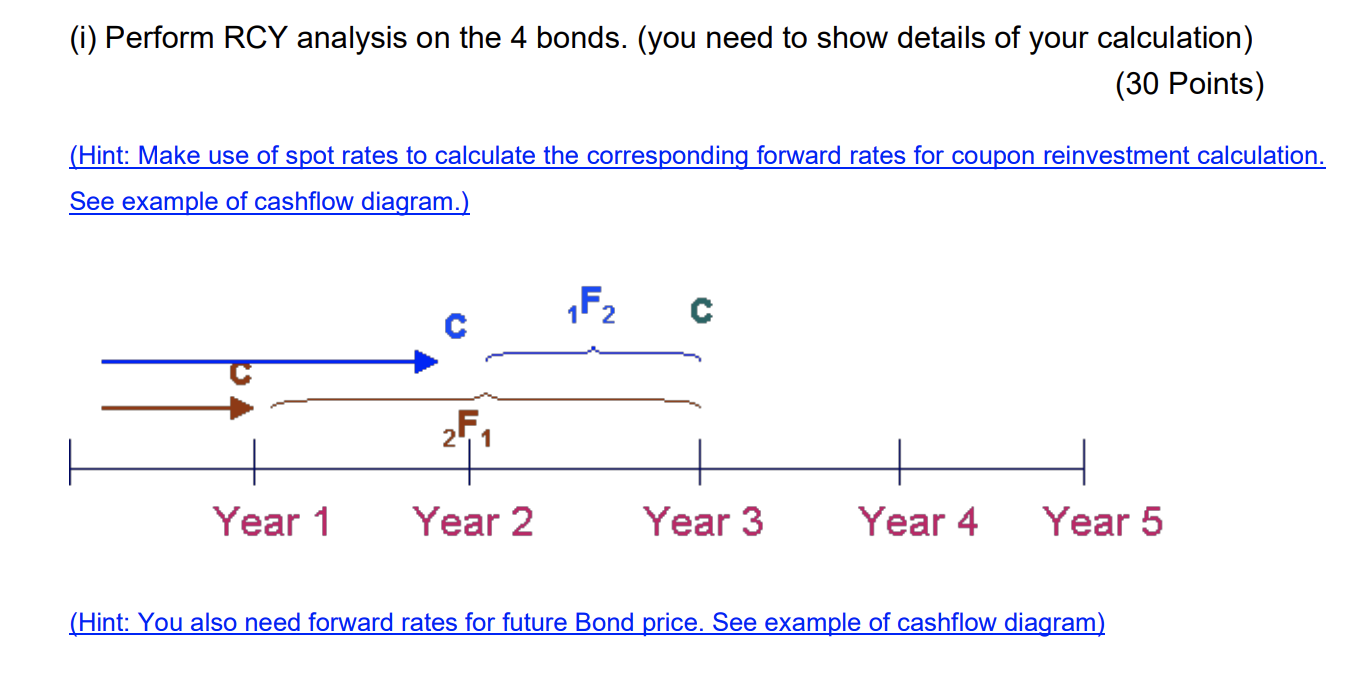
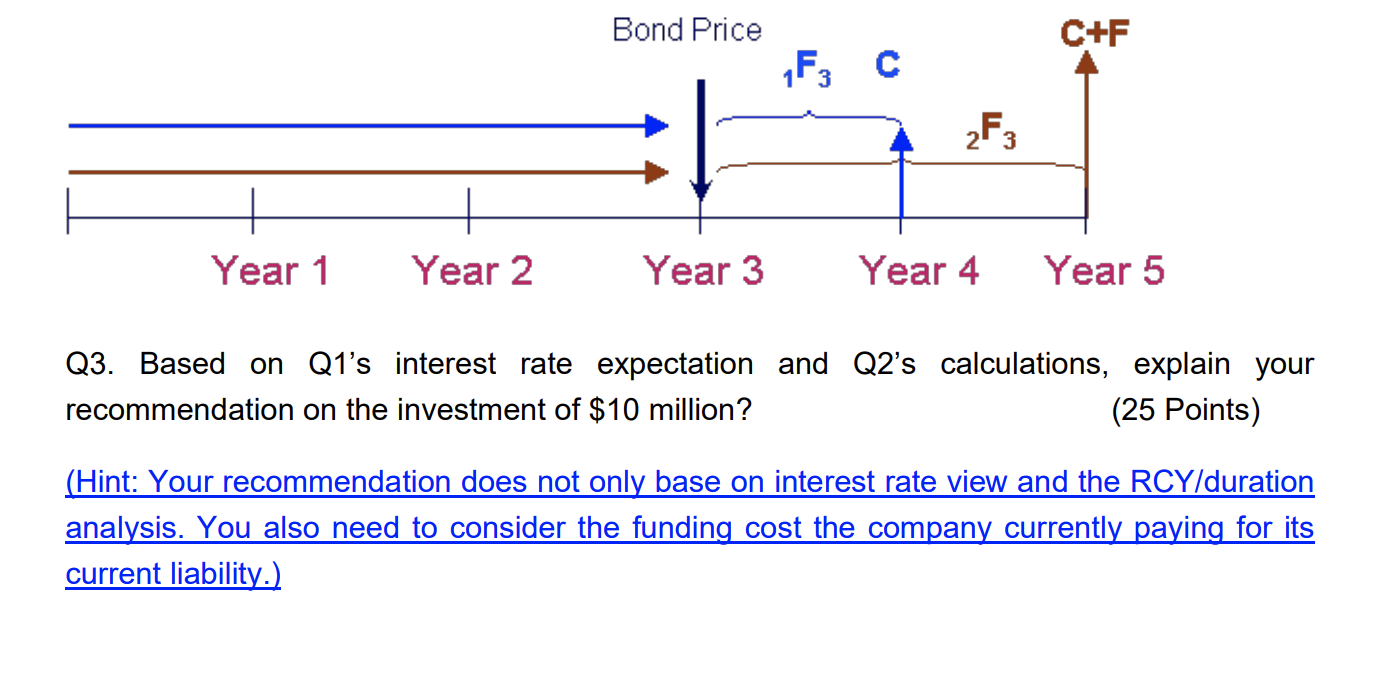
Case Background Ah Lee, a financial manager at a US based mid-sized manufacturing firm, has been caught off-guard before. To earn the most on excess cash, Ah Lee once bought five-year coupon Treasury bonds (a maturity longer than the firm's liabilities) only to see interest rates rise. The loss when the Treasury bonds were sold did not make Ah Lee's supervisor, Carlo, Chief Financial Officer, very happy. Ah Lee is now in a similar situation - Carlo has asked for a recommendation on the investment of another $10 million in excess cash. Risk and Return using RCY The risk of unexpected changes in interest rates is a given with coupon-paying bonds. Even if the bonds are held to maturity, there is reinvestment risk. A bond's exposure to interest rate risk depends on the size and the number of coupon payments made to the bondholder. The realized return from a bond depends on the rates at which the coupons are invested; the rates can only be estimated at the time the bond is purchased. Given estimates of futures spot interest rates, however, the expected realized compound yield (RCY) can be calculated in order to cope with the reinvestment problem. For a bond that pays annual coupons over n years, the appropriate calculation for the annualized return is: RCY = [(Total future dollar value/Initial Purchase price of bond) - 1]. Where the "Total future dollar value is the sum of (1) the coupon payments, (2) the reinvestment value on each coupon, and (3) the value of the bond at the end of the holding period. In estimating the future dollars from a bond investment, Ah Lee needs a forecast of the direction and level of future interest rates in order to calculate the RCY. At yesterday's investment meeting, Carlo stated his view that interest rates would remain unchanged for the next three years because of an unchanging expected inflation rate. Ah Cheung, another financial manager at the firm and someone that has earned Carlo's respect for his reasoned judgment, suggests Ah Lee use the current term structure to gauge interest rate expectations. He uses the following table from Federal Reserve Statistical Release H.15 to get current spot rates on one-, two-, three- and four-year constant maturity Treasury securities. (See table below). Treasury constant maturities Spot Rate Today 1-month 1.58% 3-month 1.57% 6-month 1.58% 1-year 1.54% 2-year 1.61% 3-year 1.61% 4-year 1.63% 5-year 1.65% 7-year 1.75% 10-year 1.84% 20-year 2.16% 30-year 2.31% Case Task "Carlo will accept a recommendation different than his own only if it is justified by analysis," advises Ah Cheung. "Well-reasoned analysis is an opportunity to gain back some of Carlo' trust, which was lost after your last bond investment." Ah Cheung also adds, "If you follow Carol's view, he also wants to see the reasons behind your agreement." Ah Lee's problem is to recommend the best investment strategy among the four different US Treasury bonds. The $10 million investment will be liquidated in three years to help repay a bank loan charging a fixed rate interest at 8.50% per year. The bonds, each with a $1,000 par value and annual convention, are described as following: Bond Annual Coupon Current Price Maturity (yrs) Bond 1 0% $882.50 Bond 2 11.625% $1403.39 Bond 3 5.5% $1107.59 3 Bond 4 3.5% $905.25 4 Note: This is an important recommendation for Ah Lee that can affect his career. Although no one knows the future course of interest rates (not even Carlo), Ah Lee knows it is essential to consider the impact of an unexpected change in interest rates on each of the bonds. To Ah Lee, it is probably least risky to assume Carlo's forecast is the best because he'll have no one to blame but himself if Ah Lee makes a recommendation based on the forecast. Case Questions Q1. Would Ah Lee go by Carol's view on interest rate remain unchanged for the next 3 years? Why or why not? (25 Points) (Note: Apart from HR issue that can affect Ah Lee's choice. I am open to what you want to discuss about the interest rate term structure over the next 3 years. Whether you agree or disagree with the view that term structure will remain the same in the coming 3 years. You should frame your discussion on interest rate view with current economic and market factors (such as ate cut after rate hike, inflation/deflation expectation, monetary/fiscal policy, trade war etc). Your interest rate view would directly affect your investment decision in Q3.) Q2. Risk and Return analysis (i) Analyse the durations of the 4 bonds. (you need to show details of your calculation) (10 Points) (ii) Explain why duration matters in the analysis. (10 Points) (Hint: You need to calculate the YTM of the 4 bonds in order to calculate the Duration. The discussion should also include company's asset and liability balance). 2 DEFGH 1 Answer - 1 2 The term structure of interest rate shows the effect of short-term future interest rates on long-term interest rates. Compute the forward rates to conclude that the interest rate will remain unchanged in next 3-years or not as follows: 3 Maturities Spot rates Forward rates (1+y) / (1+yt-1 (t-1))-1 6 1 72 8 3 0.0154 0.0161 0.0161 =((1+B7) A7/(1+B6 (A7-1))-1 =((1+B8) A8/(1+B7) A8-1))-1 9 10 From the above table it could be concluded that the interest rate will not remain same in the next 3-years. 11 Note: The Yield is y. The time in years ist The obtained result is provided below. 12 C D E F G H 1 Answer - 1 2 The term structure of interest rate shows the effect of short-term future interest rates on long-term interest rates. Compute the forward rates to conclude that the interest rate will remian unchaged in next 3-years or not as follows: Maturities Spot rates Forward rates =(1+y)^/(1+y-1)^(t-1)-1 / 1 2 3 1.54% 1.61% 1.61% 1.68004826% 1.61000000% From the above table it could be concluded that the interets rate will not remain same in the next 3-years. 11 12 Note: The Yield is y. The time in years is t. 1 Answer - 2 - 0 2 Bond 1 Face value $1.000 3 Years to maturity YTM (Rate) 2.531440% Time in years Coupon = Coupon rate * Face value $0.00 $0.00 $0.00 $0.00 $0.00 Calculation of YTM Coupon rate Coupon pon Price 0.00% $0.00 $882 50 Calculation of duration Cash inflows PV factor @ PV of cash inflows 2.33144% 10.9753105974 0.0000 0.9512307614 0.0000 0.9277454422 0.0000 0.90483996151 0.0000 $1.000 0.88250000 882.5000 SUM >> 882 5000 Present value of cash inflows Years 0 0 0 4412.5000170 4412.5000170 14 Macaulay duration =(Sum of PV of cash inflows Years Sum of PV of cash inflows) 5.0000 Years Bond 2 Years to maturity Face value $1,000 Coupon rate 11.63% YTM (Rate) 2.85358475% Cash inflows Coupon Time in years = Coupon rate Face value $116.25 21 $116.25 3 $116.25 4 $116.25 5 116.25 Calculation of YTM Coupon Price $116.25 $1,403.39 Calculation of duration PV factor @ PV of cash inflows 2.85358475% 0.9722358552 113.024743165308 0.9452814479 109.888968323302 0.9190554226 106.84019287262 0.8935570158 103.87600308955 0.8687660406 969.76009279404 SUM >> 1403.3900 116.25 116.25 116.25 116.25 $1,116 Present value of cash inflows Years 113.02474316531 219.77793664660 320.52057861786 415.50401235818 L 4848.80046397019 5917.62773475814 24 25 28 Macaulay duration -(Sum of PV of cash inflows. Years) Sum of PV of cash inflows) 4.2167 Years Bond 3 Face value $1,000 Years to maturity Coupon rate 5.50% Calculation of YTM Coupon Price $35.00 $1,107.59 Calculation of duration YTM (Rate) 1.78488768% Time in years Cash inflows PV factor @ 1.78488768% PV of cash flows Present value Years Coupon - Coupon rateFace value $55.00 $55.00 $55.00 55 $1.055 0.9825 0.9652 0.9483 SUM >> 34.03552654389 5 3.08796597956 1 000.46650762540 1107.59000014885 34.035526344 106.175931959 3 001.399522876 3161.610981379 40 Macaulay duration (Sum of PV of cash inflows * Years) Sum of PV of cash inflows) 2.854496 Years Bond Annual coupon Current price Maturity Yield to maturity Duration in years Bond 1 0% S 882.50 2.531440% 5.0000 Comparison Its duration is equal to the years to maturity, but it is offering a decent yield to maturity. The Bond 2 has highest yield to maturity, but its duration is around 15% lesser that the years to maturity. The bond 3 has the least duration and the yield to maturity, but its duration is very close to its maturity in years. Bond 2 11.63% $ 1.403.39 2.85358475% 4.2167 Bond 3 5.50% $ 1,107.59 1.78488768% 2.8545 Duration is used to measure the sensitivity of price to changing interest rates. Thus, the 3-years coupon bond is more volatile than the 5-years coupon bond G H 2 1 Answer - 2 - Bond 1 Purchase Future dollar price value 4 S 882.50 $1,000 1 Years to maturity RCY =((Future dollar value / Purchase price) (1/Years to maturity)) - 1 2.5314% RCY=Realized Compound Yield 9 Bond 2 10 Purchase price $ 1,403.39 Maturities in years Spot rates Forward rates Annual coupon rate Annual coupon payment Cumulative future value of coupon =((1+Forward rate) *Previous cumulative future value of coupon) + Coupon Redemption payment Total future dollar value 12 13 14 15 16 17 18 1 2 3 4 5 1.54% 1.61% 1.61% 1.63% 1.65% 1.68004826% 1.61000000% 1.69002362% 1.73003937% 11.625% 11.625% 11.625% 11.625% 11.625% 116.25 116.25 116.25 116.25 116.25 116.25 234.4530561 354.4777503 476.718508 601.2159259 1000 L 1601.215926 RCY =((Future dollar value / Purchase price) (1/Years to maturity)) - 1 2.673% 19 20 Bond 3 Maturities in $ 1,107.59 Cumulative future value of coupon =((1+Forward rate) *Previous cumulative future value of coupon) + Coupon 21 years Maturities Spot rates Forward rates Annual coupon rate Annual coupon payment Cumulative future value of coupon Redemption payment Total future dollar value 1 55 24 1.54% 1.61% 1.61% 1.68004826% 1.61000000% 5.500% 5.500% 5.500% 55 55 55 110.9240265 167.7099034 25 3 1000 1167.709903 26 27 RCY =((Future dollar value / Purchase price) (1/Years to maturity)) - 1 1.7775% 28 29 From the above computation it is evident that the RCY for the bond 2 is the highest. Considering, yield to maturity, RCY, and duration investing in Bond will be a good decision. (i) Perform RCY analysis on the 4 bonds. (you need to show details of your calculation) (30 points) (Hint: Make use of spot rates to calculate the corresponding forward rates for coupon reinvestment calculation. See example of cashflow diagram.). F2 21 Year 1 Year 2 Year 3 Year 4 Year 5 (Hint: You also need forward rates for future Bond price. See example of cashflow diagram) Bond Price C+F 2F3 Year 1 Year 2 Year 3 Year 4 Year 5 Q3. Based on Q1's interest rate expectation and Q2's calculations, explain your recommendation on the investment of $10 million? (25 Points) (Hint: Your recommendation does not only base on interest rate view and the RCY/duration analysis. You also need to consider the funding cost the company currently paying for its current liability.) Case Background Ah Lee, a financial manager at a US based mid-sized manufacturing firm, has been caught off-guard before. To earn the most on excess cash, Ah Lee once bought five-year coupon Treasury bonds (a maturity longer than the firm's liabilities) only to see interest rates rise. The loss when the Treasury bonds were sold did not make Ah Lee's supervisor, Carlo, Chief Financial Officer, very happy. Ah Lee is now in a similar situation - Carlo has asked for a recommendation on the investment of another $10 million in excess cash. Risk and Return using RCY The risk of unexpected changes in interest rates is a given with coupon-paying bonds. Even if the bonds are held to maturity, there is reinvestment risk. A bond's exposure to interest rate risk depends on the size and the number of coupon payments made to the bondholder. The realized return from a bond depends on the rates at which the coupons are invested; the rates can only be estimated at the time the bond is purchased. Given estimates of futures spot interest rates, however, the expected realized compound yield (RCY) can be calculated in order to cope with the reinvestment problem. For a bond that pays annual coupons over n years, the appropriate calculation for the annualized return is: RCY = [(Total future dollar value/Initial Purchase price of bond) - 1]. Where the "Total future dollar value is the sum of (1) the coupon payments, (2) the reinvestment value on each coupon, and (3) the value of the bond at the end of the holding period. In estimating the future dollars from a bond investment, Ah Lee needs a forecast of the direction and level of future interest rates in order to calculate the RCY. At yesterday's investment meeting, Carlo stated his view that interest rates would remain unchanged for the next three years because of an unchanging expected inflation rate. Ah Cheung, another financial manager at the firm and someone that has earned Carlo's respect for his reasoned judgment, suggests Ah Lee use the current term structure to gauge interest rate expectations. He uses the following table from Federal Reserve Statistical Release H.15 to get current spot rates on one-, two-, three- and four-year constant maturity Treasury securities. (See table below). Treasury constant maturities Spot Rate Today 1-month 1.58% 3-month 1.57% 6-month 1.58% 1-year 1.54% 2-year 1.61% 3-year 1.61% 4-year 1.63% 5-year 1.65% 7-year 1.75% 10-year 1.84% 20-year 2.16% 30-year 2.31% Case Task "Carlo will accept a recommendation different than his own only if it is justified by analysis," advises Ah Cheung. "Well-reasoned analysis is an opportunity to gain back some of Carlo' trust, which was lost after your last bond investment." Ah Cheung also adds, "If you follow Carol's view, he also wants to see the reasons behind your agreement." Ah Lee's problem is to recommend the best investment strategy among the four different US Treasury bonds. The $10 million investment will be liquidated in three years to help repay a bank loan charging a fixed rate interest at 8.50% per year. The bonds, each with a $1,000 par value and annual convention, are described as following: Bond Annual Coupon Current Price Maturity (yrs) Bond 1 0% $882.50 Bond 2 11.625% $1403.39 Bond 3 5.5% $1107.59 3 Bond 4 3.5% $905.25 4 Note: This is an important recommendation for Ah Lee that can affect his career. Although no one knows the future course of interest rates (not even Carlo), Ah Lee knows it is essential to consider the impact of an unexpected change in interest rates on each of the bonds. To Ah Lee, it is probably least risky to assume Carlo's forecast is the best because he'll have no one to blame but himself if Ah Lee makes a recommendation based on the forecast. Case Questions Q1. Would Ah Lee go by Carol's view on interest rate remain unchanged for the next 3 years? Why or why not? (25 Points) (Note: Apart from HR issue that can affect Ah Lee's choice. I am open to what you want to discuss about the interest rate term structure over the next 3 years. Whether you agree or disagree with the view that term structure will remain the same in the coming 3 years. You should frame your discussion on interest rate view with current economic and market factors (such as ate cut after rate hike, inflation/deflation expectation, monetary/fiscal policy, trade war etc). Your interest rate view would directly affect your investment decision in Q3.) Q2. Risk and Return analysis (i) Analyse the durations of the 4 bonds. (you need to show details of your calculation) (10 Points) (ii) Explain why duration matters in the analysis. (10 Points) (Hint: You need to calculate the YTM of the 4 bonds in order to calculate the Duration. The discussion should also include company's asset and liability balance). 2 DEFGH 1 Answer - 1 2 The term structure of interest rate shows the effect of short-term future interest rates on long-term interest rates. Compute the forward rates to conclude that the interest rate will remain unchanged in next 3-years or not as follows: 3 Maturities Spot rates Forward rates (1+y) / (1+yt-1 (t-1))-1 6 1 72 8 3 0.0154 0.0161 0.0161 =((1+B7) A7/(1+B6 (A7-1))-1 =((1+B8) A8/(1+B7) A8-1))-1 9 10 From the above table it could be concluded that the interest rate will not remain same in the next 3-years. 11 Note: The Yield is y. The time in years ist The obtained result is provided below. 12 C D E F G H 1 Answer - 1 2 The term structure of interest rate shows the effect of short-term future interest rates on long-term interest rates. Compute the forward rates to conclude that the interest rate will remian unchaged in next 3-years or not as follows: Maturities Spot rates Forward rates =(1+y)^/(1+y-1)^(t-1)-1 / 1 2 3 1.54% 1.61% 1.61% 1.68004826% 1.61000000% From the above table it could be concluded that the interets rate will not remain same in the next 3-years. 11 12 Note: The Yield is y. The time in years is t. 1 Answer - 2 - 0 2 Bond 1 Face value $1.000 3 Years to maturity YTM (Rate) 2.531440% Time in years Coupon = Coupon rate * Face value $0.00 $0.00 $0.00 $0.00 $0.00 Calculation of YTM Coupon rate Coupon pon Price 0.00% $0.00 $882 50 Calculation of duration Cash inflows PV factor @ PV of cash inflows 2.33144% 10.9753105974 0.0000 0.9512307614 0.0000 0.9277454422 0.0000 0.90483996151 0.0000 $1.000 0.88250000 882.5000 SUM >> 882 5000 Present value of cash inflows Years 0 0 0 4412.5000170 4412.5000170 14 Macaulay duration =(Sum of PV of cash inflows Years Sum of PV of cash inflows) 5.0000 Years Bond 2 Years to maturity Face value $1,000 Coupon rate 11.63% YTM (Rate) 2.85358475% Cash inflows Coupon Time in years = Coupon rate Face value $116.25 21 $116.25 3 $116.25 4 $116.25 5 116.25 Calculation of YTM Coupon Price $116.25 $1,403.39 Calculation of duration PV factor @ PV of cash inflows 2.85358475% 0.9722358552 113.024743165308 0.9452814479 109.888968323302 0.9190554226 106.84019287262 0.8935570158 103.87600308955 0.8687660406 969.76009279404 SUM >> 1403.3900 116.25 116.25 116.25 116.25 $1,116 Present value of cash inflows Years 113.02474316531 219.77793664660 320.52057861786 415.50401235818 L 4848.80046397019 5917.62773475814 24 25 28 Macaulay duration -(Sum of PV of cash inflows. Years) Sum of PV of cash inflows) 4.2167 Years Bond 3 Face value $1,000 Years to maturity Coupon rate 5.50% Calculation of YTM Coupon Price $35.00 $1,107.59 Calculation of duration YTM (Rate) 1.78488768% Time in years Cash inflows PV factor @ 1.78488768% PV of cash flows Present value Years Coupon - Coupon rateFace value $55.00 $55.00 $55.00 55 $1.055 0.9825 0.9652 0.9483 SUM >> 34.03552654389 5 3.08796597956 1 000.46650762540 1107.59000014885 34.035526344 106.175931959 3 001.399522876 3161.610981379 40 Macaulay duration (Sum of PV of cash inflows * Years) Sum of PV of cash inflows) 2.854496 Years Bond Annual coupon Current price Maturity Yield to maturity Duration in years Bond 1 0% S 882.50 2.531440% 5.0000 Comparison Its duration is equal to the years to maturity, but it is offering a decent yield to maturity. The Bond 2 has highest yield to maturity, but its duration is around 15% lesser that the years to maturity. The bond 3 has the least duration and the yield to maturity, but its duration is very close to its maturity in years. Bond 2 11.63% $ 1.403.39 2.85358475% 4.2167 Bond 3 5.50% $ 1,107.59 1.78488768% 2.8545 Duration is used to measure the sensitivity of price to changing interest rates. Thus, the 3-years coupon bond is more volatile than the 5-years coupon bond G H 2 1 Answer - 2 - Bond 1 Purchase Future dollar price value 4 S 882.50 $1,000 1 Years to maturity RCY =((Future dollar value / Purchase price) (1/Years to maturity)) - 1 2.5314% RCY=Realized Compound Yield 9 Bond 2 10 Purchase price $ 1,403.39 Maturities in years Spot rates Forward rates Annual coupon rate Annual coupon payment Cumulative future value of coupon =((1+Forward rate) *Previous cumulative future value of coupon) + Coupon Redemption payment Total future dollar value 12 13 14 15 16 17 18 1 2 3 4 5 1.54% 1.61% 1.61% 1.63% 1.65% 1.68004826% 1.61000000% 1.69002362% 1.73003937% 11.625% 11.625% 11.625% 11.625% 11.625% 116.25 116.25 116.25 116.25 116.25 116.25 234.4530561 354.4777503 476.718508 601.2159259 1000 L 1601.215926 RCY =((Future dollar value / Purchase price) (1/Years to maturity)) - 1 2.673% 19 20 Bond 3 Maturities in $ 1,107.59 Cumulative future value of coupon =((1+Forward rate) *Previous cumulative future value of coupon) + Coupon 21 years Maturities Spot rates Forward rates Annual coupon rate Annual coupon payment Cumulative future value of coupon Redemption payment Total future dollar value 1 55 24 1.54% 1.61% 1.61% 1.68004826% 1.61000000% 5.500% 5.500% 5.500% 55 55 55 110.9240265 167.7099034 25 3 1000 1167.709903 26 27 RCY =((Future dollar value / Purchase price) (1/Years to maturity)) - 1 1.7775% 28 29 From the above computation it is evident that the RCY for the bond 2 is the highest. Considering, yield to maturity, RCY, and duration investing in Bond will be a good decision. (i) Perform RCY analysis on the 4 bonds. (you need to show details of your calculation) (30 points) (Hint: Make use of spot rates to calculate the corresponding forward rates for coupon reinvestment calculation. See example of cashflow diagram.). F2 21 Year 1 Year 2 Year 3 Year 4 Year 5 (Hint: You also need forward rates for future Bond price. See example of cashflow diagram) Bond Price C+F 2F3 Year 1 Year 2 Year 3 Year 4 Year 5 Q3. Based on Q1's interest rate expectation and Q2's calculations, explain your recommendation on the investment of $10 million? (25 Points) (Hint: Your recommendation does not only base on interest rate view and the RCY/duration analysis. You also need to consider the funding cost the company currently paying for its current liability.)