The questions are in the photos below: I would like all of them solved, and the correct answers too.

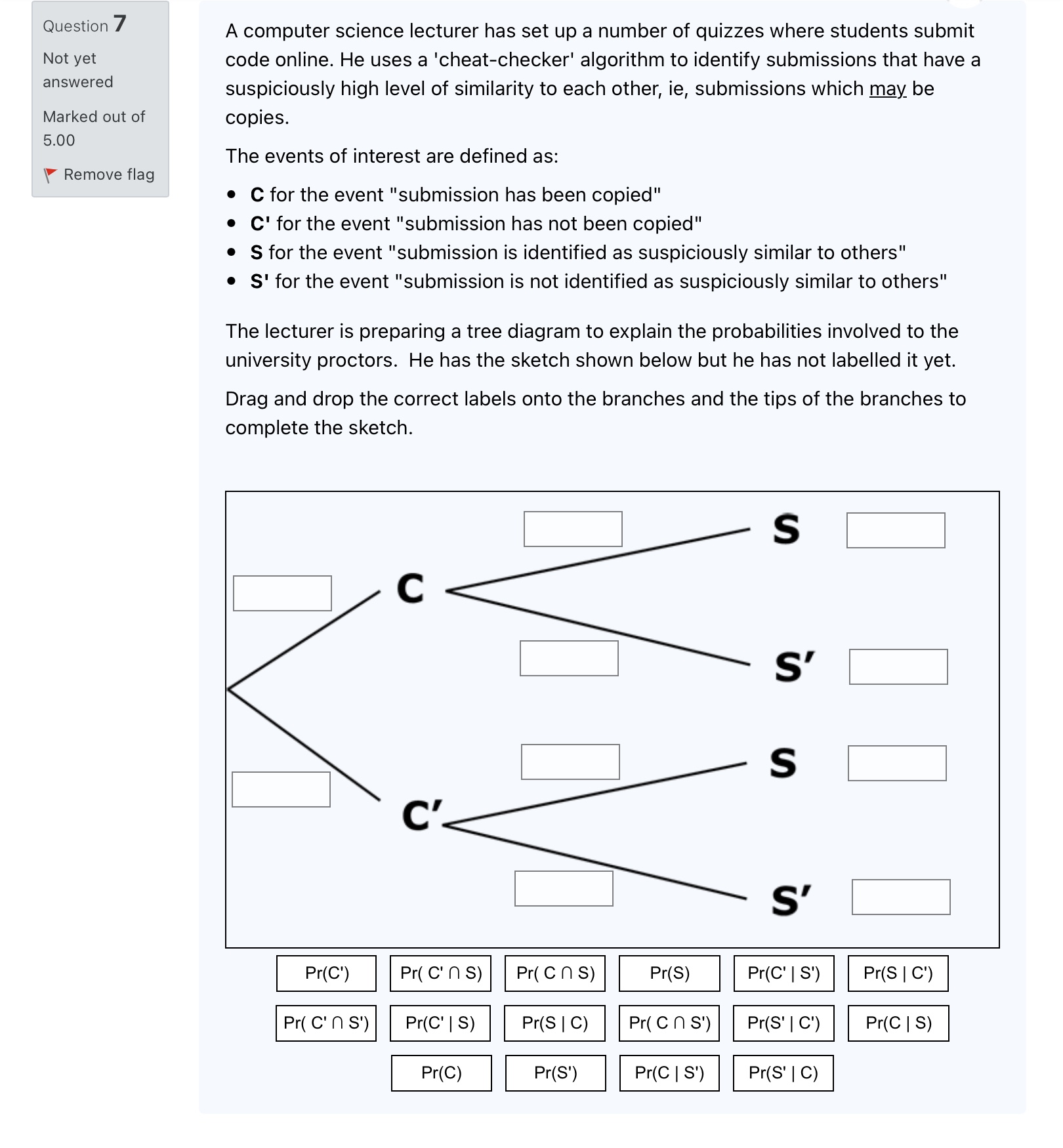
Question 7 Not yet answered Marked out of 5.00 \\V Remove flag A computer science lecturer has set up a number of quizzes where students submit code online. He uses a 'cheat-checker' algorithm to identify submissions that have a suspiciously high level of similarity to each other, ier submissions which my be copies. The events of interest are defined as: 0 C for the event "submission has been copied" 0 C' for the event "submission has not been copied" 0 S for the event "submission is identified as suspiciously similar to others" 0 S' for the event "submission is not identified as suspiciously similar to others" The lecturer is preparing a tree diagram to explain the probabilities involved to the university proctors. He has the sketch shown below but he has not labelled it yet. Drag and drop the correct labels onto the branches and the tips of the branches to complete the sketch. S cgs S S S'S Pr( 0' n S') Pr(C' | S) Pr(S | C) Pr( 0 n 5') Pr(S' | C') Pr(C | S) Pr(C) Pr(S') Pr(C | S') Pr(S' | C) QUEEN)\" 9 An accounting firm summarises their clients in terms of the sector and the number of them that sent late tax returns in the Not yet following table: answered Sector Number sending late returns Number on time Total number of clients Marked out of 100 Health 2 13 15 l7 Flag Real estate 5 73 78 queSOH Retail 5 52 57 Other 30 97 127 Total 42 235 277 If a client is from Real Estate, what is the probability of sending their return on time? Give your answer to 2 decimal places. Answer: Question 15 Not yet answered Marked out of 1.00 '7 Flag question A very large accounting firm has found that 15% of the tax returns they process are very complex. A new technician is given 10 returns to process. What is the probability that fewer than 2 of them will be very complex? Give the answer to 3 decimal places. Answer: Question 3 Not yet answered Marked out of 1.00 V Flag question Which of the following describes collectively exhaustive events? Select one: Q a. A person's buying choices: Event the person buys the product; Event the person does not buy the product. 0 b. Events concerning an individual: Event that the person misses the bus to work; Event that the person has a black briefcase. O c. Planning a picnic: Event that Friday 13th is chosen for a picnic; Event that it rains on the day of the picnic. Q d. Type of job applicant: Event the job applicant is an NZ citizen; Event the job applicant is a man. Question 5 Not yet answered Marked out of 1.00 '7 Flag question The lifetime of a certain brand of batteries is known to be normally distributed. A sample of 21 batteries gives a mean lifetime of 134.0 hours with a standard deviation of 7.0 hours. Use Excel to calculate the upper limit of a 95% confidence interval for the mean lifetime of batteries of this brand. Give your answer to 1 decimal place. We are standardising )2 with distribution to calculate 2*). Answer: S W so we will need to use the t distribution to calculate t* (rather than using the Standard Normal Quesnon 8 An accounting firm summarises their clients in terms of the sector and the number of them that sent late tax returns in the Not yet following table: answered Sector Number sending late returns Number on time Total number of clients Marked out of 100 Health 13 2 15 '7 Flag Real estate 5 73 78 question Retail 5 52 57 Other 30 97 127 Total 42 235 277 An account is chosen at random. What is the probability that it is from the Health sector and had a late return? Give your answer to 2 decimal places. Answer: Question 18 Not yet answered Marked out of 1.00 F Flag question The times for a running race have a mean of 75 minutes and a standard deviation of 15 minutes, and are well approximated by the normal distribution. A runner wants to be in the fastest 10% of times. What is the longest time she can take in the race and still be in the top 10%? Remember that the top 10% of runners are the lower 10% of the distribution of times! It may help if you draw yourself a diagram. Give the answer to one decimal place (omit the units, just give the numbers)