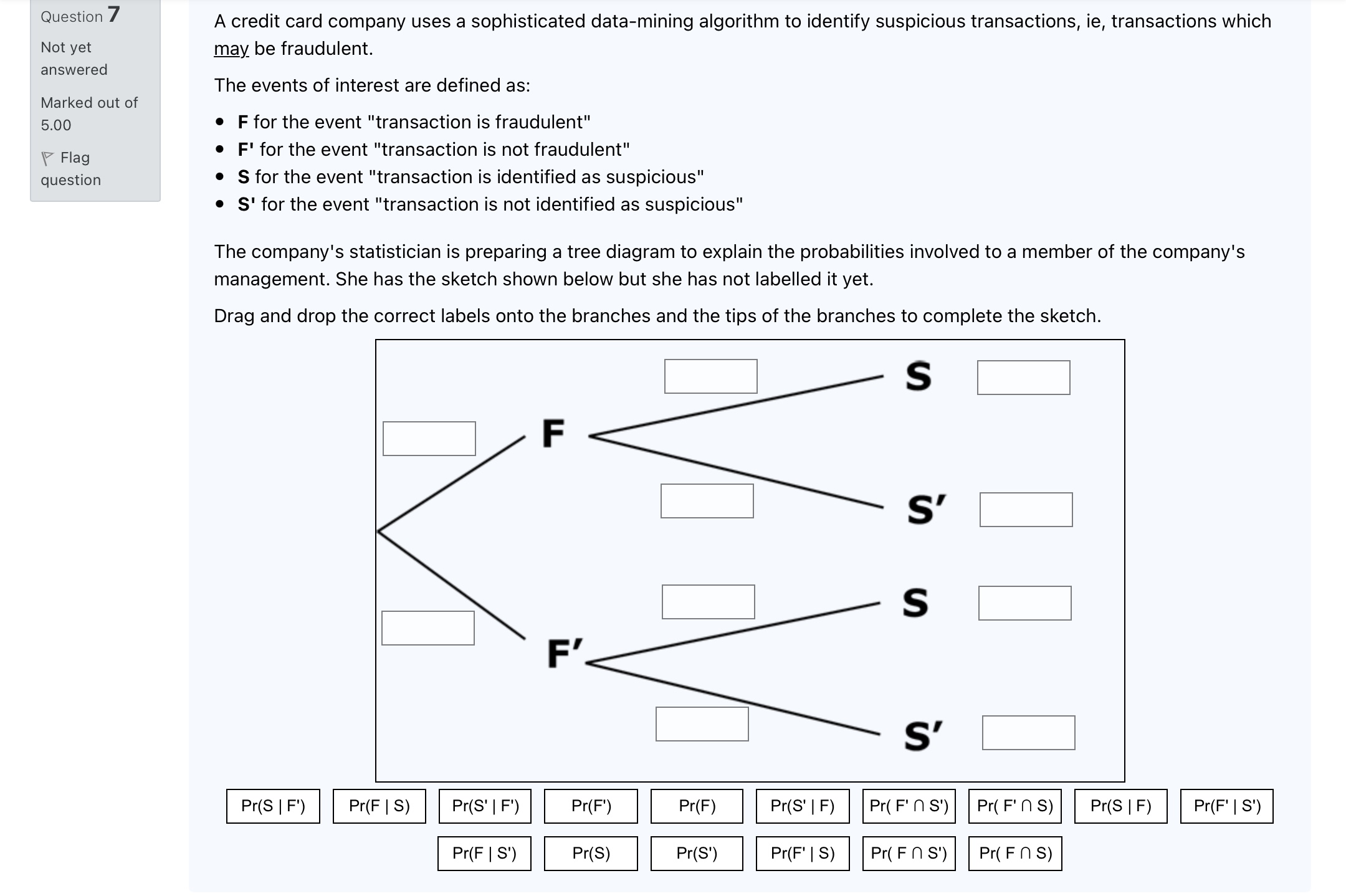
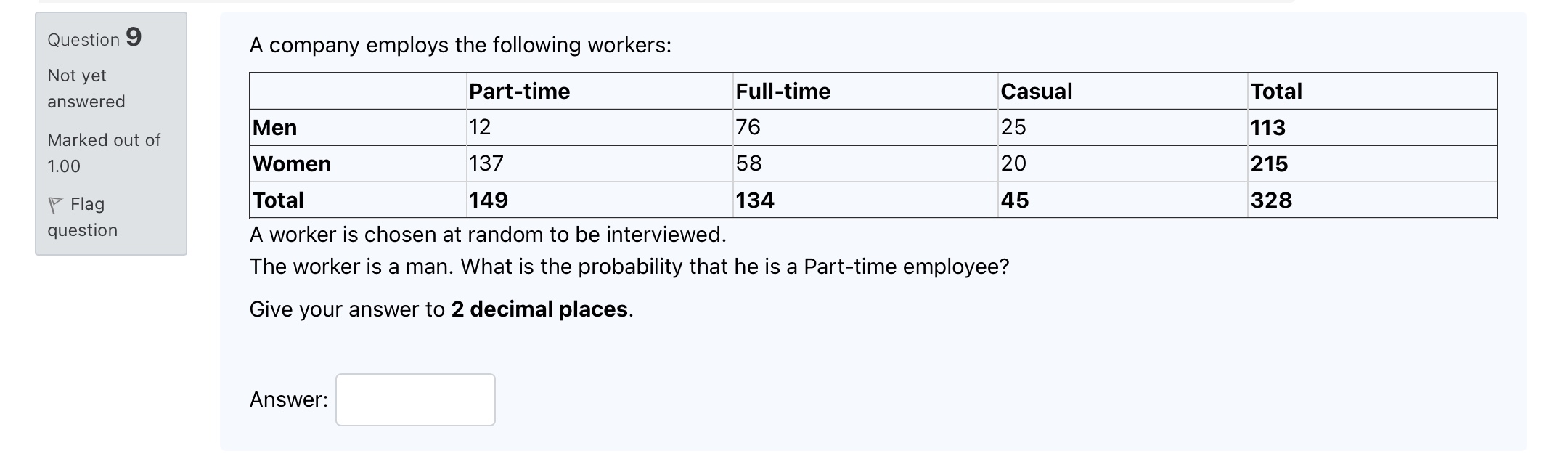
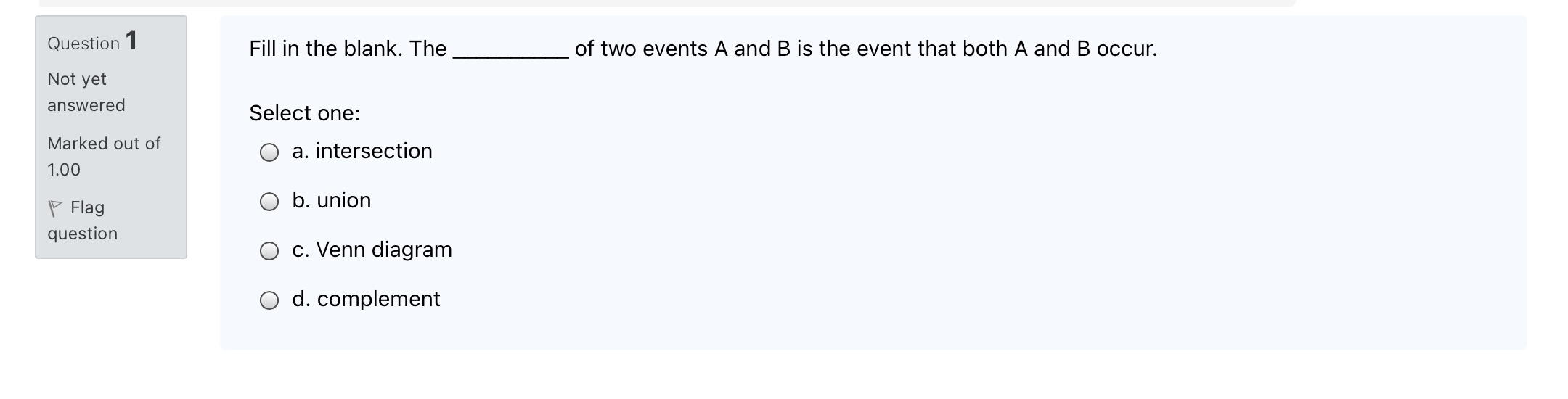
The questions are in the photos below: I would like all of them solved correctly, and the correct answer options too.

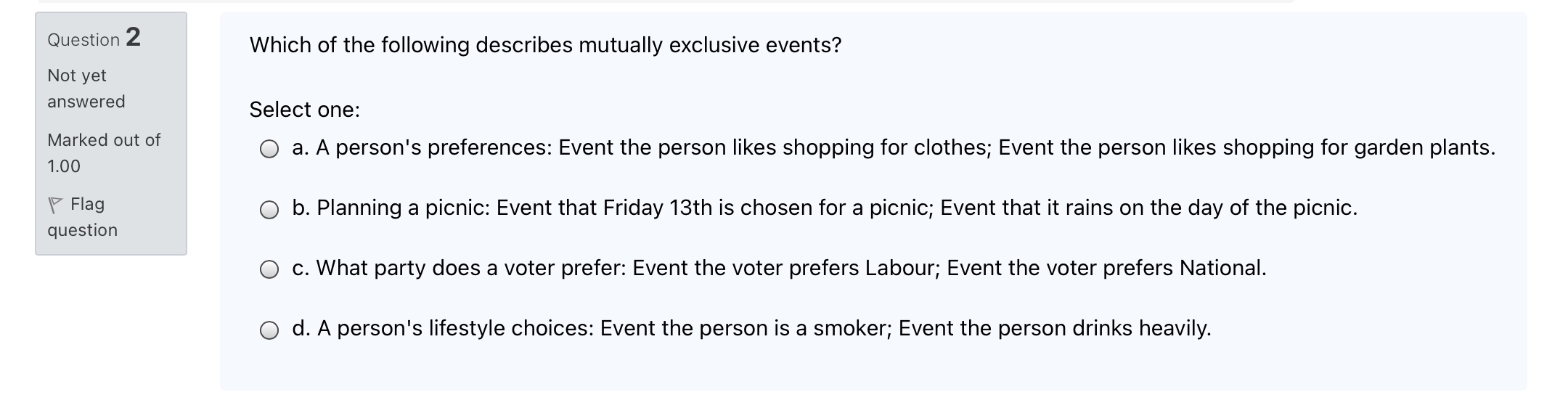
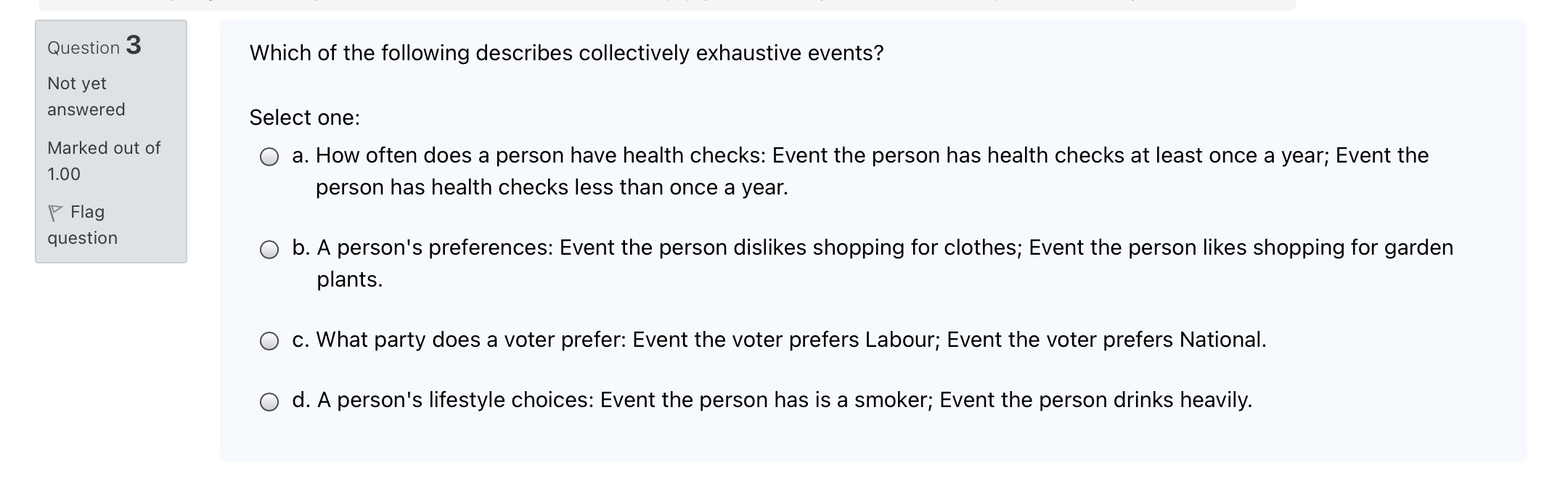
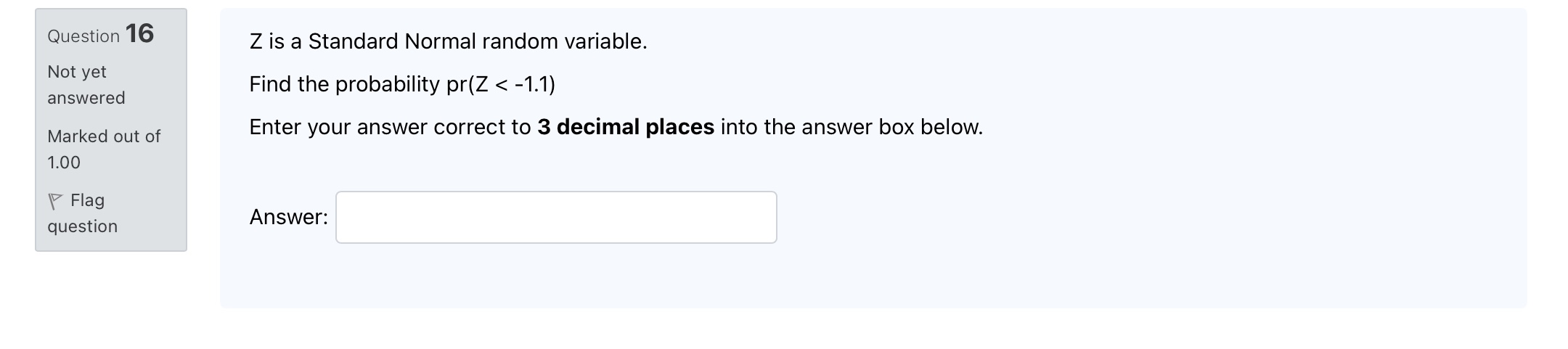
Question 10 Not yet answered Marked out of 1.00 '7 Flag question Suppose a basketball player is an excellent free throw shooter and makes 96% of his free throws (i.e., he has a 96% chance of making a single free throw). Assume that free throw shots are independent of one another. Suppose this player gets to shoot three free throws. Find the probability (calculated to 4 decimal places) that he misses all three consecutive free throws. Select one: 0 a. 0.9999 0 b. 0.0001 0 c. 0.1153 0 d. 0.8847 Question 2 Not yet answered Marked out of 1.00 F Flag question Which of the following describes mutually exclusive events? Select one: Q a. A person's preferences: Event the person likes shopping for clothes; Event the person likes shopping for garden plants. 0 b. Planning a picnic: Event that Friday 13th is chosen for a picnic; Event that it rains on the day of the picnic. O c. What party does a voter prefer: Event the voter prefers Labour; Event the voter prefers National. 0 d. A person's lifestyle choices: Event the person is a smoker; Event the person drinks heavily. Question 17 Not yet answered Marked out of 1.00 'F Flag question A filling machine fills jars with syrup. The amounts poured is well approximated by a Normal distribution. The mean amount poured is 503ml, with a standard deviation of 1.4ml. What is the probability that a randomly chosen container will have less than 500ml of syrup in it? Give the answer to 3 decimal places. Answer: Question 5 Not yet answered Marked out of 1.00 '7 Flag question A Canterbury energy agency mailed questionnaires on energy conservation to 1,000 homeowners in Christchurch. Five hundred questionnaires were returned. Suppose an experiment consists of randomly selecting one of the returned questionnaires. Consider the events: A: {The home is constructed of brick} B: {The home is more than 30 years old} In terms of A and B, describe a home that is constructed of brick and is more than 30 years old. Selectone: O a.AnB O b.(AnB)'3 O c.AnBc O d.AUB QUQSth" 8 An accounting firm summarises their clients in terms of the sector and the number of them that sent late tax returns in the Not yet following table: answered Sector Number sending late returns Number on time Total number of clients Marked out of 100 Health 2 13 15 '7 Flag Real estate 5 73 78 question Retail 5 52 57 Other 30 97 127 Total 42 235 277 An account is chosen at random. What is the probability that it is from the Retail sector and had an on-time return? Give your answer to 2 decimal places. Answer: Question 3 Not yet answered Marked out of 1.00 '7 Flag question Which of the following describes collectively exhaustive events? Select one: Q a. How often does a person have health checks: Event the person has health checks at least once a year; Event the person has health checks less than once a year. O b. A person's preferences: Event the person dislikes shopping for clothes; Event the person likes shopping for garden plants. 0 c. What party does a voter prefer: Event the voter prefers Labour; Event the voter prefers National. 0 d. A person's lifestyle choices: Event the person has is a smoker; Event the person drinks heavily. Question 15 Not yet answered Marked out of 100 '7 Flag question The leading brand of dishwasher detergent has 20% market share. A random sample of 30 dishwasher detergent customers is taken and asked which brand they use. What is the probability that exactly 4 or exactly 5 of the customers in the sample use the leading brand? Give the answer to 3 decimal places. Answer: Question 4 Not yet answered Marked out of 100 '7 Flag question Classify the events as independent or not independent. Events A and B where P(A) = 0.6, P(B) = 0.3, and P(A and B) = 0.2 Select one: 0 a. independent 0 b. not independent Question 12 Not yet answered Marked out of 1.00 F Flag question Calculate the mean for the discrete probability distribution shown here. x 3 4 5 1O pr(X=x) 0.20 0.24 0.21 0.35 Select one: Q a. 1.0 O b. 22 O c. 6.11 Q d. 6.29 Question 18 Not yet answered Marked out of 1.00 \\V Flag question Tests are scaled to a mean of 60% and a standard deviation of 15%. Marcus wants to be in the top 5% of the class. Assuming that the distribution of the marks is approximated by a normal distribution, find the minimum mark that Marcus needs to get to be in the top 5%. Give the answer to one decimal place. (Omit the units, just give the numbers. For example, if you think the answer is 10.1%, type in 10.1) Answer: Question 16 Not yet answered Marked out of 100 '7 Flag question Z is a Standard Normal random variable. Find the probability pr(Z