Question: The random vector (X,Y,Z) follows a multivariate Normal distribution with mean vector 0 = (0,0,0) and covariance matrix 1 1 (249) In particular, X

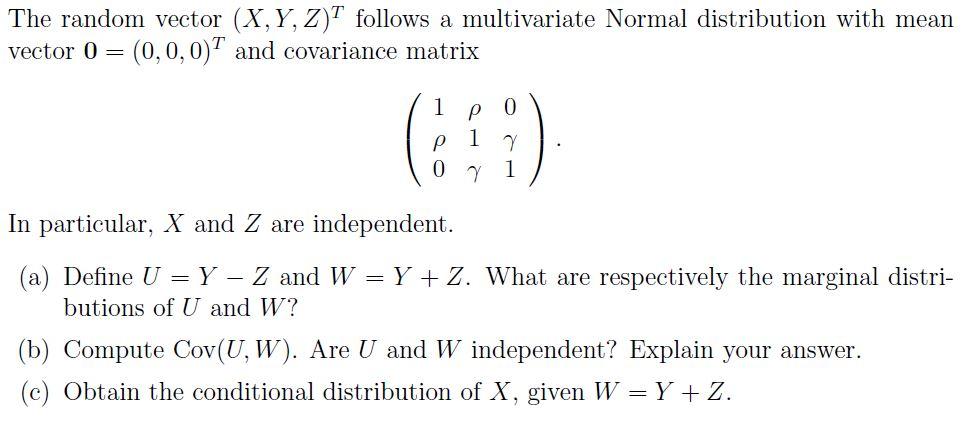
The random vector (X,Y,Z) follows a multivariate Normal distribution with mean vector

The random vector (X,Y,Z) follows a multivariate Normal distribution with mean vector 0 = (0,0,0) and covariance matrix 1 1 (249) In particular, X and Z are independent. 1 (a) Define U Y - Z and W =Y+Z. What are respectively the marginal distri- butions of U and W? (b) Compute Cov(U, W). Are U and W independent? Explain your answer. (c) Obtain the conditional distribution of X, given W = Y + Z.

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Finance Questions!

At a champagne bar, Rusell and Peter have spent the evening watching a premier league game between two of their favorite teams. Peter offers to drive Rusell home in the new Company car just before...

Post a response to the following: Describe an example of when you encountered a statistic you did not understand. In your description, include what the statistic was, how or where you encountered it,...

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...

Consider, sampling from a multivariate normal distribution with mean vector = (1, 2, . . . , M) and covariance matrix 2I. The log-likelihood function is Show that the maximum likelihood estimates of...

Solve attached? I need this paper analyzed using qualitative analysis and answer the following questions: Our problem statement was: The problem to be addressed by this assessment is the impact of...

Solve (a) On one chart, plot the flow velocity in mph as a function of the slope, varying the slope from 0 to 90. (b) On the other chart, plot the flow velocity in mph as a function of the flow...

3 In 0900'0 500O'D 1000 0 FIG. 4. Process H alloy composition: (a) & chart, (b) "chart based on log ratios, (o) X' versus 73. S 0900'0 50COD 100070 S602 R. A. Boyles For this data set, step (3) in...

please answer it in python ERM classification attempt using incorrect knowledge of data distribution (Naive Bayesian Classifier, which assumes features are independent given each class label)... For...

This exercise has to do with the MarcenkoPastur law of Theorem 16.2. You are asked to run a simulation study to numerically verify the result of the theorem. Consider n = 10 and n = 100, and p = n/2...

The probability density function (pdf) for a 2-dimensional real-valued random vector X is as follows: p(x)=P(L=0)p(xL=0)+P(L=1)p(xL=1). Here L is the true class label that indicates which...

For the company: Eli Lilly and Company Please answer for the COMPANY Eli Lilly and Company Retrieve your company's most recent annual financial statements and answer the following: 1). Compute your...

Tim and Autumn Davis are trying to figure out their current financial health. They will pay off their car loan in three years, their gross household income is $5,700 per month, and they receive $95...

5.3 Determine the output voltage when 20 V is applied to the inverting terminal of an op amp and +30 V to its noninverting terminal. Assume that the op amp has an open-loop gain of 200,000.

How can user- defined operator overloading harm the readability of a program?

What assumption do we make when we duration hedge? Is this a reasonable assumption to make?

Use MATLAB and the Symbolic Math Toolbox to find the Laplace transform of the following time functions: a. f (t) = 8t 2 cos (3t + 45) b. f (t) = 3te -2t sin (4t + 60)

Refer to the information provided for Plasma Screens Corporation in E127. Required: 1. Calculate the following profitability ratios for 2021: a. Gross profit ratio. b. Return on assets. c. Profit...

At the bottom A of the vertical inside loop, the magnitude of the total acceleration of the airplane is 3g. If the airspeed is 800 km/h and is increasing at the rate of 20 km/h per second, calculate...

Can we put that she will be making weekly payroll deductions inthe amount of $52.75 to the housing equalling $211.00 monthly

Consider again the conditions of Exercise 15, and suppose that the sample size is n = 10. Determine the value of the constant c that defines the UMP test at the level of significance 0 = 0.05. Use...

Suppose that the joint p.d.f. of two random variables X and Y is as follows: Determine (a) The conditional p.d.f. of X for every given value of Y, (b) Pr(X f(x, y)|c(x + y2) for 0-x-land 0

Prove that for every two events A and B, the probability that exactly one of the two events will occur is given by the expression Pr(A) + Pr(B) 2 Pr(A B).

23. Why do recent studies show a stronger relationship between brain size and IQ than older studies didpg109

12. An enriched environment promotes growth of axons and dendrites. What is known to be one important reason for this effectpg109

5. If all cells in an amphibians tectum produced the same amount of TOPDV, what would be the effect on the attachment of axonspg109