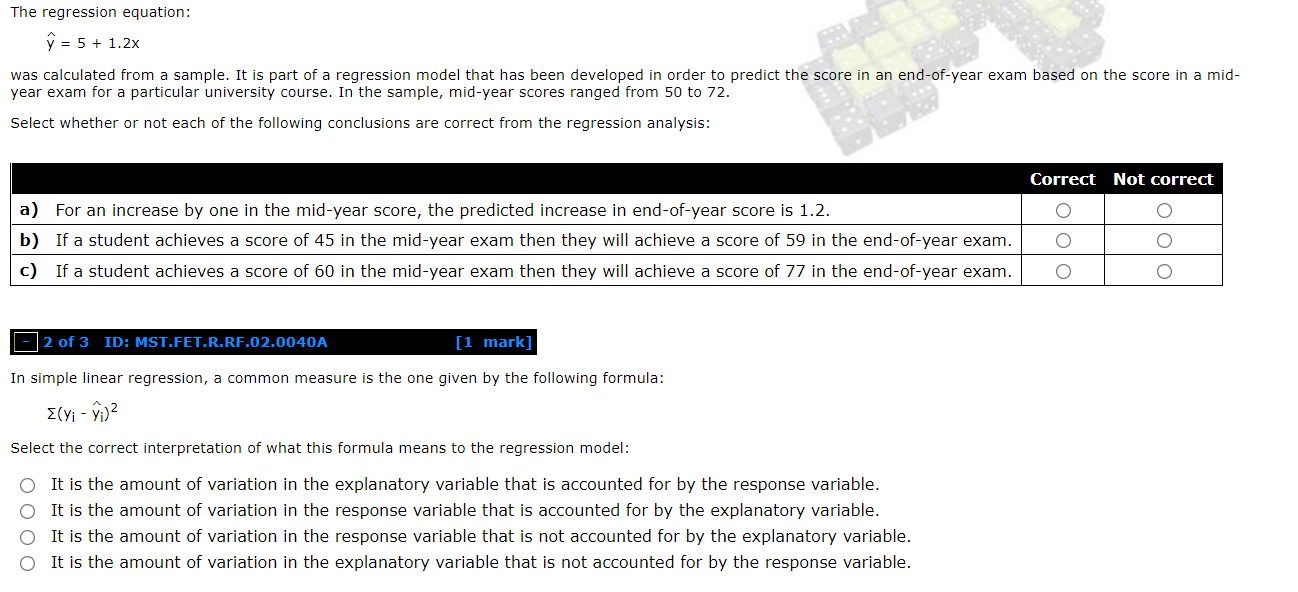
The regression equation: 5? = 5 + 1.2): was calculated from a sample. It is part of a regression model that has been developed in order to predict the score in an endofyear exam based on the score in a mid- year exam for a particular university course. In the sampler mid-year scores ranged from 50 to 72. Select whether or not each of the following conclusions are correct from the regression analysis: Correct Not correct a) For an increase by one in the midyear score, the predicted increase in endofyear score is 1.2. O O b) If a student achieves a score of 45 ln the midyear exam then they will achleve a score of 59 in the end-ofyear exam. 0 O c) If a student achieves a score of 60 ln the midyear exam then they will achleve a score of 77 in the end-ofyear exam. 0 O In simple linear regression, a common measure is the one given by the following formula: 2m , Vi)2 Select the correct interpretation of what this formula means to the regression model: It is the amount of variation in the explanatory variable that is accounted for by the response variable. [t is the amount of variation in the response variable that is accounted for by the explanatory variable. [t is the amount of variation in the response variable that is not accounted for by the explanatory variable. 0000 [t is the amount of variation in the explanatory variable that is not accounted for by the response variable. A simple linear regression equation is to be constructed to determine if there is a linear relationship between a response variable (Y) and an explanatory variable (X). A random sample of size n has been collected and the values xi and vi for i = 1, 2, ..., n have been recorded. The residuals [ei) in this analysis are defined as the difference between the observed values of Y and the values on predicted by the regression equation. The scatterplot or residual plots can be used to see if the regression equation is an appropriate model for the data. An assumption required for the simple linear regression equation to be valid is: the residuals are independent of one another the variance of X is equal to the variance of Y X and Y are independent the residuals are constant 0000