the solutions are provided. Please explain the sol. step by step.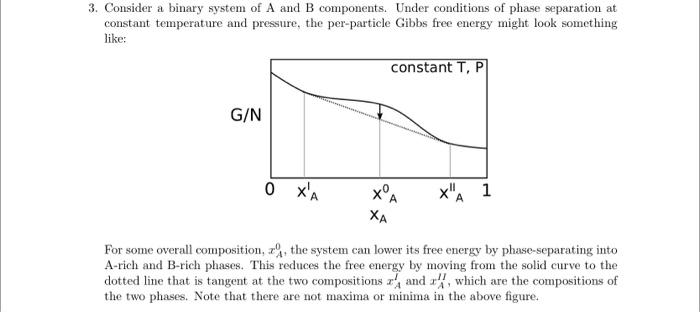

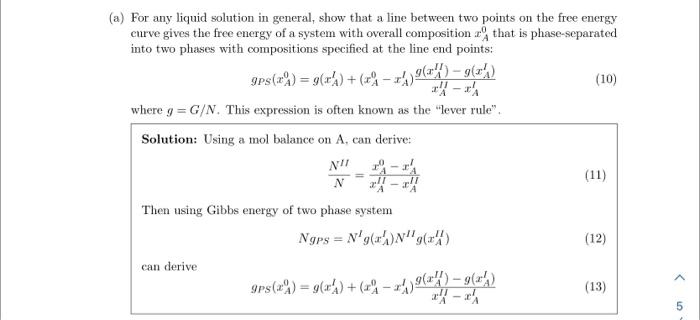
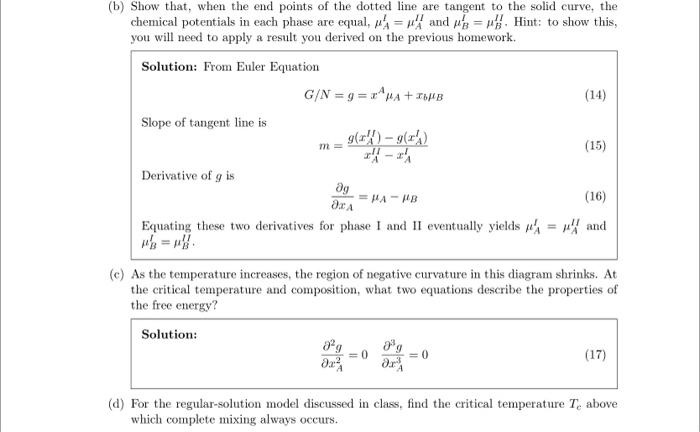
3. Consider a binary system of A and B components. Under conditions of phase separation at constant temperature and pressure, the per-particle Gibbs free energy might look something like: For some overall composition, xA0, the system can lower its free energy by phase-separating into A-rich and B-rich phases. This reduces the free energy by moving from the solid curve to the dotted line that is tangent at the two compositions xAI and xAH, which are the compositions of the two phases. Note that there are not maxima or minima in the above figure. a) For any liquid solution in general, show that a line between two points on the free energy curve gives the free energy of a system with overall composition xA0 that is phase-separated into two phases with compositions specified at the line end points: gPS(xA0)=g(xAl)+(xA0xAl)xAIIxAIg(xAII)g(xAI) where g=G/N. This expression is often known as the "lever rule". Solution: Using a mol balance on A, can derive: NNII=xAIIxAIIxA0xAI Then using Gibbs energy of two phase system NgPS=Nlg(xAI)NIIg(xAII) can derive gPS(xA0)=g(xAI)+(xA0xAI)xAIIxAIg(xAII)g(xAl) b) Show that, when the end points of the dotted line are tangent to the solid curve, the chemical potentials in each phase are equal, Al=AII and BI=BII. Hint: to show this, you will need to apply a result you derived on the previous homework. Solution: From Euler Equation G/N=g=xAA+xbB Slope of tangent line is m=xAIIxAlg(xAII)g(xAl) Derivative of g is xAg=AB Equating these two derivatives for phase I and I1 eventually yields AI=AII and Bl=BII c) As the temperature increases, the region of negative curvature in this diagram shrinks. At the critical temperature and composition, what two equations describe the properties of the free energy? Solution: xA22g=0xA33g=0 d) For the regular-solution model discussed in class, find the critical temperature Tc above which complete mixing always occurs. Solution: xA=0.5,XAB=2TC=2kBz(wAB2wAAwBB)