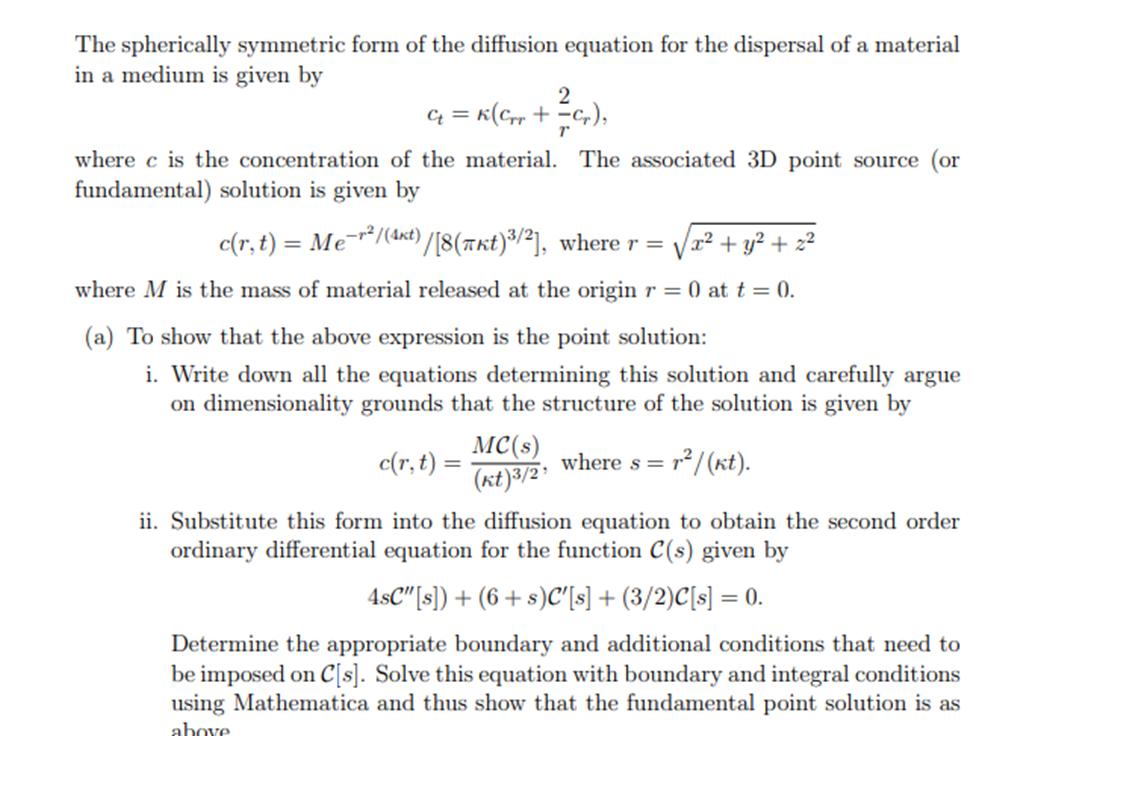
The spherically symmetric form of the diffusion equation for the dispersal of a material in a medium is given by 2 G = K (Crr + =C), where c is the concentration of the material. The associated 3D point source (or fundamental) solution is given by c(r, t) = Mer/(4kt) /[8(kt)/2], where r = x + y + 2 where M is the mass of material released at the origin r = 0 at t = 0. (a) To show that the above expression is the point solution: i. Write down all the equations determining this solution and carefully argue on dimensionality grounds that the structure of the solution is given by c(r, t) = where s= r/(kt). MC(s) (kt)3/2 ii. Substitute this form into the diffusion equation to obtain the second order ordinary differential equation for the function C(s) given by 4sC" [s]) + (6 + s)C'[s] + (3/2)C[s] = 0. Determine the appropriate boundary and additional conditions that need to be imposed on C[s]. Solve this equation with boundary and integral conditions using Mathematica and thus show that the fundamental point solution is as above (b) An explosion at a height H above the ground releases a mass M of toxic material into the atmosphere. Subsequently the material diffuses away from the point source. The atmosphere is assumed to be semi-infinite in extent and the ground impermeable to the material. Using the point source solution from part (a) determine an expression for con- centration levels as a function of time at the point at ground level immediately below the release point. By introducing an appropriate time scale to and concentration scale co express your result in the form c = coc' (tot') where d' and t' are dimensionless and all parameters are removed from the description; this allows for presenting 'all' results (for different parameter values) on the one graph. Sketch d'(t'). Determine the (scaled and then) unscaled maximum concentration level reached at ground level and the time taken to reach this value, and comment on the dependence of these results on H, K.