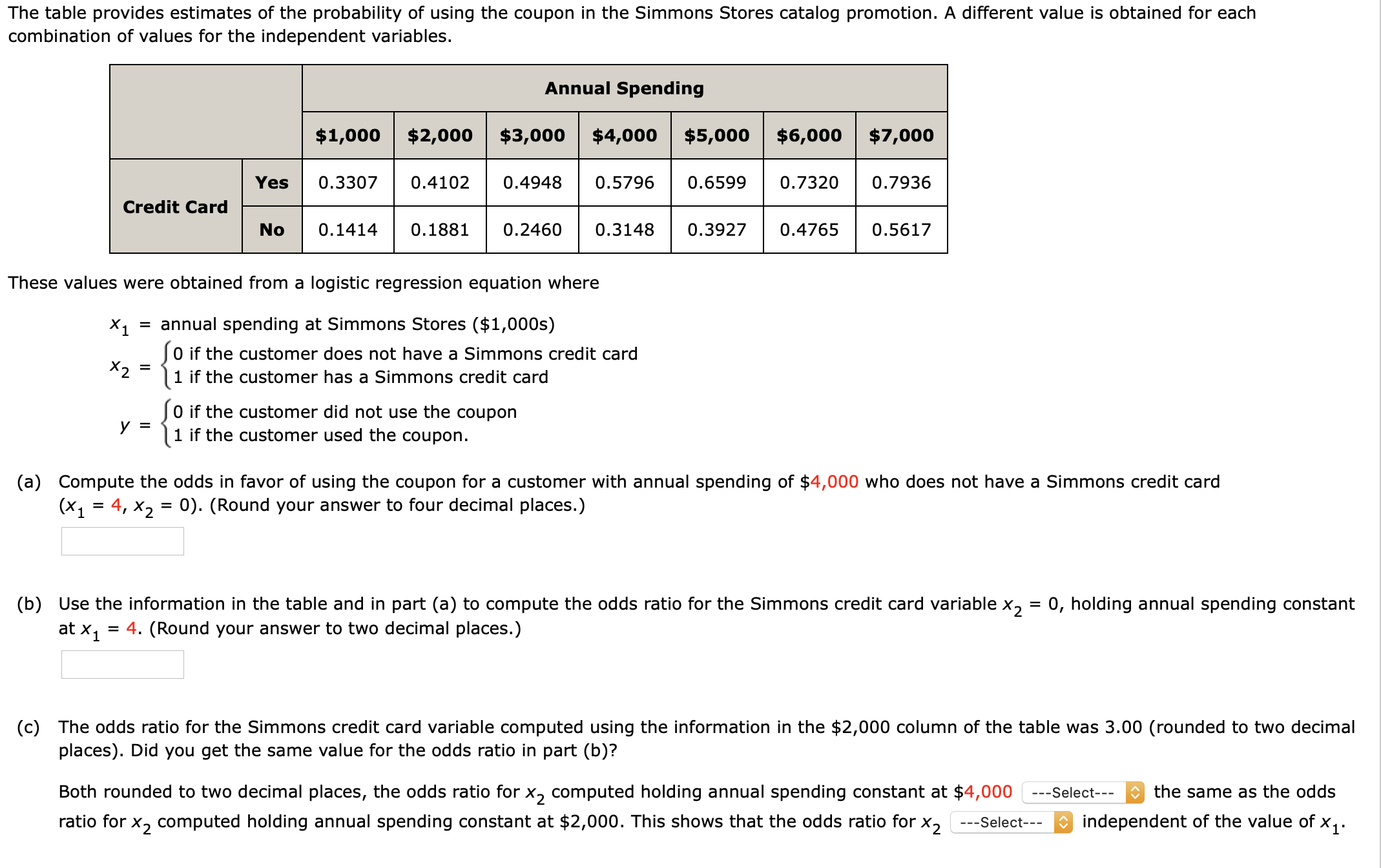
The table provides estimates of the probability of using the coupon in the Simmons Stores catalog promotion. A different value is obtained for each combination of values for the independent variables. Annual Spending $1,000 $2,000 $3,000 $4,000 $5,000 $6,000 $7,000 Yes 0.3307 0.4102 0.4948 0.5796 0.6599 0.7320 0.7936 Credit Card No 0.1414 0.1881 0.2460 0.3148 0.3927 0.4765 0.5617 These values were obtained from a logistic regression equation where X1 = annual spending at Simmons Stores ($1,000s) So if the customer does not have a Simmons credit card 1 if the customer has a Simmons credit card X2 = y = O if the customer did not use the coupon 1 if the customer used the coupon. (a) Compute the odds in favor of using the coupon for a customer with annual spending of $4,000 who does not have a Simmons credit card (x1 = 4, X2 = 0). (Round your answer to four decimal places.) (b) Use the information in the table and in part (a) to compute the odds ratio for the Simmons credit card variable x2 = 0, holding annual spending constant = 4. (Round your answer to two decimal places.) at X1 (C) The odds ratio for the Simmons credit card variable computed using the information in the $2,000 column of the table was 3.00 (rounded to two decimal places). Did you get the same value for the odds ratio in part (b)? Both rounded to two decimal places, the odds ratio for X2 computed holding annual spending constant at $4,000 ---Select--- the same as the odds ratio for X2 computed holding annual spending constant at $2,000. This shows that the odds ratio for x2 ---Select--- independent of the value of X1 The table provides estimates of the probability of using the coupon in the Simmons Stores catalog promotion. A different value is obtained for each combination of values for the independent variables. Annual Spending $1,000 $2,000 $3,000 $4,000 $5,000 $6,000 $7,000 Yes 0.3307 0.4102 0.4948 0.5796 0.6599 0.7320 0.7936 Credit Card No 0.1414 0.1881 0.2460 0.3148 0.3927 0.4765 0.5617 These values were obtained from a logistic regression equation where X1 = annual spending at Simmons Stores ($1,000s) So if the customer does not have a Simmons credit card 1 if the customer has a Simmons credit card X2 = y = O if the customer did not use the coupon 1 if the customer used the coupon. (a) Compute the odds in favor of using the coupon for a customer with annual spending of $4,000 who does not have a Simmons credit card (x1 = 4, X2 = 0). (Round your answer to four decimal places.) (b) Use the information in the table and in part (a) to compute the odds ratio for the Simmons credit card variable x2 = 0, holding annual spending constant = 4. (Round your answer to two decimal places.) at X1 (C) The odds ratio for the Simmons credit card variable computed using the information in the $2,000 column of the table was 3.00 (rounded to two decimal places). Did you get the same value for the odds ratio in part (b)? Both rounded to two decimal places, the odds ratio for X2 computed holding annual spending constant at $4,000 ---Select--- the same as the odds ratio for X2 computed holding annual spending constant at $2,000. This shows that the odds ratio for x2 ---Select--- independent of the value of X1